What is the equation for the tension on the ends of a cable suspended at different heights? [closed]

Question

I'm looking for an equation to find the tension on the ends of a cable suspended between two poles (one higher than the other) with no load but the cable itself.

I determined that the tension would be different on each end, that the shape of the suspended cable would be a catenary curve truncated at one end, and that the following would be the variables necessary in the equation:

• cable weight
• cable length
• vertical distance between ends
• horizontal distance between ends

Some links that could be helpful:

• http://www.scribd.com/doc/12736614/Sag-Tension-Calculation
• http://mysite.du.edu/~jcalvert/math/catenary.htm

Answer 1

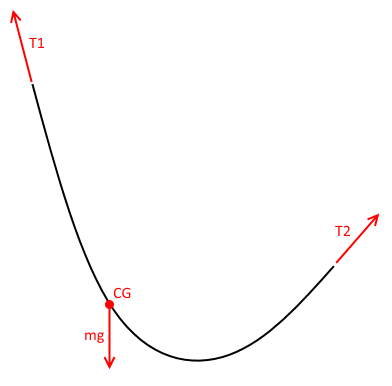
Since the cable is not moving horizontally you know the horizontal component of tension is the same at both ends. The total tension is the horizontal component divided by the cosine of the angle. So the ratio between the tensions is the ratio of the cosines. Since you know the shape of the curve you should be able to take it from here.

UPDATE

The general equation for a catenary (with lowest point at x=0) is

$$y = a \cosh \frac{x}{a}$$

Where

$$a = \frac{H}{w}\\ H = \text{horizontal tension}\\ w = \text{weight per unit length}$$

For a given horizontal distance and vertical displacement, we have to figure out the location of the lowest point and the tension - two equations, two unknowns.

From wikipedia.org/wiki/Catenary#Determining_parameters:
Given $s$, $v$, and $h$, then $a$ can be solved for numerically:

$$\sqrt{s^2 - v^2} = 2a \sinh \frac{h}{2a}, a > 0$$

where
$h$ is the horizontal distance between ends, $v$ is the vertical distance between ends, $s$ is the length of the cable, and $a$ is the y coordinate of the lowest point.

Next, we just need to find the position of the lowest point relative to the ends. To get the actual locations of $x_1$ and $x_2$ (the horizontal distances from the lowest point to the the left and right ends, respectively) you now have to solve

$$\begin{align}\\ v &= a (\cosh \frac{x_2}{a} - \cosh \frac{x_1}{a})\\ h &= x_2 + x_1 \tag1 \\ v &= a\left(\cosh \frac{x_2}{a} - \cosh \frac{x_2-h}{a}\right) \tag2 \end{align}$$

Solve (2) for $x_2$ then substitute into (1) to get $x_1$

Finally, the ratio of tensions comes from the ratio of cosines of the angle at the point of suspension:

$$\frac{T_2}{T_1} = \frac{\cos\theta_1}{\cos\theta_2}$$

We know the tangent at $x$ is given by

$$tan\,\theta = \frac{dy}{dx} = \sinh \frac{x}{a}$$

Combine with the trig identity

$$cos\,\theta = \frac{1}{\sqrt{1+\tan^2\theta}}$$

You finally obtain

$$\frac{T_1}{T_2} = \sqrt{\frac{1+\sinh^2\frac{x_2}{a}}{1+\sinh^2\frac{x_1}{a}}}$$

Answer 2

Actually, the curve is not skewed, but is in fact a symmetric curve that is truncated on one side. You can find the information you need here.

If the slope of the chain is dy/dx = tan θ, then T cos θ = H and T sin θ = wx. At B, T sin θ = ws.

T is the total tension, x is the distance from the lowest point to the desired point, w is the weight per unit length, s is the horizontal distance to B, B is the suspension point, and H is the horizontal tension.

Answer 3

Well, classical mechanics is your friend. Before going on to catenary equations and stuff, always draw some free-body diagrams :)

There's your catenary and the forces that act upon it, right? If you had $\vec {T_1}$, tension on left end would be $\sigma_1 = \frac{T_1}{A}$ ($A$ is cable cross-section). Similarly you'd find $\sigma_2$.

So it's as simple as finding $\vec {T_1}$ and $\vec {T_2}$. Use Newton's laws of motion in the static case:

$$T_{1y} + T_{2y} = mg$$ $$T_{1x} + T_{2x} = 0$$ $$y_2 T_{2x} + x_2 T_{2y} = x_{cg} mg$$

Now, I don't remember a lot about catenaries (I should, though), but I think that with the $(x, y)$ pairs of points of both ends and length of cable you should be able to find the cable equation. You can derive it to find the angles at each end so that:

$$T_{1y} = \left.\frac{dy}{dx}\right|_{x=x_1} T_{1x}$$ $$T_{2y} = \left.\frac{dy}{dx}\right|_{x=x_2} T_{2x}$$

If I'm right, you won't even need to use the third Newton equation (equilibrium of moments). But, if you do, you can find $x_{cg}$ with the catenary equation (is it on the lowest point? I don't know, but I read something along that line somewhere).

That should get you going. Good luck.