Moving coil contradiction of Faraday Induction

Question

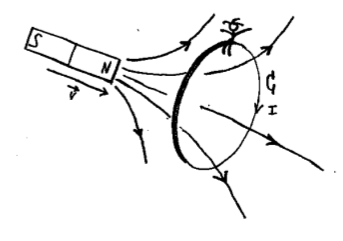
In Faraday's Induction Experiment, the e.m.f. induced in the induction coil becomes zero when the relative velocity of the coil and the magnet becomes zero. But one can also argue from a stationary observer's reference frame. Let's assume the magnet and the coil to be moving at an equal velocity.

For a stationary observer (who measures the induction apparatus in motion), there will be an induced e.m.f. in the region of space around the moving magnet, since there will be a relative velocity between any point in surrounding space and the magnet. Now since the coil moves through this region of space, it should therefore possesses an induced electric field as well.

I totally trust Relativity, and therefore this means that the stationary observer should compute a reverse e.m.f. that exactly cancels out the e.m.f. caused by the moving magnet, or maybe I am on the whole absurdly wrong (in which case I would like to know where my thought experiment went wrong).

Answer 1

For a stationary observer (who measures the induction apparatus in motion), there will be an induced e.m.f. in the region of space around the moving magnet, since there will be a relative velocity between any point in surrounding space and the magnet. Now since the coil moves through this region of space, it should therefore possesses an induced electric field as well.

There is electric field in the region of space around the moving magnet. Electromotive force is present not in space like the fields, but is present in the wire, acting on the charge carriers. The electromotive force acting on the charge carrier has two components; electric and magnetic force. In the frame of the observer,

$$ \mathbf F_{electromotive} = q\mathbf E + q\mathbf v\times \mathbf B. $$

In the frame of the moving system, $$ \mathbf F'_{electromotive} = q\mathbf E' + q\mathbf v'\times \mathbf B'. $$ Since the latter electromotive force and the product $\mathbf E'\cdot \mathbf v'$ vanish for all times in the frame of the moving system, the electromotive force has to vanish also in the frame of the observer. This is possible since the magnetic force $q\mathbf v\times \mathbf B$ may cancel the electric force $q\mathbf E$.

Answer 2

In Faraday's experiment, the relative velocity between the coil and the magnet define whether a change of induced magnetic field flux occurs around the coil or not. So if you consider them at rest, and when you consider them moving at the same velocity relative to one another, then there's no change of magnetic field flux felt by the coil.

Be careful there are only two interpretations of the Faraday's experiment, in the first one, one studies the change of flux in the coil, so the observer is in the coil's frame of reference, and in the second interpretation (which does not always hold) the observer is sitting on the magnet, and the current in the coil caused by the Lorentz force is studied.

Elaboration of the first interpretation: Observer in coil's frame of reference:

Flux of the induced magnetic field $\vec{B}$ : $$\Phi = \int \int_{S} \vec{B} d\vec{S} $$

Where "S" is the surface confined by the coil. Due to the relative motion of coil-magnet, the total flux increases and induces a difference of potential: $$ V = -\frac{d\Phi}{dt} $$

Finally as for your relativity argument, it hold perfectly here in the sense that the physics in either frames is the same, as the driving element in Faraday's law is defined by the relative motion of the coil-magnet pair, whereas for an outside observer, although a change of magnetic flux is created around the observer due to the motion of the magnet, but as the coil (in your example) is moving with the same velocity, the change of $\Phi$ is not felt by the coil.

Answer 3

Now since the coil moves through this region of space, it should therefore possesses an induced electric field as well.

I don't follow your reasoning here. The electric and magnetic fields are reference frame dependent; the fields 'mix' in a certain way via the Lorentz transformation.

In the reference frame in which the magnet is at rest, the magnetic field is static, there is no induced electric field and thus, no emf in this frame.

In a relatively moving reference frame, the magnetic field is not static, there is an induced non-conservative electric field and, thus, a non-zero emf along some closed paths in this frame.

This is a straightforward consequence of the fact that the scalar electric and vector magnetic potentials are components of a four-vector - a four-potential - in special relativity.