I'm currently revising for a vibrations and waves module that I am taking as part of my physics degree.
One of the our final questions involved finding equations for the displacements of the two masses in this system as a superposition of their normal modes:
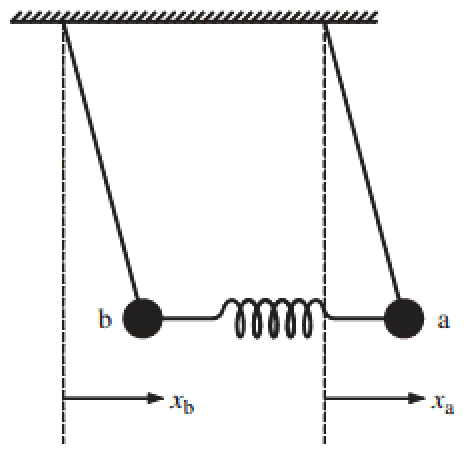
I found the equations of motion for each mass to be: $$ \ddot{x_a} + \gamma\dot{x_a} + x_a(\omega_0^2+\omega_s^2) = x_b\omega_s^2\\\ddot{x_b} + \gamma\dot{x_b} + x_b(\omega_0^2+\omega_s^2) = x_a\omega_s^2\\Here: \omega_0^2 = \frac{g}{l}~~\omega_s^2=\frac{k}{m}~~\gamma=\frac{b}{m}$$ Here I let $ q_1 = x_a+x_b~and~q_2 = x_a-x_b: $ $$\ddot{q_1} + \gamma\dot{q_1} + q_1\omega_0^2=0\\\ddot{q_2} + \gamma\dot{q_2} + q_2(\omega_0^2+2\omega_s^2)=0 $$ From here I can't see where to go. I did attempt substituting in a general solution such as $q_1 = C_1 \cos(\omega t)$ but I get a mixture of sines and cosines and I can't solve it for anything useful.
Any help would be great as this is the last topic that I need to learn! Thanks, Sean.
