Is this way of solving Thevenin theorem correct?

Question

This thevenin theorem is really giving me a headache, and I've really searched many sources in the internet but they confused me more and more. Take this as an example:

The guy in the above link says:

The 6Ω resistor is short circuited and the 5Ω one is open. Therefore, their currents are zero and RTh=2Ω .

But see this link for example and especially this picture:

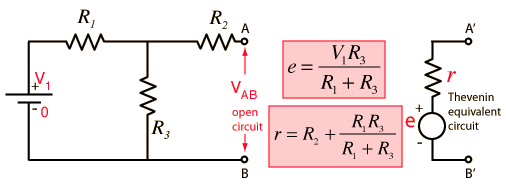
According to the above the $R_1$ and $R_3$ resistors should be shorted and the only one that should count is $R_2$.

And see this link also.

The three links solve the Thevenin theorem in different ways and especially in finding $R_{th}$ can somebody explain this to me in a simple way?

Answer 1

Both examples are using the same methods.

In the first example, the break in the circuit removes the 6 ohm and 5 ohm resistors altogether. All of the current then flows through the 2 ohm resistor, causing the Thevenin resistance to be 2 ohms.

In the second example, the current first passes through $R_2$, then is split between $R_1$ and $R_3$ in parallel. The parallel resistance between $R_1$ and $R_3$ is $\frac{R_1 R_3}{R_1 + R_3}$, and adding on the $R_2$ which is in series to both of those, we get the Thevenin resistance as $R_2 + \frac{R_1 R_3}{R_1 + R_3}$. This value is the same as the one provided by your source.

If you need any more clarification, feel free to ask in the comments.

Answer 2

See here. There's instructions on how to calcuate $V_{th}$ and $R_{th}$.

Let $I_3$ be the current through $R_3$, $I_1$ through $R_1$, etc.

With $V_{AB}$ open, by KVL, we have $V_1 - R_1I_1 - R_3 I_3 = 0$ and $V_1 - R_1 I_1 - R_2 I_2 -V_{AB} = 0$, but when $V_{AB}$ is open we have $I_2 = 0$ and so $I_1 = I_3$, so $V_1 = (R_1 + R_3) I_1\implies\frac{V_1}{R_1 + R_3} = I_1$ and $V_1 - V_{AB} = R_1 I_1 \implies I_1 = \frac{V_1 - V_{AB}}{R_1}$. Set the two equations equal and you get $V_{AB} = V_1( 1 - \frac{R_1}{R_1 + R_3}) = V_1(\frac{R_3}{R_1 + R_3})$.

Now according to the article I linked, you can go about calculating $R_{th}$ in two ways. I'll cover the second way, that of finding $R_{th}$ by short-circuiting $V_1$. When you do that you get $R_1 || R_3 = \frac{R_1R_3}{R_1 + R_3}$, which is then in series with $R_2$, so $R_{th} = R_2 + \frac{R_1R_3}{R_1 + R_3}$.

Hope that helps, please comment for any questions :)