Charge density in wires [closed]

Question

I have a very simple circuit, composed of a capacitor and a battery with constant $V$. The wires that connect these two components allow the capacitor to gain a voltage V ( the same V of the battery). Do the wires that connect the capacitor and battery maintain a neutral charge density? And why?

Answer 1

Yes they do. To see this, note that because your only resistance is the small wire resistance, the capacitor reaches it's steady state instantaneously (the time constant RC is small). In this steady state, the current going through the circuit is zero because $I=C \frac {dV_c}{dt}=0$. Now that we know the current is zero, consider Gauss's law: $$\boldsymbol \nabla.\mathbf E(\mathbf x) = \frac { \rho(\mathbf x)}{\epsilon_0}$$ From ohm's law we have $\mathbf J(\mathbf x) = \sigma \mathbf E(\mathbf x)$ or $\mathbf E(\mathbf x) = \sigma^{-1} \mathbf J(\mathbf x)$, where $\sigma$ is the conductivity of the wire. Now with zero current this gives $\mathbf E(\mathbf x)=0$ inside the wire. Plugging this into Gauss's law we have: $$\rho(\mathbf x)=\epsilon_0 \boldsymbol \nabla.\mathbf E(\mathbf x)=0$$ Therefore, the charge density inside the wire is identically zero, which means that it is neutral.

Edit (answer to comment) :

The exact same reasoning also applies to the inside of the conducting plates. Inside the thickness of each plate the electric field is zero, making the charge density inside the plates also zero. The charge you are referring to builds up on the outside surface of the plates, not on the inside. Similarly, the argument above only works for the inside of the wires.

The charge distribution on the outside surface of the conductors depends on the electric field outside the wire. Explicitly, using the boundary conditions on the normal electric field we have: $$\rho_s(\mathbf x) = \epsilon_0\hat {\mathbf n}.\mathbf E(\mathbf x)|_{wire \ boundary}$$ Where $\hat {\mathbf n}$ is the normal unit vector for the outer boundary of the wire, and $\rho_s$ is the surface charge density. Now suppose you have the circuit below, without any other outside charges/currents.                                                                    

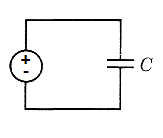
The magnitude of the electric field outside the plates (which is where the wires are), neglecting fringing effects, is: $$ E = \frac {\rho_{sp}}{2\epsilon_0}+\frac {-\rho_{sp}}{2\epsilon_0}=0$$ Where $\rho_{sp}$ is the surface charge density on the surface of the plates (one positive and one negative). So the electric field outside the wires is zero. Which means that by the equation for $\rho_s$, we have: $$\rho_s(\mathbf x) =\epsilon_0 \hat {\mathbf n}.\mathbf E(\mathbf x)|_{wire \ boundary}=0$$ Thus, there is also no surface charge density on the outside surface of the wires.

Answer 2

Just my guess only.

What you mean by charged density should be about -ve electron charged density as the +ve ion nucleus center of the conductor lattice are fixed. As there is no current flow, there should be equilibrium within the conductors where there is zero net electric field intensity - the emf of the battery being exactly balanced by the back emf of the capacitor.

So there is net zero charge density within the conductor wire.

[edit] I think the above is wrong. The wire and the plate connected to the +ve battery terminal has a +ve charge density. It is -ve charge density for the -ve terminal. The wires and the plates are just an extension of a battery terminals; they acquire the same potential as the terminals. The two wire/plate pairs have the same potential difference as that of the battery. I don't know if it is easy to calculate the charge distributions

Answer 3

The wire will have the neutral chrge densinty because the movement of electrons on to the plate and away from the other plate is compensate d by battery