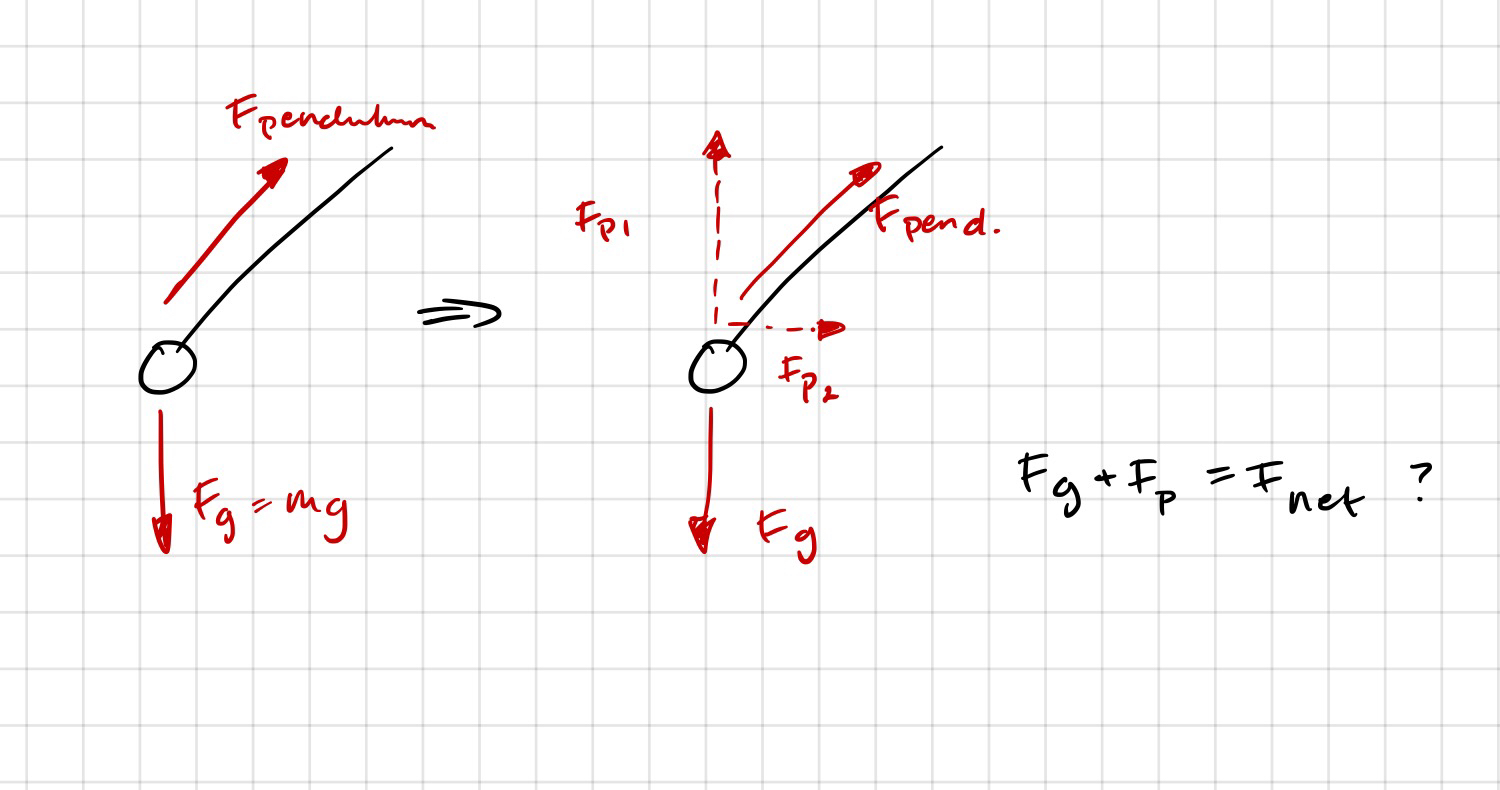
Regarding potential and kinetic energy, when a pendulum swings we do not count the force from the string because it doesn't affect the energy, but why? if we split the force from the string to it's components it does affect the force of gravity which in turn affects the acceleration of the pendulum which in turn affects the kinetic energy? Or something like skating down a ramp, the normal force can be split into it's components and therefore affecting the gravitational force.
When we calculate potential and kinetic energy of a pendulum, we can ignore the action of the string, because the string (if it does not stretch and contract) does not produce or consume any energy.
We can predict that because there is no source or storage of energy behind it.
We can also notice that its force is always normal to the trajectory of the mass and therefore the Force x Distance product is always zero as well.
The work-kinetic energy theorem states that $W_{net}=\Delta KE$. But we also know that $W=\vec{F}\cdot\vec{d}=Fdcos(\theta)$. In the case of the ramp, the normal force is perpendicular to the ramp, but the displacement is along the ramp. This means the displacement is perpendicular to the normal force and $W=\vec{F_N} \cdot \vec{d}=F_Ndcos(90^\circ)=0$ and there is no contribution to the change in the kinetic energy.
Same idea for the pendulum. The tension is always pointed toward the center of the object's circular path. The tension vector is always perpendicular to the circle the object is traveling on. Again, making $W=\vec{F_T}\cdot\vec{d}=F_Tdcos(90^\circ)=0$.
In these systems, if they are closed (and there is no friction or air resistance), $\Delta KE=-\Delta PE$ so the same idea applies to potential energy.
The diagram you drew is misleading. We are not taking apart the components of normal force. Instead, we are taking apart the components of gravitational force. Imagine a box sliding down a ramp. The ramp has an angle of $\theta$. Firstly, you must assign the x and y directions. Let x be parallel to the ramp and y be perpendicular to it. We must split the gravitational force $F_g$ on the box along x and y. The force $F_g$ is split into $F_{gx}$ and $F_{gy}$. We use trigonometry to determine the magnitude of the forces $$F_{gx}=F_g\sin{\theta}$$ $$F_{gy}=F_g\cos{\theta}$$The normal force is perpendicular to the slope and its magnitude is $$F_N=F_{gy}=F_g\cos{\theta}$$ The normal force vector is facing the opposite of the gravitational force vector.$$F_n=-F_{gy} \Rightarrow F_N+F_{gy} = 0$$ Therefore, the net force along y cancels out. Along x, the net force is $F_{gx} $. The same can be done on a pendulum. We split the gravitational force into its components. If we were to split the pendulum force into its components just like you did in your diagram, the pendulum force vector facing in the opposite direction of the gravitational force vector would be smaller than the gravitational force. $$F_{p1}<F_g$$ In fact, at almost all points on the pendulum, the total pendulum force is less than the gravitational force.
Notice that your $F_{p1}$ and $F_{p2}$ components point toward opposite sides of the string. That means one points in the direction of motion and the other points against the direction of motion, so one will contribute positive work and the other will contribute negative work.
We might leave it as a exercise for the student to show that the contributions cancel out, but this is an opportunity to talk about the importance of the mathematical properties of vector products.
In particular we know that the dot (inner; scalar) product of vectors distributes over additions $$ \vec{A} \cdot \left(\vec{B} + \vec{C}\right) = \vec{A} \cdot \vec{B} + \vec{A} \cdot \vec{C} \;,$$ so we conclude immediately that if $\vec{F}_\text{pend} = \vec{F}_{p1} + \vec{F}_{p2}$ and $F_\text{pend} \cdot \mathrm{d}s = 0$ then $$ \vec{F}_{p1}\cdot \mathrm{d}s + \vec{F}_{p2}\cdot \mathrm{d}s = 0\;,$$ and that your proposed treatment has the same result.
