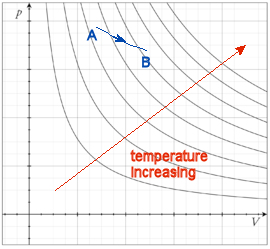
I have read in my notes that seeing the slope of a process in a PV diagram and comparing it with the isothermal slope, we can predict that is the temperature increasing or decreasing at that point. But i haven't mentioned the reason or the result for that. Since i had taken up thermodynamics a long time ago i don't remember any explanation for this. Can anyone please help me confirm if this is true or not?
Also isothermal slope at that point be taken as P/V (just to clarify).