Phasors are complex quantities used to partially represent real quantities that vary sinusoidally in time and perhaps in space. All phasors are time-independent. They represent partially the real quantity, and not completely, because they don't have information about the frequency.
To say that phasors are like vectors, is to say that complex numbers are like vectors, which is wrong. First of all, the similarities are for two-dimensional vectors only. Yes, addition and subtraction of two 2D vectors is analogous to addition and subtraction of two complex numbers; and multiplication of a 2D vector by a scalar is analogous to multiplication of a complex number by a real number. But, division of two vectors isn't even defined, however division of two complex numbers is defined; also, you can't just "multiply two 2D vectors, you must specify if it's a dot product or a cross product, however you can "just" multiply two complex numbers.
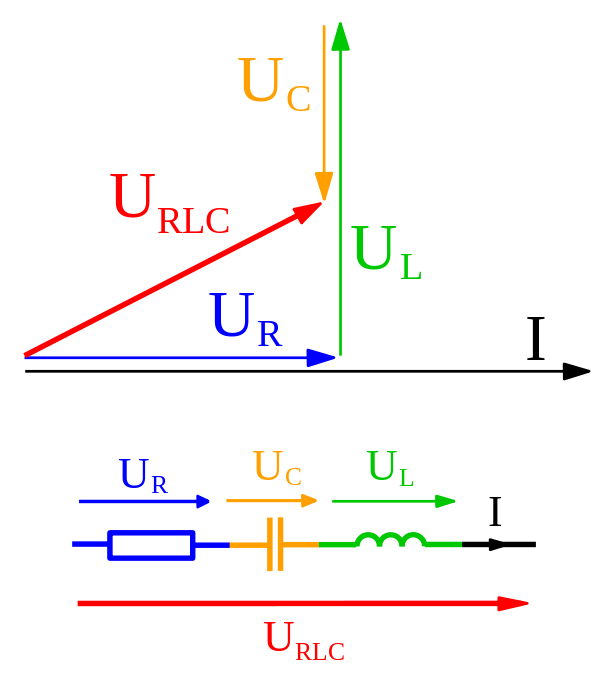
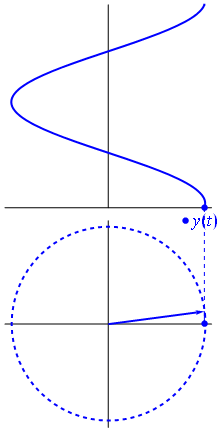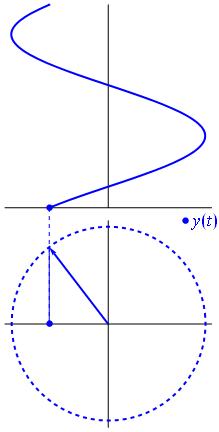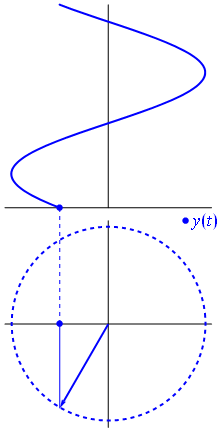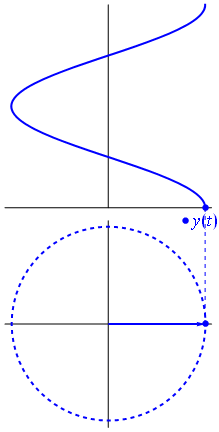
For electric circuits, a phasor voltage $ \tilde V $ is a complex constant, and it represents the amplitude and phase of the signal, but not its frequency. The signal $v(t)$ is a real-valued function of one real variable (one temporal, $t$), and it represents the real instantaneous value of the signal. The sinor $ v_c(t) $ is a complex-valued function of one real variable (one temporal, $t$), and it represents the complex instantaneous value of the signal. Some relations:
$v(t) = V_m \cos {(\omega t + \phi)} = \Re [\tilde V e^{j \omega t}] = \Re [v_c(t)] \tag*{}$
$\tilde V = V_m e^{j \phi} = V_m \cos {(\phi)} + j V_m \sin {(\phi)} \tag*{}$
$v_c(t) = \tilde V e^{j \omega t} = V_m e^{j \phi} e^{j \omega t} = V_m e^{j (\omega t + \phi)} = V_m \cos {(\omega t + \phi)} + j V_m \sin {(\omega t + \phi)} \tag*{}$
Note: $v(t)=\Re [\tilde V]$ only when $\omega t = \ldots, -4 \pi, -2 \pi, 0, 2 \pi, 4 \pi, \ldots$; in other words, only when $\omega t = 2 \pi k$, where $k$ is any integer.
For long transmission lines (electric circuits with distributed rather than concentrated parameters), a phasor voltage $ \tilde V(x) $ is a complex-valued function of one real variable (one spatial, $x$). The signal $v(x,t)$ is a real-valued function of two real variables (one spatial, $x$; and one temporal, $t$), and it represents the real instantaneous value. The sinor $v_c(x,t)$ is a complex-valued function of two real variables (one spatial, $x$; and one temporal, $t$), and it represents the complex instantaneous value. Some relations:
$v(x,t) = V_m e^{a x} \cos {(\omega t + \beta x + \phi)} = \Re [\tilde V(x) e^{j \omega t}] = \Re [v_c(x,t)] \tag*{}$
$\tilde V(x) = V_m e^{j \phi} e^{a x} e^{j \beta x} = V_m e^{a x} e^{j (\beta x + \phi)} = V_m e^{a x} \cos {(\beta x + \phi)} + j V_m e^{a x} \sin {(\beta x + \phi)} \tag*{}$
$v_c(x,t) = \tilde V e^{j \omega t} = V_m e^{j \phi} e^{a x} e^{j \beta x} e^{j \omega t} = V_m e^{a x} e^{j (\omega t + \beta x + \phi)} = V_m e^{a x} \cos {(\omega t + \beta x + \phi)} + j V_m e^{a x} \sin {(\omega t + \beta x + \phi)} \tag*{}$
For general electromagnetic theory, phasors are complex-valued functions of three real variables (three spatial, $x$, $y$, $z$). For instantaneous electric field vector, $\mathbf E (x,y,z,t)$, its phasor is $\mathbf {\tilde E} (x,y,z)$, and the relation $\mathbf E (x,y,z,t) = \Re [\mathbf {\tilde E} (x,y,z) e^{j \omega t}]$ is satisfied.