I was thinking that maybe photons loss energy naturally when they travel great distances.
Or maybe the mass of all matter is increasing over time and therefore photons emitted in the past are necessarily less energetic.
Or is the expansion of space backed up by so much other evidences that trying to find another explanation is completely foolish?
I posted more-or-less the same thing in response to another query about whether "tired light" still holds any water. I don't think there is any doubt that the redshifts we see in the spectra of distant objects are real doppler shifts that can be explained by an expanding universe. A crucial piece of evidence that seems to be ignored by almost everyone promoting "tired light" or similar, is that the expansion of the universe does not just result in the redshift of light but also results in the time dilation of other observed phenomena.
A key observation is that the light curves of Type Ia supernova, which should be almost exactly consistent as they arise from a very consistent astrophysical phenomenon - the detonation of a white dwarf close to the Chandrasekhar mass - become broader at higher redshifts. The amount of broadening is exactly in proportion to the amount of redshift $(1+z)$. i.e the cosmological time dilation works as expected for an expanding universe, see for example Blondin et al. (2008). A "gradual redshifting" of light as it travelled a distance cannot explain this time dilation.
The plot below shows how the measured "de-dilation factor" from type Ia light curves depends on their redshift (from Blondin et al. 2008). It goes as $(1+z)^{-1}$, exactly as expected (well to within 10%). The horizontal dashed line is what would be expected from "tired light" (no time dilation) and is rejected at 10 sigma.
This exact method was proposed by Wilson (1939) as a test of the expanding universe theory versus the "gradual dissipation of photonic energy" (aka "tired light"). The test has been passed with flying colours.
Any alternative hypothesis for why the redshift of galaxies is proportional to their distance must also explain why the duration of type Ia supernovae is stretched by the same factor $(1+z)$. Or, if it is not to be some arbitrary change in the properties of type Ia supernovae with time, then an alternative theory must explain why photons emitted at the start of a supernova explosion appear to take take less time to get to us than those emitted towards the end of the supernova.
Your last paragraph hits the bullseye.
General Relativity seems to be a good theory - at least it appears to be supported by the experimental evidence available. If we make a couple of apparently plausible assumptions about the universe (homogeneity and isotropy) then General Relativity predicts the universe is (approximately) described by the FLRW metric, and the FLRW metric predicts the cosmological red shift.
If you decide that the cosmological red shift is caused by tired light, or any of the other suggestions that have emerged over the years, then there's a problem because the universe can't be described by the FLRW metric. That means either our assumptions of the universe are wrong or GR is wrong, and neither possibility seems even remotely plausible.
There have been suggested alternatives, e.g., photons' losing momentum due to interactions with plasma. I just reviewed a paper which actually found that this mechanism accounted for the variations, as one varies one's line-of-sight, in the empirically observed value of Hubble's constant. I.e., the statistical fluctuations in the measurement of Hubble's constant vary by 20,000 parts per million, depending on which line-of-sight you adopt.
Here is a quote, "The all-sky maps of the observed variation of Hubble's constant can be reproduced from a theoretical point of view by introducing an intergalactic plasma with a varying density of electrons."
The paper, which I thought was reasonable and deserved publication, quotes other respectable papers in the literature, including a review paper by L. Marmet, "Survey of Redshift Relationships for the Proposed Mechanisms at the 2nd Crisis in Cosmology Conference", in: F. Potter (Ed.), Astronomical Society of the Pacific Conference Series, Vol. 413, 2009, 315-335.
For more on the intergalactic plasma of electrons, see D. L. Mamas, "An explanation for the cosmological redshift", Physics Essays 23 (2010), 326.
None of the suggested alternatives have yet received a consensus of acceptance by those who favour the Doppler effect explanation, which is the consensus explanation accepted at present.
Objects with really large gravity also causes red shifting.
It may be instructive to see how one measures red shift:
This describes the doppler shift, receding red, nearing blue.
There exists what they call the cosmic distance ladder a sequential combination of methods in estimating cosmic distances.
The doppler shift would not be enough to tell us about expansion or contraction or steady state, if there were not the information from the atomic spectra. It is the displacement of the spectra that measure the infrared change and thus the velocity of the object emitting the light.
From bottom to top, star, near galaxy, medium distance galaxy, far distance, and the lines are identified with specific atoms ( read the link). The near galaxy is moving away from us ( from the shift in the lines) at 1% the speed of light.
If it were scatters, the lines would be blurred, not recognizable, not clear as we measure them. The most economical interpretation of the distances ( measured with the cosmic distance ladder) and the redshifts is that everything is receding from us. Physicists choose the most frugal models.
The answer is a definite YES.
A larger atom in the past explain all the observed features, namely the redshift and the time dilation.
from Ned Wright's FAQ in Cosmology:
Q: Is the Universe expanding or is it just that our definitions of length and time are changing?
A: The definitions of length and time are not changing in the standard model. The second is still 9192631770 cycles of a Cesium atomic clock and the meter is still the distance light travels in 9192631770/299792458 cycles of a Cesium atomic clock.
In physics the units of measure are derived from the atom's properties. This fact makes us insensitive to any eventual atomic change and, it was not found one single law sensible to the absolute and actual size of the atoms.
The study of the evolution of the universe should be done from above, not depending on atomic properties, and it was already done here (arxiv) with a more formal proof here (vixra pdf).
It is true that in the SM "The definitions of length and time are not changing" but I will show that they should vary. In the past, several authors tried variations on units or on constants like $G,c$ and failed because they did not understood that all units M,L,T,Q (mass,length,time and charge) must vary in sync to stay aligned with the way we define them. In other words the units are derived from the atom's size, whatever it is.
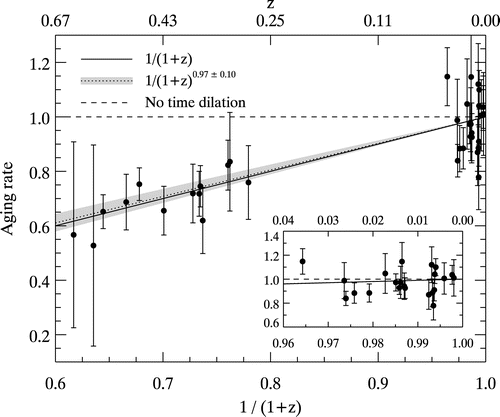
Below I will show in equations that a Larger atom in the past reproduces exactly the features we see in the skies (redshift of the ancient light, time dilation of ancient events - slower clock).
For example the definition of the electron mass $m_e$ is a tautology: in short - the electron mass is an electron mass. Lengthy - the electron mass is a tiny part of the unit kg which is equal to the mass of X atoms (prototype in Paris) each atom in turn having the mass of protons, neutrons, and binding energy expressible in $m_e$.
What do NOT vary: dimensionless constants like $\alpha$ (the fine structure) and space dependent properties $c, \varepsilon_0,G$.
M,L,T,Q units vary (dilation, a self-similar scaling) in sync with the atom under the law $\alpha(t)$ (see bellow)
velocity and force are insensitive to the scaling.
The most fundamental laws are insensitive the those variations, i.e. the force is always the same. Using dimensional analysis check that the sum of the exponents is zero:
Newton'2nd law : $[M^1][L^1][T^{-2}]$
Electromagnetic interaction ${M^{-1}}{Q^{2}}{L^{-3}}{T^{2}}$
Gravitational interaction $M^{-1}L^{3}T^{-2}$
Why the time unit change? because it is proportional to two specific energy levels, of a specific atom, which in turn is dependent on the distance between two specific orbitals which finally is dependent on $m_e$ which is $\propto \alpha(t)$.
Horror: the $h$ constant vary as $\alpha^2$ (the sum of exponents is 2 from dimensional analysis $M^1L^2T^{−1}$)
(The Horror is because everyone assume as sacred that the $h$ is an universal constant whithout further thinking that it depends on the units - see comment A below on Planck's law)
Bohr radius: $\propto \alpha$
Energy : $[M^1L^2 T^{−2}]$ i.e $\alpha$
Schrodinger equation is $\propto \alpha$ (WP - "In plain language, it means "total energy equals kinetic energy plus potential energy" )
Atomic Spectra: the Sommerfeld relation, $E_{j,n}=−m_e∗f(j,n,\alpha,c)$ thus $\propto \alpha(t)$ shows, without any doubt, that the redshift can be explained by a 'larger' atom.
Note :
The law of evolution of the units was derived to be
\begin{equation}
\alpha(t_{S})=e^{-H_{0}\cdot t_{S}}\
\end{equation}
where $t_S$ is the time unit in the reference S (from Space) where the units are constant thru time, by definition.
Some consequences of this viewpoint are:
It is applied to all scales in contrast with the SM that no one know how to apply locally. It allows that the orbits enlarge as time goes by and the Young Earth Paradox falls down (vixra pdf).
One parameter $H_0$ is enough to explain the evolution of the universe in large scale instead of the SM stack (6 parameters).
There is no need to invoke GR (the space is flat, isn't it?) , no BB, no cosmological inflation, no cosmological constant, no space expansion, no superluminal motion, no Dark Energy, and it will be shown in the future no Dark matter.
Physicist community adopted, as consensus, the SM expansion without knowing that another and quite simple model is possible. To read or not to read that vixra document? That is the question. It is up to the readers.
In the past the physics and the philosophers would say :
A more recent doc named "On the Evolution of the Universe" is in preparation.
a few sentences from it:
2.2 Does space expand or does the unit length decrease?
The difficulties of all cosmological theories indicate that particular attention must be paid to the fundamentals and assumptions behind all theoretical work presented thus far. In accomplishing this goal,several questionable aspects emerge, but one is particularly relevant: the statement “space expands” is an
absolute statement of a result that is not absolute. The correct form of the statement is “space expands in standard units”. Because matter has invariant properties in standard units, this relative occurrence can result from three different phenomena: space expansion, evanescence of matter, or variations in both space and matter at different rates.
A priori, the statement “space expands” is as valid as the statement “the universe rotates around us in 24 h” – both are statements of what is observed disregarding that these observations are not absolute but relative to us. Of course, there are sound reasons that support both statements.
The reason that supports the anthropocentric form “space expands” is the following: only in units that verify the concept of a reference body are physical laws known to be valid. All standard units support this concept, even when defined from constants that are assumed to represent properties of space, such as Planck units. Therefore, this type of unit, as far as is known, is privileged for the description of the universe. It is also known, however, that the invariance of the standard length unit is only apparent, as stressed by Einstein when he called the reference body a “reference-mollusc” [10]. Furthermore, in contrast to the case of Earth’s rotation, where the possibility of Earth rotating at such a fast speed without being noticed seemed impossible, here, the rate of decrease in the standard length unit would be so small that it could hardly be detected, even if it had detectable consequences.
3 Dilation Model
The following analysis assumes the full validity of the laws of physics for the scenario in which they were established, i.e., invariant space and matter, as well as their validity in this evolving universe within the accuracy of measurements of current data; in this way, there is no possible conflict with tested physics. It is also assumed that all constants hold invariant in standard units — note that we do not know, for example, whether the Boltzmann constant had in the distant past the same value it has today; this invariance is assumed because it is the simpler scenario and because it is in accordance with the characteristics of a self-similar phenomenon. There are no ad hoc hypotheses; therefore, the model cannot be adjusted by parametric fitting, and its validity does not depend on ad hoc hypotheses. The model is obtained by deduction from two fundamental results of cosmic observations (in standard units):
space expansion is a dilation, and field constants are invariant. No considerations of cosmological nature are made (e.g., concerning a cosmological gravitational field, or space curvature), in accordance with 2.1. Naturally, there is no distinction between “cosmic scale” and “local scale” for the Dilation
model.
comment A: on Planck's law
comment B: On the evanescence of photons
The evanescence of a photon in S (with $\alpha^2$ ) and in A (with $\alpha$, which is implied by field evanescence, has a known important consequence: a photon locally produced is identical to a photon that arrives from a distant source.
... the evanescence of photons is puzzling for the A(tomic) observer, who is unaware of the evanescence of fields. The space expansion produces a decrease in energy density with $\alpha^3$ but not with $\alpha^4$. The A observer can find a logical association: as the frequency shifts with space expansion, this evanescence of the photon energy maintains an invariant Planck constant in A along the propagation of the radiation. However, invariance of the Planck constant cannot be assumed as the cause for the variation in energy of a photon while it propagates. Above all, in the framework of the standard model, energy conservation is supposed to exist: the evanescence of photons is a violation of the fundamental principles of the physics of the standard model. Thus, it is necessary to explain what happens to the missing energy of electromagnetic waves. This problem arises for all space expansion theories. The answer is that fields evanesce in relation to space, which solves two problems of the standard model: why expansion is not detected locally by current measurements and why the energy density of radiation decreases with the 4th power of the expansion rate (negative).
In the Dilation model, there is no conservation of energy as currently defined; thus, the evanescence of the field and the correspondent decrease in the energy density do not violate any fundamental principle.
Still, the phenomenon is also a mystery. To explain this result, it can be considered that the evanescence of matter and of its static field may feed the expansion of the field. In this case, one can expect that the total amount of some still unknown entity, related to both matter and field, must hold constant during the process of field expansion; understanding this process is a new line of research.
The question is worded "is it possible?" -- Well ... If space is expanding from some unknown force related to "dark energy" following a "Big Bang" then there definitely would be a red-shift. But the only reason that we consider the red-shift a "proof" or "evidence" that space is expanding is that other than gravitational red shift, cosmologist can see no other reason for the red-shift. So the whole body of cosmology is hanging on a thin thread -- an assumption that there is no other explanation for red-shift consistent with the other unmentioned assumption -- that spacetime is flat (Euclidian) and that if we could travel to two other distant points in space and measure the angles of a large triangle that the angles would add to 180 degrees as they would on any flat surface. Note that on a spherical surface if you take 3 points that are sufficiently distant from each other the sum of the angles of a spherical triangle measured on the surface will exceed 180 degrees. For example if we choose the points where the zero meridian crosses the equator and another point where the 90 degree meridian crosses the equator the triangle will have 3 90 degree angles including the one at either the North or south pole. But we cannot get to two points in spacetime far enough from Earth to detect any "curvature" of spacetime by measuring the angles as seen from each of the 3 points. So our belief that spacetime is flat is based on our assumption (which seems reasonable) that the density of matter in space is close to uniform on a large scale. On a curved surface like a globe, the area of a circle does not increase as the square of the radius as it does on a flat surface. But based on our measurement of distance (based on brightness of cephiad variable stars and the brightness a certain kind of nova it appears that the amount of matter within any spherical volume increases as the cube of the radius and this is "evidence" that space time is flat in the region of space that we can see. However,the red shift of light decreases its brightness and the margin of error in determining brightness and (in the case of Cephied variables)the margin of error in determining their period, sort of waters down this evidence of flatness.
The ramification of curvature of space time under the equations of general relativity which have been calculated ONLY for flat space is not clearly understood. If there is a subtle curvature of space then the red shift might be a measure of the curvature of spacetime rather than a measure of expansion of a flat spacetime. In a flat universe, general relativity offers no escape from expansion, and the belief that the red shift is a result of the Doppler effect applied to light. However, if spacetime is curved then it might be that each point in space has a unique XYZT frame of reference such that we can have time dilation and red-shift based on separation alone WITHOUT a relative velocity.
So my answer to the question posed is that IF the universe is NOT flat (as it seems to be from the distribution of matter at various distances), then our assumption that the Doppler effect on light of galaxies rushing away from us as being evidence of the expansion of spacetime CAN BE challenged! I don't have the mathematical ability to apply the equations of the General Theory to curved spacetime. It might be that space curvature would cause a "blue shift" rather than a red shift for all I know. But I understand GT enough to believe that if space time is curved, then each point in space will have its own unique XYZT coordinates. And the curvature of space does not need to be positively curved as in the case of a globe -- it could be negatively curved. If it is positively curved then (as on the globe) if we went far enough in one direction we would come back to the same spot from the opposite direction and we would have a "closed" space time rather than a universe of infinite volume as is currently proposed. My "intuition" tells me that a positively curved space time would be indicated by a time dilation and red shift (reciprocally) of each observer at a great distance from the other.
But there IS "evidence" of expansion other than the red-shift. The cosmic microwave background SEEMS to be very consistent with The Big Bang Theory and the Big Bang Theory and the expansion of spacetime go "hand in hand".
But Albert Einstein was "suspicious" of the Big Bang Theory ... and the idea of the "beginning of time". The Big Band is NOT "something from nothing" but rather "everything from nothing"! It does not set well with me! Hocus Pokus Micerosis!!! :-). The application of the equations of the General Theory of relativity are tough enough in flat (Euclidean) spacetime, their application to curves space would be even more challenging.
