Otto and Carnot efficiency comparison

Question

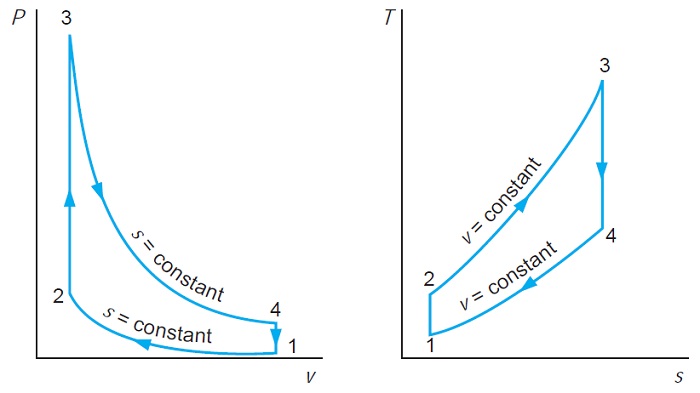
Lets say both engines operate between same temperature limits.

Carnot eff: $$ e_\text{Carnot} = 1-\frac{T_L}{T_H} $$ Otto eff: $$ e_\text{Otto} = 1-\frac{T_4-T_1}{T_3-T_2} $$ assuming that for Otto cycle points are located as below on a P-V diagram: $$ \array{ 3 & 4 \\ 2 & 1 } $$ I assumed the following for the Temperature limits. \begin{align} T_3&=T_H \\ T_1&=T_L \end{align} Question is: is there any way that the Otto cycle can be more efficient than the Carnot? (If e.x. $T_4$ will be close to $T_1$ enough, so the numerator of the Otto efficiency will become zero. Then the Otto cycle is more efficient, but as far as I know the most efficient is Carnot)

Please also suggest how to use the $T_4$ and $T_2$. (or get rid of them to calculate eff)

Answer 1

Otto cycle consists of two isochoric and two isentropic processes.

If $T_4$ approaches to $T_1$, then $T_3$ will approach to $T_2$, because for Otto cycle, line $3\to 4$ in $T-s$ diagram must always be vertically.

Thus, although the numerator of the Otto efficiency approaches to zero, but the fraction doesn't approach to zero, because the denominator approaches to zero too and we will have an indeterminate form.

Answer 2

well , the thermal efficiency of otto cycle can be reduced to

e (otto) = 1- T1 / T2 when T1= TL

e (carnot)= 1- T1 / T3

T3 is greater than T2 , then carnot is still higher efficiency than a

reversible otto cycle