To clarify my question further I'll use a practical example.
Here is the simplest paper plane design I could think of - it is a rectangular thick drawing paper with a weight attached at the middle of one of the long sides. I used a paper clip holding a metal key.
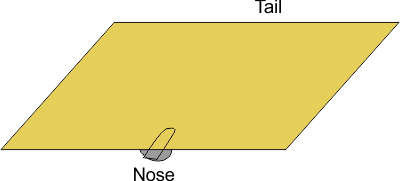
Then I perform this simple experiment: I hold the sheet of paper at the "tail" with approximately 45° nose down attitude and simply let it go - that is, there is no initial velocity. If the weight has the proper mass, the sheet of paper glides gracefully forwards (in fact I was quite surprised that such a crude design behaves so well).
What bothers me is that I can not explain to myself why the plane has such behavior. Here's the way I try to analyze it (which should be wrong since it does not stand to the facts). The plane is drawn as seen from the side:
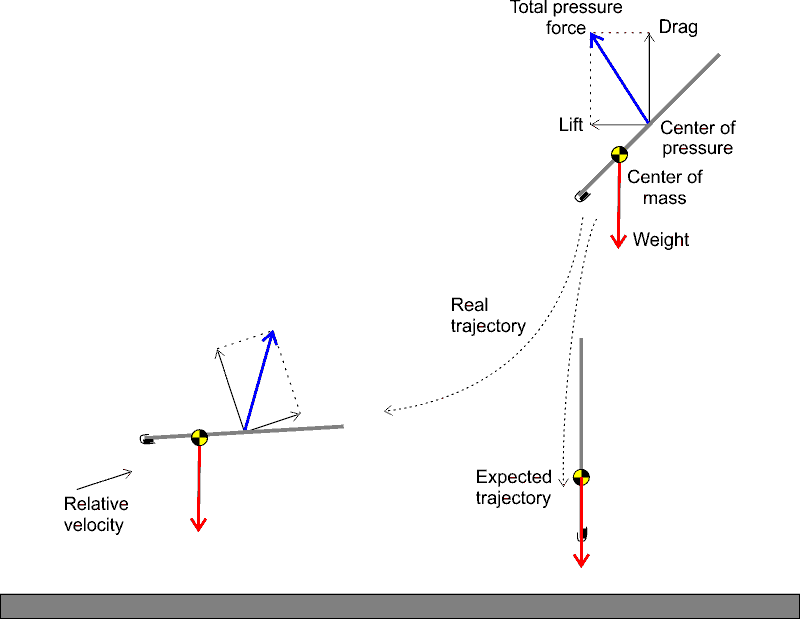
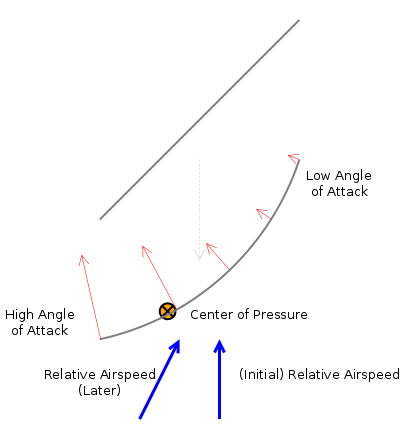
Since the wing is of very simple rectangular shape the center of pressure should be in its center. On the other hand by placing the weight at the nose we have moved the center of mass a little forward (which is said to be necessary for longitudinal stability of an airplane).
At moment t=0s when I let go of the plane there are no other forces acting on it except gravity. The plane gains a little velocity downwards and immediately the pressure forces begin to act. I've drawn the total pressure force and for clarity decomposed it into lift and drag components (the magnitudes and directions are only illustrative). The lift component explains why the plane starts to gain forward velocity.
If the forces act in this way then the pressure force should create a torque around the center of mass and rotate the plane counterclockwise (from the perspective of the drawing). But in reality the exact opposite happens - the plane rotates clockwise and continues to gain forward speed. We know that in airplanes the tail is used to produce torque which cancels the torque of the main wing but there is nothing here to cancel it. In fact the torque should act until the angle of attack is nullified and only the drag component remains. That is how a dart or an arrow will fall if dropped in the same way! The difference between dart and the plane above is that the center of mass of the former is much further away from the center of pressure. But still I don't understand where is that clockwise torque coming from and how it gets cancelled later when the plane gains enough forward velocity and achieves pitch stability.
EDIT/ADDON: Thanks everyone for the answers.
Since the bending of the paper really adds much complexity to the analysis I made the plane from firm cardboard as you can see from the following footage:

The wing is also square now. Since it is considerably heavier than before it does not glide so far but still the effect is the same.
Now with the wing curvature out of the picture, the explanation should lie in what Peter suggests: that the center of lift is not at the center of the wing but 1/4 closer to the nose. Something like this:
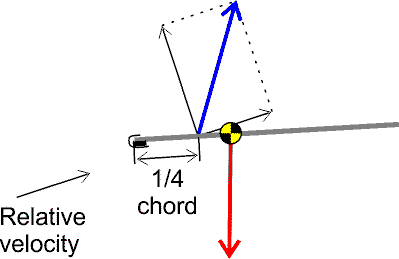
Why the lift center is not at the center but at 1/4th chord is quite unclear to me but I'll try and read about it.
Then the last thing that I would like to know would be how the wing flies in more or less stable angle of attack (or at least in some oscillating mode). The explanation would be easy if this 1/4th rule is not exactly solid and the point of pressure can move forwards and backwards in function of the angle of attack and/or velocity. Then if it moves very close to the c.m the moment will tend to zero. If it moves behind the c.m the torque will be ccw, the nose will drop and then the pitch oscillations could be explained. But is this really the case? Or the physics are much more complex for such simplification?