I tried to calculate one of the problems in the textbook Gauge Theory of Elementary Particle Physics by Ta-Pei Cheng and Ling-Fong Li.
On page 248 you can find the following calculation of a loop integral: 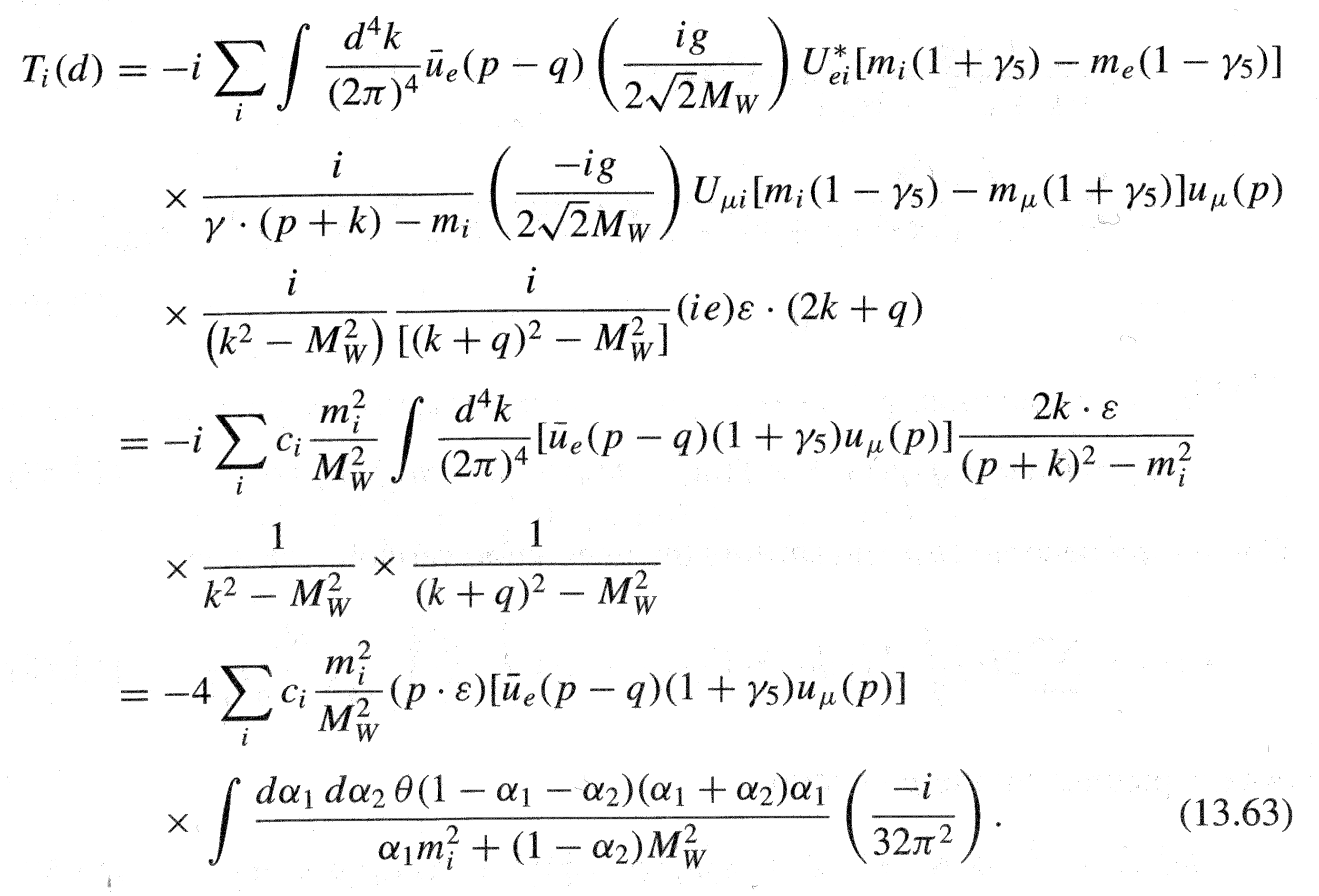
After the scond $=$ is there a $\gamma\cdot k$ missing? $$...\bar u_e(p-q)(1+\gamma^5)\gamma\cdot k\;u(p)...$$ instead of $$...\bar u_e(p-q)(1+\gamma^5)u(p)...$$ Because the mass dimension do not match. Am I right or did I make a bad mistake? I think they neglect terms with $m_e$ here, which would be OK.
With $c_i=\frac{g^2e}{4}U^*_{ei}U_{\mu i}$ and $\gamma\cdot p\;u_\mu(p)=m_\mu u_\mu(p)$.
