First, let's get user Jim's comment down to preserve it, as it is the first essential idea:
Note, you can treat the source like a focal point. Then any lens that is big enough to handle the laser can be used. You just have to put it one focal length away from the source
Now, to add to ARMS's great answer, you have yourself a nontrivial design problem.
If you go form ARM's suggestion, then the two lenses "stepping up" the beamwidth are what is known as a Galilean Telescope: the first lens is a diverging lens that forms a virtual focus of the collimated beam and then the second is a converging one that collimates the first len's virtual focus, i.e. the two lens's focal points are on top of one another. I have another suggestion below.
You probably need to find someone in your school (if you're a university researcher) with optical design skills and access to a program like Zemax or Code V. If you're in industry, you'll need to buy in this expertise. Since you're designing a system to image only an on-axis point, you could probably write a simple raytracer in Mathematica and use it to optimise the following general idea.
Your system, somewhat ironically given its huge size, is pretty much the same design problem as that of a high-power, infinity conjugate microscope objective. Born and Wolf, "Principles of Optics" has a good description of the general idea, using the aplanatic points of a spherical lens. Section 6.4, especially under the discussion of the high power objective, is a must-read for you. The basic idea is that one uses a sequence of weakly converging lenses to collimate the beam in many steps. You'll use a small diameter optic near to your beam to converge the beam a little bit: this forms a virtual image of the focus of the laser's output at a point that is behind the real focus. Then a slightly bigger optic converges the beam a little more, making a virtual image even further behind the laser. Eventually you are collimating light from a virtual image that can be metres away: the trick is to only do a little bit of convergence at once: weak lenses make for very low aberration: if you split a total optical power between $n$ lenses, each one's aberration varies roughly like between $\frac{1}{n^2}$ and $\frac{1}{n^4}$, so you can get drastic lowering of aberration by this splitting. I have sketched the rough idea below:
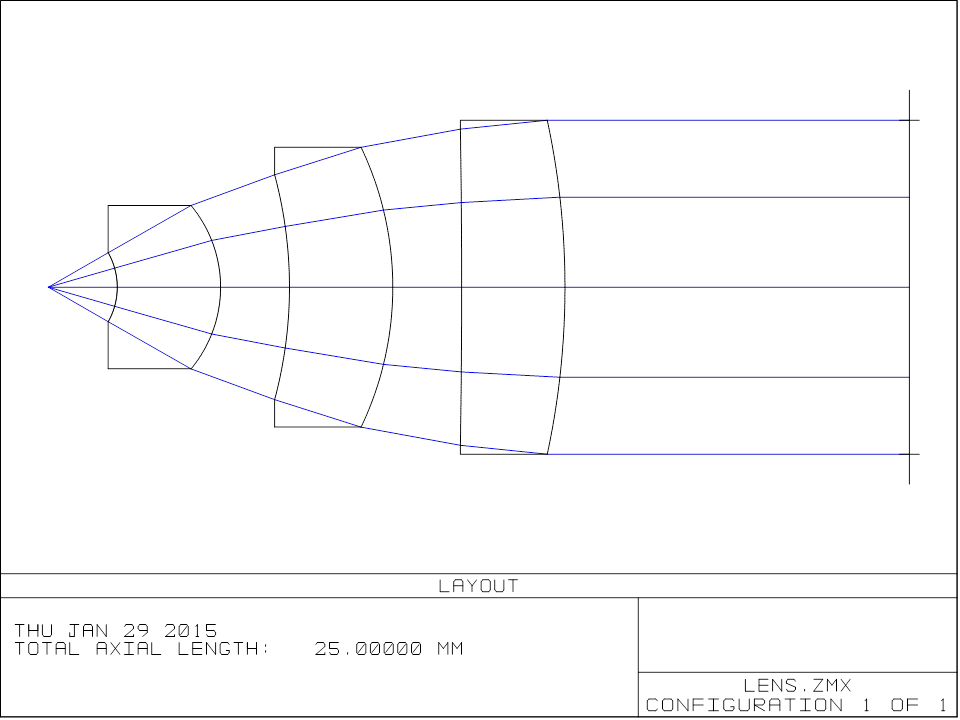
It may even be that someone like QED Technologies could build a huge custom aspheric to do your job with one lens: QED use magnetorheological finishing to build all kinds of shapes to magnificent surface quality. This is where the flow of an abrasive, ferromagnetic fluid is controlled through precise magnetic fields by a computer that uses the fluid to cut the surface, then automatically inspects the surface interferometrically then makes another polishing pass to automatically build pretty much any kind of surface you need. (DISCLAIMER: I do not work for QED nor have I done work for them and nor am I an investor in them: I simply find their processes fascinating: they are pretty much unique to QED and extremely accurate and effective. Unfortunately QED are having a hard time finding enough customers for their most excellent skills in such a specialised field).
Depending on your aberration needs, building this system from custom spherical or aspherical optics will be either very time consuming yourself or expensive if you need to buy the skills in. I would definitely try the Fresnel idea first, although this will be lossy.
Two comments worth noting:
From user ThePhoton
RE: "you could probably write a simple raytracer in Mathematica and use it to optimise the following general idea." I met a guy who did this once, and the result was Optica
My own copy of Optica cost several thousands of dollars but there are academic versions. You only need a very small subset for your problem.
From user J...:
For the price of a custom job like this, if aberrations were important, you could probably find a used 6" interferometer that would deliver an excellent collimated beam.
This is an excellent suggestion. 4" and 6" are standard sizes for interferometers: there are many collimators and beam expanders for them floating around. This site may be worth a look.
You will need to know that your output beam is diverging at $\frac{\sqrt{3}}{2}\approx 0.87{\rm NA}$ (numerical aperture) and this is what you want to collimate to a 4.52" diameter: this is the specification you must let suppliers know. The lens input - 0.87NA - is REALLY fast - rather faster than most interferometer collimators I've seen (that's optic speak - historically it comes from camera lenses: the bigger the numerical aperture, the more light is let in and thus the faster an image will be acquired).


