Why does this ball have potential energy at its lowest point?

Question

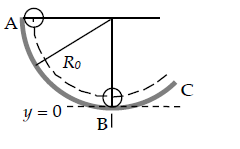
A ball of radius $r_0$ starts from rest at point $A$ on the inside of a track of radius $R_0$. The question is what will its speed be when it reaches the lowest point of the track, point $B$, assuming it is rolling without slipping?

My textbook has this as answer. We use conversation of mechanical energy to find the speed at the lowest point, and since the ball is rolling without slipping, we can use $\omega = v/r_0$. Call the zero level for gravitational potential energy to be the lowest point on which the ball rolls. Hence we have \begin{align*} mgR_0 &= mgr_0 + \frac{1}{2} m v_B^2 + \frac{1}{2} I \omega^2 \\ &= mgr_0 + \frac{1}{2} mv_B^2 + \frac{1}{2}(\frac{2}{5} mr_0^2) (\frac{v_B}{r_0})^2. \end{align*} It follows that $v_B = \sqrt{10/7 g(R_0 - r_0)}$.

My question is why do we have to add the factor '$mgr_0$' in the calculation? I thought all the gravitational potential energy was transformed into kinetic energy at the lowest point of the track? How can the ball have potential energy there when we set $y=0$ at that point?

Answer 1

To see why the $r_0$ term is needed, try to imagine the limit case where $R_0= r_0$. In that case the ball can not move at all and $$\frac{1}{2}m v_b^2+\frac{1}{2}I\omega ^2=mg(R_0-r_0)$$ gives the correct answer.

The change in potential energy is given by the vertical displacement of the center of mass of the ball and this is exactly $R_0-r_0$.

Answer 2

The answer is in the diagram. Look where the ball was initially. $R_0$ is the distance from $y=0$ to the center of the ball when the ball was at A. So, when the ball reaches the lowest point the center of the ball has to be at $y=0$ to have all the potential energy $mgR_0$ converted but the center of the ball is above B and by how much? $r_0$.

Answer 3

Let me first put the things otherwise :

The potential energy that actually transforms into kinetic is $mg(R_0 - r_0)$. If the book would have taken as zero level of gravitational potential energy, the lowest point that the center-of-mass of the ball could reach, i.e. $R_0 - r_0$, you wouldn't have your problem. Anyway, the equality between consumed gravitational potential energy and kinetic energy, looks as follows

$mg(R_0 - r_0) = \frac {mv_B^2}{2} + \frac {Iω^2}{2}$.

Answer 4

What you have to notice is that the Centre of Mass of the ball is at a distance $r_o$ from the zero potential energy coordinate, therefore the ball still has potential energy. (at the bottom most point). If instead, you considered a particle, the potential energy would effectively be zero.