Do muons and antimuons have different penetration depth in matters?

Question

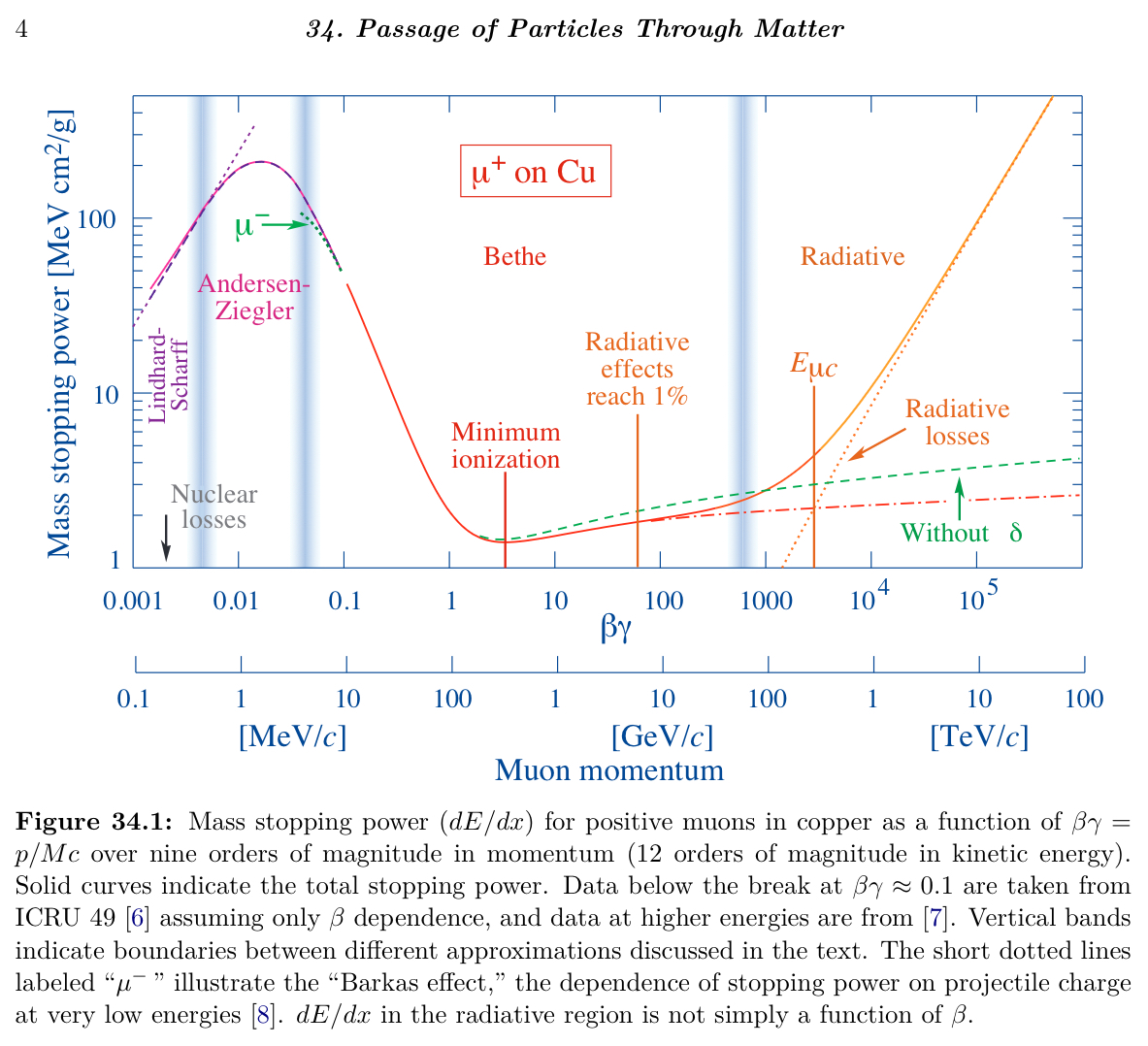
Muons are an ideal tool to study the interaction between charged particles and condensed matters. Besides electromagnetism, muons have very weak interactions with matters (unlike protons). Muons and antimuons have exactly the same mass, and neither particle can annihilate with ordinary matters (unlike positrons).

I am curious if muons and antimuons interact differently with matters due to the signs of their charges. Antimuons are positively charged. Because of its large mass, it should behave like protons. For example, naked protons are extremely polarizing and can form a strong covalent bond with whatever atoms (including helium). Muons should interact more weakly with electrons, but due to its mass, it can bind to atomic nuclei 200 times more strongly than electrons. Would such a difference affect their penetration depth?

Answer 1

There are two main differences that I can think of, both of which are due to the fact that the nucleus is positively charged.

1. Muons can form bound states with nuclei. Antimuons cannot. Thus, one would expect muons to have a shorter penetration depth because their cross section for capture would be larger.

2. Muon capture can lead to muons being converted into muon neutrinos in matter: $$ p^+ + \mu^- \to n + \nu_\mu \tag{1} $$ A similar process can also happen for antimuons, in principle: $$ n + \mu^+ \to p^+ + \bar{\nu}_\mu \tag{2} $$ However, this latter process is much less common because the neutrons are in a positively charged nucleus, which would repel the antimuons. The antimuons would either have to tunnel through an energy barrier or have a significant amount of kinetic energy (or both.) In contrast, the positively charged nucleus will actually attract the negatively charged muons, enhancing this effect for "free" muons.
   
   Whether this latter process is frequent enough to make a difference in the penetration depth would depend on the specific material involved. In particular, the relative stability and binding energy of the parent and daughter nuclides would affect the cross section for this type of capture.

Answer 2

Dependence of muon stopping power on muon charge has been known since the 1950s. The charge dependence is negligible for a muon momentum above 10 MeV/c. Here is a figure from the current Particle Data Group literature review (PDF), who cite Barkas et al. (1956).

Barkas et al. cite a private communication from Fermi which models each electron-meson scattering event (using Mott theory) as transmitting slightly less impulse to a negative meson than to a positive meson. They model this as a slightly larger "effective mass" for the negative mesons, at the part per thousand level. Note that we no longer refer to muons as "mesons," but electromagnetism doesn't care about whether there is strong interaction or not.

Note also that negative muons are more penetrating than positive muons, which was the opposite of my expectation.

Answer 3

I'm going with "no". $\mathrm{d}E/\mathrm{d}x$ is about a charge interacting with atomic electrons, and the sign doesn't matter.

Regarding forming exotic atoms, I'm taking the energy scale to be a Rydberg (on the order of $13.8 \ \text{eV}$), while $\mathrm{d}E/\mathrm{d}x$ is $\text{MeV}/\text{g cm}^{-2}$, so we're talking $10 \ \text{microns}/\text{g cm}^{-2}$, which may be a factor, but still is a "No".

Note to down voters: yes Rob's answer is better, but 10 MeV is trivial. Even cosmic rays are 1000 MeV, and anything from an accelerator is orders of magnitude higher.

The stopping power is, as I said, $100\,{\rm MeV\cdot cm^2/g}$, so a 10 MeV muon stops after $10 {\rm MeV\cdot cm^2/g}$. With lead being on the order of $10{\rm g/cm^3}$, that muon stops in 100 microns.

And the difference, per @rob, is on the order of 1 part in a thousand, so 100 nm. Have you ever stacked lead bricks (standard 2" x 4" x 8", 25 pounds each) bricks for 2 days around a detector? That less than a scratch, and they do scratch easily.

If you're designing shielding for an instrument in a muon beam environment, the sign of the muon charge is not even going to be considers. The $511\,{\rm MeV}$ gamma's from the $e^+$ decay might be, though (but then you're not shielding muons).

So the practice answer is still: "no".

Answer 4

Well the charge plays a big role.Since antimuons have positive charge they pass through matter without effort in the process of breaking bonds of the crystal(by ionising some atoms) because the positive charge of the atom is at its center,it is like the Rutherford experiment I expect a very similar pattern.