How long does it take to achieve buoyancy in a body of water?

Question

Non-Engineer here. Question:

As an example, the Canal du Centre Water Bridge, in Belgium.

Is the weight on column C greater than the weight on columns A and E?

If no, because of buoyancy, how fast is "equilibrium" achieved as the boat moves through the water, taking into account that buoyancy distributes the weight equally to the entire body of water that it is in, which in the case of a river, could be hundreds of miles?

If the boat was not in the water, but was lowered into the water over column C, how long before a weight change could be measured at columns A and E? I assume it cannot be instantaneous, that there must be some time delay as the "force" is distributed through the medium (water).

I assume the buoyancy "force" could be plotted on a graph as a decreasing force over distance, until it is effectually (though never actually) zero, but how far is that, and how long does it take to reach that state?

I am not an engineer, so I do not even know if I have used the right terminology to describe this.

Answer 1

If the boat were lowered from above into the position shown in your diagram, then I would expect the load to spread out in both directions as a soliton surface wave. The speed of the wave would depend on the cross-section of the aqueduct, but I imagine it would be some single-digit number of meters per second. https://www.youtube.com/shorts/yQ6llZucY-Q

Answer 2

A lovely problem! I have always loved the fact that for stationary ships the weight on the viaduct supports is indifferent to the ship being there or not.

The issue here is that a moving ship creates waves. In a shallow channel the waves have speed $\sqrt{gh_0}$ where $h_0$ is the undisturbed depth, so some parts of the canal have not yet noticed that the ship is moving. A second issue is that the water squeezed under the moving ship has a lower pressure that expected because of Bernoulli. Now Bernoulli generates a lot of nonsense in physics posts, but I believe (I.e. I have not worked it out for myself) that this can causes the ship to squat and so lowers the pressure on on the canal bed. It seems hard to figure exactly. So my "answer here" is not really a proper "answer", but it suggests that numerics may be needed if you are really designing a viaduct for fast moving ships.

Answer 3

If there was no water present, gravity would pull the boat down. Water pushes the boat up, with part of the boat underwater and part above. This means the boat makes a hole in the water the shape of the hull.

You could take the boat out of the hole. The water around the hole would push inward and quickly fill in the hole.

One way to keep this from happening would be to fill the hole with water until the surface was level. If the surface is level, water doesn't go anywhere.

The weight of enough water to fill in the hole would push down on the hole just as hard as the weight of the boat did. As far as the bridge is concerned, it doesn't matter whether there is a boat or water filling the hole. The weight over column C is the same either way.

This is how it works when water is still. If water is moving, there are additional forces. It is the same for a rock. If you set a rock on an object, the force is the weight of the rock. If you throw the rock at the object, the force on the object is greater. For a floating or slowly moving boat, consider the water to be still.

Answer 4

Speed of Sound

Equilibrium is not achieved at the speed of sound, but the speed of sound in water is literally the speed of "water causality", or the fastest you can send information through water using only the bulk water molecules themselves as messengers. In general, any disturbance to the water will cause waves, and equilibrium is achieved when enough waves redistribute the mass to an equilibrium state. As you can imagine, the duration of the settling time depends on the magnitude of the disturbance. If you drop the ship into the water while suspended above, it will create enormous waves that will slosh around considerably for some time. If the ship is already in the water, but moving at a few knots, then the waves will be much smaller and gentler.

Even a slowly moving ship will disturb the water for a long time (just watch the wake until you can't see it any more), so in general, equilibrium will take a long time. Even so, the small waves you can see on the surface will not produce large differences in the forces you see on the columns. I don't have a formula for you, but if I had to guess, I'd say that the "mass anomaly" decreases exponentially. I define "mass anomaly" to be the variance of the water depth, or how much different points in the water surface vary from the mean elevation. So the waves probably transfer the bulk of the water very quickly to where it will end up, and the water takes longer and longer to smooth over at smaller scales.

Answer 5

Imagine we have canal locks on either side of the middle column $\text{C}$ just before columns $\text{B}$ and $\text{D}$. If we lower a ship into this enclosed section, the water will rise in that section, and the weight of the ship will register as an increased stress on column $\text{B}$ with a slight increase in the stress measured in columns $\text{C}$ and $\text{D}$ (and the other columns) due to simple mechanical distribution of stress. When the locks are opened, the high level of water in the centre section will flood outwards in both directions as a wave at typical finite channeled water wave speeds. Since canals are designed to maintain a constant water level, the water level will eventually return to its normal level before the ship is lowered, and the stress measured in all the columns will return to what they were before the ship was added. As others have mentioned, the ship displaces its own water's weight to float, so the total weight on the columns is the same with or without the ship when everything has settled to equilibrium. In the dynamic case of a ship progressing along the aqueduct, there is a slight wave in front of the ship and a slight dip in the water level behind the ship because of the delay caused by the time it takes the water to backfill the hole left behind the ship as it moves. Very sensitive weight detectors in the columns could conceivably measure this moving fluctuation in the water levels as the ship moves along.

Answer 6

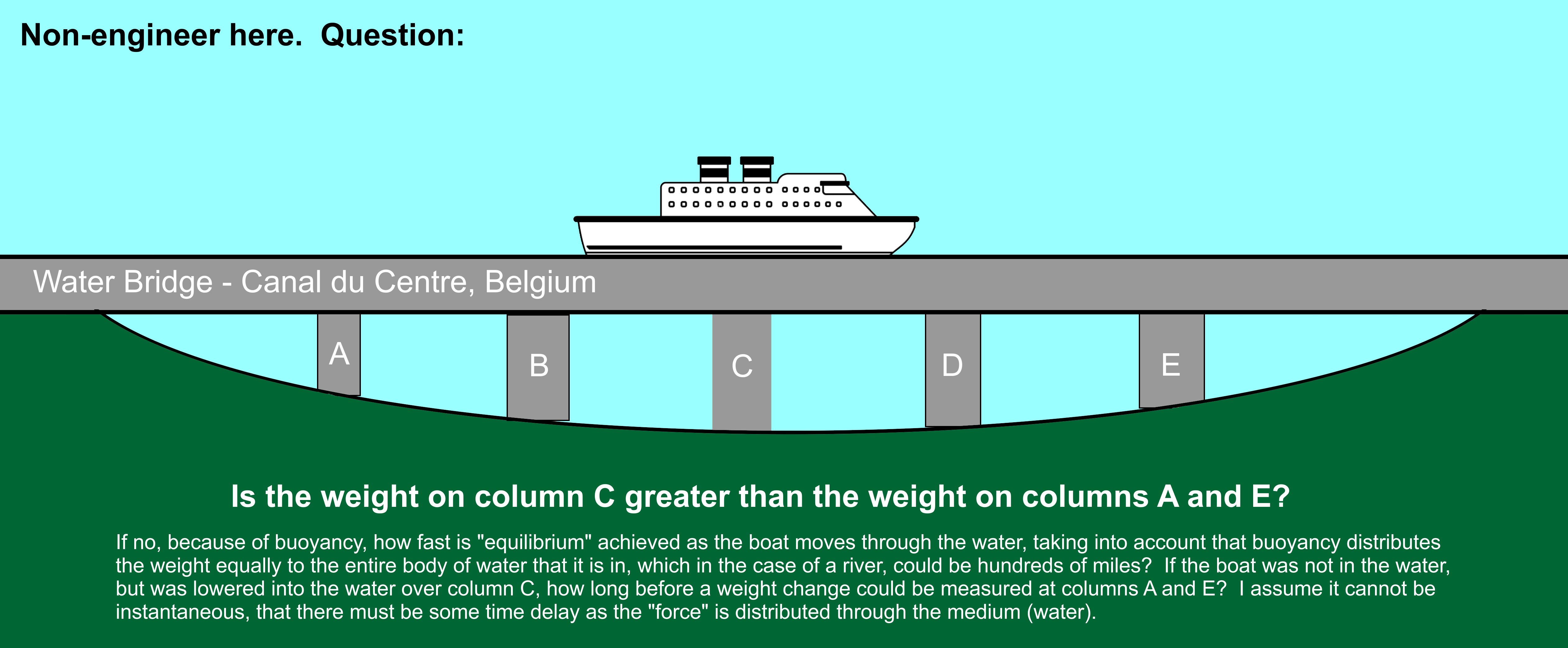
There are two separate pieces here. One is what happens to the bottom pressure profile as a ship transits at a steady rate. The second is what happens when a there is a sudden displacement to the fluid at one location. The latter is governed entirely by the fluid properties and the basin geometry. It is basically a free surface gravity wave problem, albeit a potentially difficult one if linear wave theory is not reasonable. And as mentioned, there may be a soliton component in the solution. Solitons are shock waves. They are highly entropic. The linear wave theory can be employed behind them, though.

In the case of the ship transiting the canal, the pressure pattern will advance at the speed of the ship. The pattern is very characteristic of each vessel, and undersea hydrophone networks can identify exact ships, and their speed and direction, from just the pressure signature - independent of the acoustical signature.

If you have access to Leo Lazauskas's Michlet software, the paid version had the ability to predict the induced bottom channel pressure profiles of ships in channels. Leo is no longer with us, and the software is not supported any longer. But it was a cool piece of engineering code with a popular freeware version.

In general, all these are awkward boundary value integral problems. Michelle's method, which is basically a zeroth order Green's theorem solution to the linear wave equation, is usually evaluated as a seven-integral over the hull surface with appropriate boundary conditions on the channel. The geometry of the ship is broken into flat patches. The size and orientation is converted into an equivalent source or sink, and a five-integral computes the wave field from each one. The wave fields of each hull patch are then added together. With reflections off the channel walls, the process becomes iterative. Leo's Michlet employs thin ship theory to produce the Havelock source geometry.

The article below by Leo has bottom pressure signatures.

https://www.researchgate.net/publication/266298258_The_Hydrodynamic_Resistance_Wave_Wakes_and_Bottom_Pressure_Signatures_of_a_5900t_Displacement_Air_Warfare_Destroyer/download?_tp=eyJjb250ZXh0Ijp7ImZpcnN0UGFnZSI6Il9kaXJlY3QiLCJwYWdlIjoiX2RpcmVjdCJ9fQ

And here's a video of your "drop a boat into the channel" example. In this case, the result is strongly a solitary wave. There is little energy anywhere except in the one wave pulse. This is because in the geometry here, the dominant solution to the wave equation is one dimensional, and solutions with an odd number of spatial dimensions have energy confined to the advancing wave front, while solutions with even numbered dimensions distribute energy over the entire area behind the wave front.

https://www.google.com/search?q=ship+bow+wave+in+river+soliton+video&rlz=1C1RXQR_enUS1086US1087&oq=ship+bow+wave+in+river+soliton+video&gs_lcrp=EgZjaHJvbWUyBggAEEUYOTIHCAEQIRigATIHCAIQIRigATIHCAMQIRigAdIBCTI2ODY5ajFqN6gCALACAA&sourceid=chrome&ie=UTF-8#fpstate=ive&vld=cid:f076ab56,vid:w-oDnvbV8mY,st:0