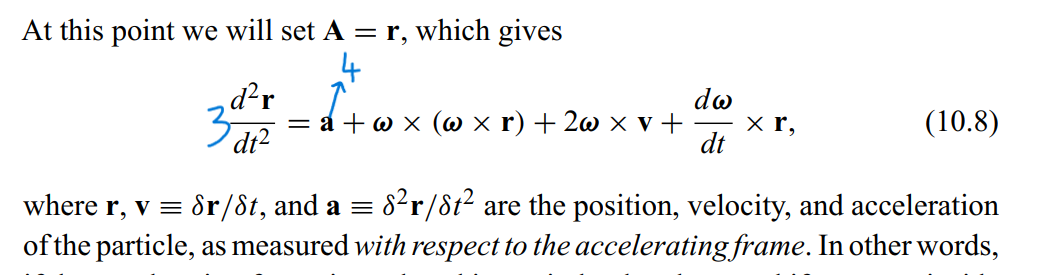but isn't dA/dt the real thing, the physical thing?
Let's write $\frac{d\mathbf{A}}{dt}=\frac{d}{dt}\mathbf{A}(t)$. $\mathbf{A}(t)$ is just a symbol, we use it to represent the physical vector (which in this context can be viewed as a literal arrow from an origin in space at a given time).
I know 2 is what the non-inertial frame measures
Sure, let's look at a primitive example, say you are dropping a ball on the equator of an asteroid rotating at angular velocity $\omega$. An inertial observer measures the position of the ball as $\mathbf{r}(t)=\left(h_0-\frac{1}{2}g_at^2\right)\mathbf{\hat{y}}'$, where the primes denote the inertial observer.
In the asteroid's frame we have $\mathbf{\hat{x}}=\cos(\omega t)\mathbf{\hat{x}}'+\sin(\omega t)\mathbf{\hat{y}}'$ and $\mathbf{\hat{y}}=-\sin(\omega t)\mathbf{\hat{x}}'+\cos(\omega t)\mathbf{\hat{y}}'$. So in the asteroid's frame it sees: $$\mathbf{r}(t)=\sin(\omega t)\left(h_0-\frac{1}{2}g_at^2\right)\mathbf{\hat{x}}+\cos(\omega t)\left(h_0-\frac{1}{2}g_at^2\right)\mathbf{\hat{y}}$$
See this visual. The asteroid's frame is the solid black lines that the dashed lines are projecting to, and the inertial observer's frame is the global stationary gridlines.
You can see that to find $\frac{d}{dt}$ of $\mathbf{r}(t)$ we need to see how our $\mathbf{\hat{x}}$ and $\mathbf{\hat{y}}$ change with time, so we use the chain rule.