I am curious if there are results available for the Kitaev model with a magnetic field -- in his 2006 paper, Kitaev obtains the form of the effective hamiltonian (Eq. 46 in https://arxiv.org/abs/cond-mat/0506438), however does not give the precise prefactors. Also wondering about the 2nd order contribution (which is proportional to the identity) -- I just would like to see what would be the correct counting.
Thanks!
Yes, the precise result can be found in Eq. (30) of our paper arXiv:1109.4155:
$$\kappa = \frac{h_xh_yh_z}{8 u_0^2 J^2},$$
where $u_0$ is a numerical factor obtained by solving the mean field equation numerically. Kitaev mentioned in his paper that $\Delta E = |u_0J|\approx 0.27|J|$ (i.e. $u_0\approx -0.27$), and we provide a more accurate result $u_0\approx −0.262433$ in Eq. (27) of our paper. The explicit perturbation path is illustrated in Fig. 5(a) of our paper (which is a 3rd order perturbation, not a 2nd order one, as stressed in Kitaev's paper).
Let me briefly outline the derivation below. We start from an isotropic Kitaev honeycomb model with a perturbative Zeeman field ($|\boldsymbol{h}|\ll J$),
$$H=-J\sum_{\langle ij\rangle}S_i^{a}S_j^{a}-\sum_{i}\boldsymbol{h}\cdot\boldsymbol{S}_i,$$
where $a=1,2,3$ depends on the type ($x,y,z$) of the link $\langle ij\rangle$. Introduce four Majorana spinons $\chi_i^\alpha$ ($\alpha=0,1,2,3$) on each site $i$, defined by the anticommutation relation $\{\chi_i^\alpha, \chi_{j}^{\beta}\}=\delta_{ij}\delta_{\alpha\beta}$ (note the unusual normalization of the Majorana operator here). Under the gauge constraint (on-site constraint) $\chi_{i}^0\chi_{i}^1\chi_{i}^2\chi_{i}^3=1/4$, the spin operator $\boldsymbol{S}_i$ can be written in terms of the spinon bilinear form as
$$\boldsymbol{S}_i=\frac{i}{2}\Big(\chi_i^0\boldsymbol{\chi}_i-\frac{1}{2}\boldsymbol{\chi}_i\times\boldsymbol{\chi}_i\Big),$$
where the vector $\boldsymbol{\chi}_i=(\chi_i^1,\chi_i^2,\chi_i^3)$ is made of the last three components of the Majorana fermion. We can see that the $\chi^0$ ($c$-fermion) differs from $\chi^{1,2,3}$ ($b$-fermion) in this fractionalization scheme. This difference is also reflected in the mean-field Hamiltonian $H_\text{MF}$. In the unperturbed limit $\boldsymbol{h}=0$, $H_\text{MF}$ can be obtained by substitute the expression for $\boldsymbol{S}_i$ to the spin Hamiltonian and take the mean-field decomposition described by Kitaev:
$$H_\text{MF}=J\sum_{\langle ij\rangle}\big(\text{i}u_a \chi_i^0\chi_j^0+\text{i}u_0\chi_i^a\chi_j^a\big),$$
where the bond parameter $u_\alpha=\langle\text{i}\chi_{i}^\alpha\chi_{j}^\alpha\rangle$ (for $\alpha=0,1,2,3$) is determined self-consistently from the Majorana fermion correlation on the mean-field ground state (note $a=1,2,3$ is fixed by the link type, not a dummy index to be summed over). It is found that the mean-field solution reads $u_a=1/2$ and $$u_0=-\frac{1}{3N}\sum_{\boldsymbol{k}\in\text{BZ}}\big|e^{\text{i}k_y}+2e^{-\text{i}k_y/2}\cos(\sqrt{3}k_x/2)\big|\approx -0.262433,$$ where $N$ is the number of sites (we can evaluate the summation numerically on a finite lattice and then take the thermodynamic limit $N\to\infty$). The band structure of the spinon can be obtained by diagonalizing the mean-field Hamiltonian, as shown below:
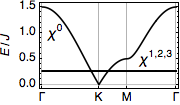
One can see that the $\chi^0$ fermion is itinerant and has a gapless spectrum. But the $\boldsymbol{\chi}=(\chi^1,\chi^2,\chi^3)$ fermions are dimerized on the corresponding type of links and are therefore gapped (as the flat band). The energy gap for $\boldsymbol{\chi}$ fermions is $\Delta E=|u_0J|$.
If we are only interested in the low energy physics, we can neglect the high-energy $\boldsymbol{\chi}$ fermions. However, once the Zeeman field is introduced to the system, the mixing is turned on between the low-energy $\chi^0$ and high-energy $\boldsymbol{\chi}$ fermions (and also mixing among the components of $\boldsymbol{\chi}$). Thus a perturbation pathway illustrated below becomes possible:
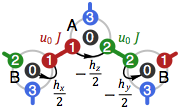
which results in a 2nd nearest neighbor coupling between the low-energy $\chi^0$ fermion,
$$H_{\text{MF},0}=\text{i}u_a J\sum_{\langle ij\rangle} \chi_i^0\chi_j^0+\text{i}\kappa\sum_{\langle\!\langle ij\rangle\!\rangle}\chi_i^0\chi_j^0,$$
with the coefficient $\kappa$ given by the 3rd order perturbation (see this Wikipedia page for the 3rd order perturbation formula)
$$\kappa=\Big(\frac{h_x}{2}\Big)\frac{1}{u_0J}\Big(-\frac{h_z}{2}\Big)\frac{1}{u_0J}\Big(-\frac{h_y}{2}\Big)=\frac{h_xh_yh_z}{8u_0^2J^2}.$$
The 2nd neighbor coupling term $\kappa$ breaks the time-reversal symmetry and gaps out the low-energy fermion $\chi^0$. The gapless Kitaev spin liquid is then driven into the non-Abelian phase with the Ising topological order.
