Why is linear velocity represented as cross product of angular velocity of the particle and its position vector? Why not vice versa? (Consider rigid body rotation)
$\begingroup$
$\endgroup$
3
-
2$\begingroup$ Are you asking why velocity is given by $v = \omega \times r$ rather than $v = r \times \omega$? $\endgroup$– John RennieCommented Mar 19, 2015 at 7:18
-
$\begingroup$ yes!if you know the answer please let me know! $\endgroup$– user74370Commented Mar 19, 2015 at 7:23
-
4$\begingroup$ I think it's just because we conventionally take anti-clockwise angles to be positive. I can't think of any deeper reason. $\endgroup$– John RennieCommented Mar 19, 2015 at 7:45
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
6
OK, I'm assuming you want the formal proof of this well known kinematics formula! So here goes:
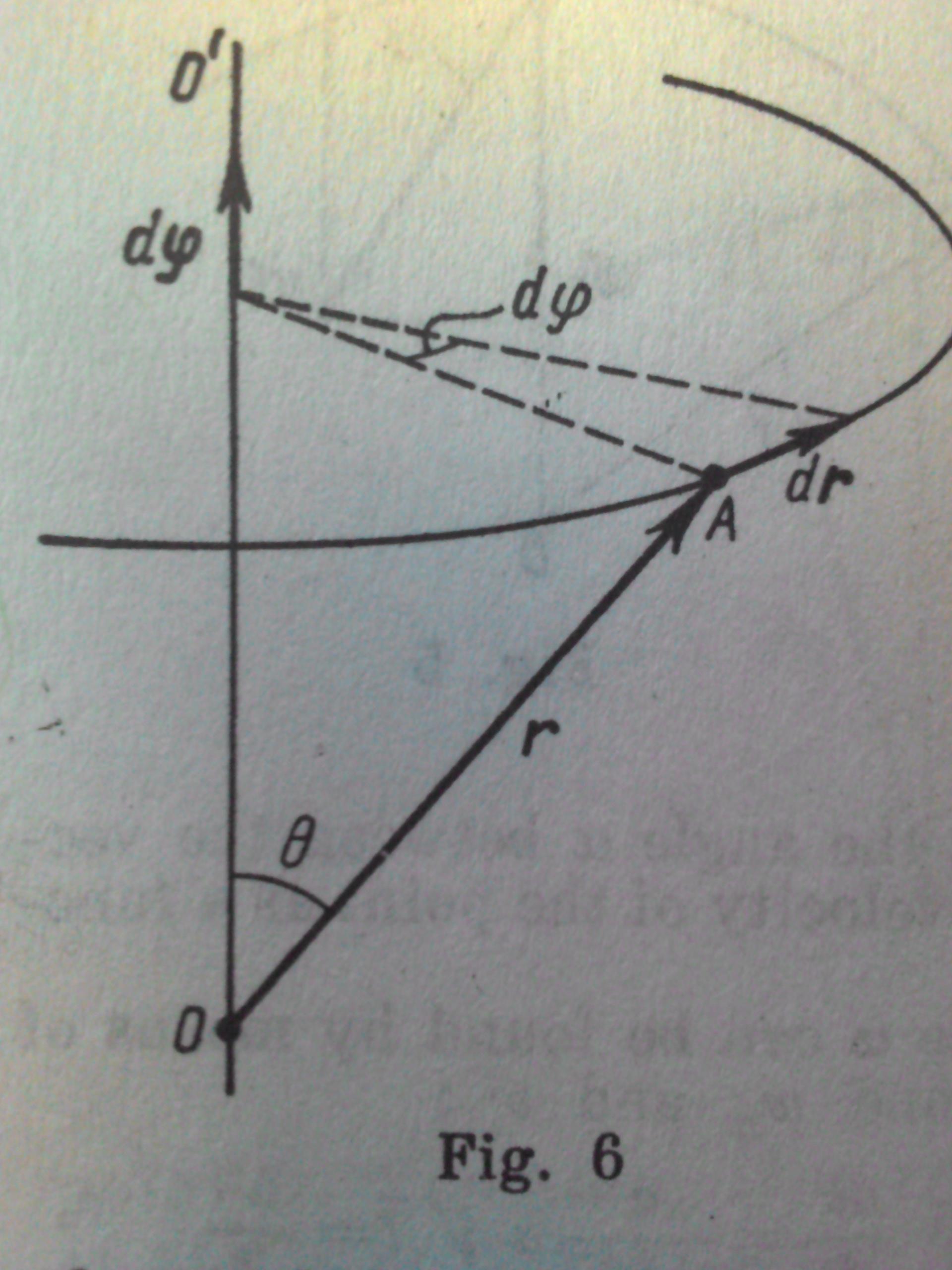
Let the particle rotate about the axis OO' ... Within time interval $dt$ let its motion be represented by the vector $d\varphi$ whose direction is along axis obeying the right-hand-corkscrew rule, and whose magnitude is equal to the angle dφ.
Now, if the elementary displacement of particle at a be specified by radius vector $r$,
From the diagram, it is easy to see that, for infinitesimal rotation, $dr= d\varphi\times r \tag{1}$
By definition, $ω = dφ/dt$
Thus taking the elementary time interval as $dt$, all given equations surely hold!
Thus we can divide both sides of the equation $(1)$ by $dt$ which is the corresponding time interval!
So we get $dr/dt = dφ/dt \times r$ of course $r$ value won't change WRT the particle and axis, so $r$/dt is essentially $r$!
So result is, $$\boxed{v = ω \times r}$$
-
$\begingroup$ And just so u know, if u tried v = rXω, that would be fundamentally wrong, bcuz of how the direction of ω us assumed by the right hand rule convention!, then of course you would obtain an illogical result, anyway , that's if u go by convention!! $\endgroup$ Commented Mar 19, 2015 at 7:46
-
$\begingroup$ Ok then basically i've been questioning the right hand screw rule itself..and do you know a proof for the right hand screw rule? Btw this is a good approach :-) $\endgroup$– user74370Commented Mar 19, 2015 at 7:56
-
2$\begingroup$ The right hand screw rule is just convention; we could define the cross product the opposite direction and it would be fine as long as the same new version was applied universally $\endgroup$– danimalCommented Mar 19, 2015 at 10:27
-
$\begingroup$ @VIVID, Sir, would you please elaborate why $dr=d\phi × r$ $\endgroup$– RAHULCommented Mar 29, 2022 at 9:47
-
$\begingroup$ @Rahul If you treat that as a normal triangle (i.e. nothing about infinitesimal rotation) $dr = r\sin\phi$. But $\sin\phi \approx \phi$ for sufficiently small angles $\endgroup$– VIVIDCommented Mar 29, 2022 at 10:48
$\begingroup$
$\endgroup$
$\omega$ is a axial vector, not an ordinary or polar vector, and its sense depends on the handedness of the coordinate system. For a right handed coordinate system v=ω×r. See the textbook Symon Mechanics.
answered Nov 22, 2020 at 1:01