Why light can't escape a black hole but can escape a star with same mass? [duplicate]

Question

I'm new to astronomy and was wondering why light can't escape from a black hole but can escape from a star with the same mass. In theory, the gravity of a star 100x the mass of the sun, and the gravity of a black hole 100x the mass of the sun are the same, so why can light escape from the star but not from the black hole? even if the force of gravity is the same in both cases. Is it just because the black hole is smaller?

Answer 1

yes, it is because the black hole is much more dense which means it packs the same mass & gravitational pull into a much smaller diameter. This means its surface gravity yields an escape velocity equal to the speed of light. For the same-mass star, its "surface" is much farther away from its center and if you were standing on that "surface", the surface gravity there would be crushingly huge but not enough to yield c as the escape velocity.

Oh yes and you would be instantly fried to a crispy crisp.

To make the surface gravity of the sun yield an escape velocity of c would require you to squeeze it down to a diameter of 2.5 kilometers. That's really dense!

Answer 2

There are a lot of ways to explain this, with various levels of accuracy. I'm going to choose a way to justify this, in the spirit that the original question seems to not come from a place with a lot of familiarity with general relativity. So, let's use Newtonian mechanics

First, let's calculate the speed required for an object of mass $m$ to escape from an object with mass $M$, and a radius $R$. An escaping object will be leaving the surface at some speed $v$. We want to find the minimum speed required to "escape". If this is the case, at "late times", the object will have exactly been slowed down and stopped by gravitational forces, and will be infinitely far from the mass. So, conservation of energy tells us:

$$\begin{align} E_{i} &= E_{f}\\ \frac{1}{2}mv^{2} - G\frac{Mm}{R} &= \frac{1}{2}m(0)^{2} - G\frac{mM}{\infty}\\ v^{2} - \frac{2GM}{R} &= 0\\ v &= \sqrt{\frac{2GM}{R}} \end{align}$$

So, if $M$ is fixed, the smaller $R$ is, the larger the speed required to escape the object, so it is harder to escape from a dense object, even before one considers general relativistic effects.

It even turns out, thanks to a lot of mathematical coincidences that should not be taken too literally, that you do, in fact get the correct answer $\frac{2GM}{c^{2}}$ for "the radius of an object whose escape velocity is $c$."

Answer 3

Yes, it's just because the black hole is smaller. The only mass factor relevant to the trajectory of a particle in a spherically symmetric mass distribution is the total mass that is closer to the center of mass than the particle. For any given photon at any given radius in Schwarzschild coordinates, the mass "under" the photon is much less than the mass associated with a black hole with that radius. Consequently, a photon emitted near the center of a 100 solar mass star is able to propagate outwards (although an individual photon will be almost immediately absorbed in the dense hot medium). The associated energy is transported as heat from the core to the surface after many interactions, in which photons are scattered, absorbed, and emitted.

Answer 4

This relates to the shell theorem:

If the body is a spherically symmetric shell (i.e., a hollow ball), no net gravitational force is exerted by the shell on any object inside, regardless of the object's location within the shell.

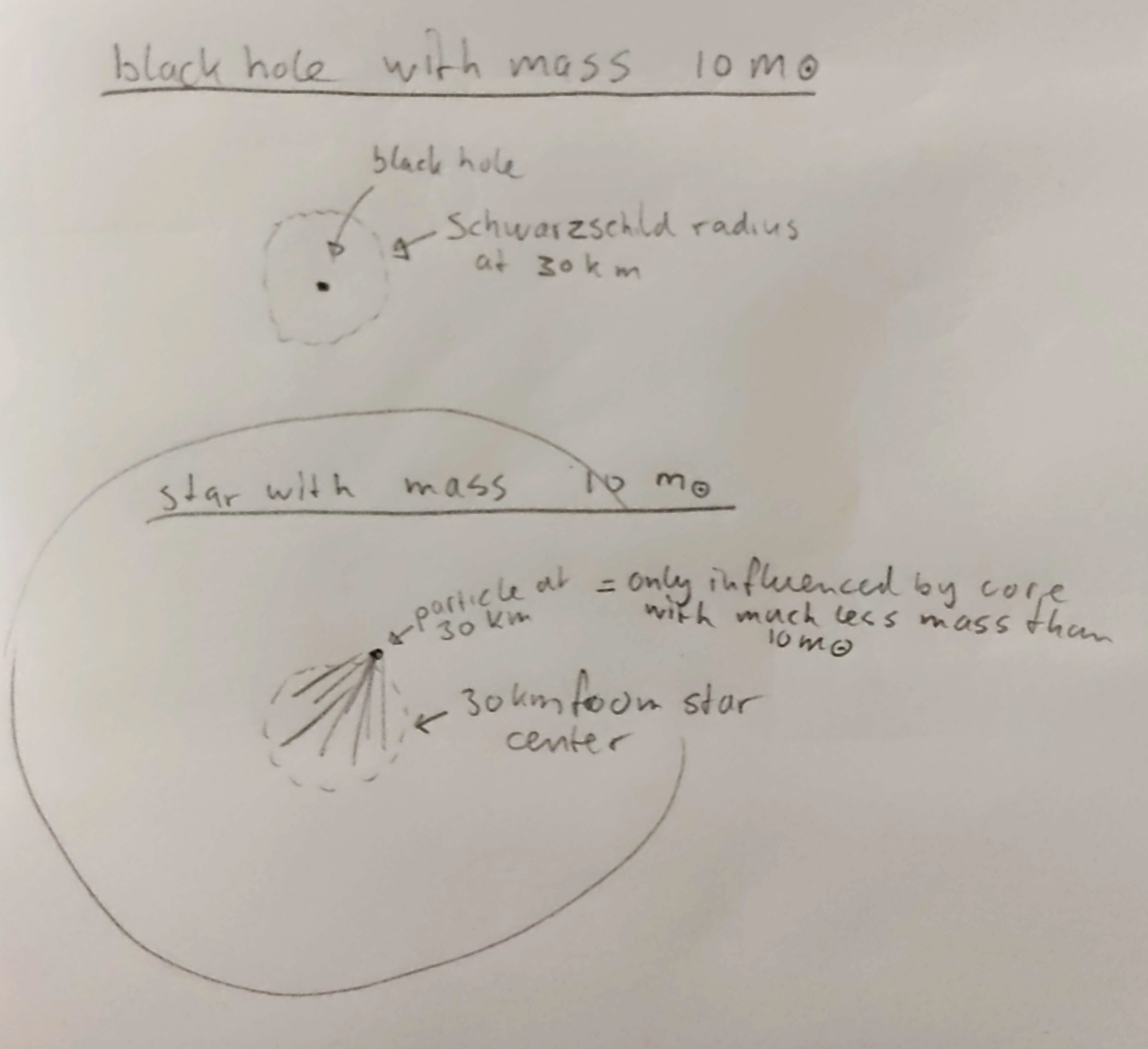
So say you regard a stellar mass of $10m_{\odot}$, then the Schwarzschild radius is 30 km.

• When 'you are' at 30km distance away from the centre of a $10m_{\odot}$ black hole then you are within the Schwarzschild and light can not escape from that point.

• When you are at 30km distance away from the centre of a $10m_{\odot}$ star. Then a lot of that star's mass is in a shell around you and the gravitational effects cancel.

Answer 5

The answer is that the black hole is more compact and so attraction at the surface is higher.

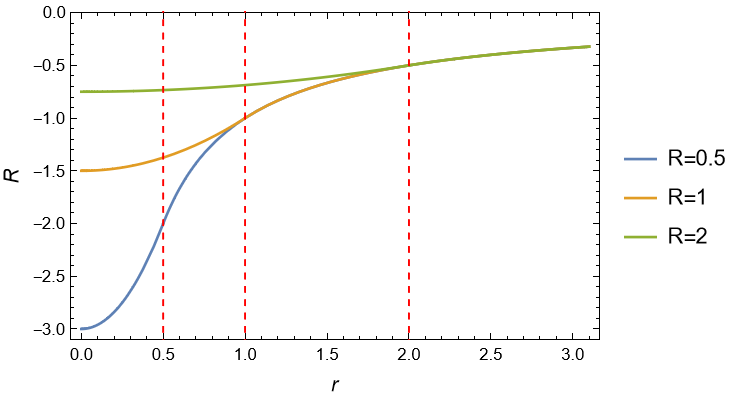
If you have two stars, having the same mass $M$ but different sizes, the potential outside the stars is written as $$U(r)=-\frac{GM}{r}$$ The potential inside the stars is different and it is smaller than the potential outside the star. If we assume that the stars have constant density, which is a very bad assumption, we can write an expression for the potential inside the star $$U(r)=\cases{-\frac{GM}{r}&$r\geq R$\\-\frac{GM(3R^2-r^2)}{2R^3}&$r<R$}{}$$

It will look something like this for $GM=1$:

The biggest force occurs at the steepest slope of the potential. So given different stars of the same mass, the one which is most compact has the largest max force. To describe light falling into a black you would have to replace force with spacetime curvature but the reasoning stays the same.

Answer 6

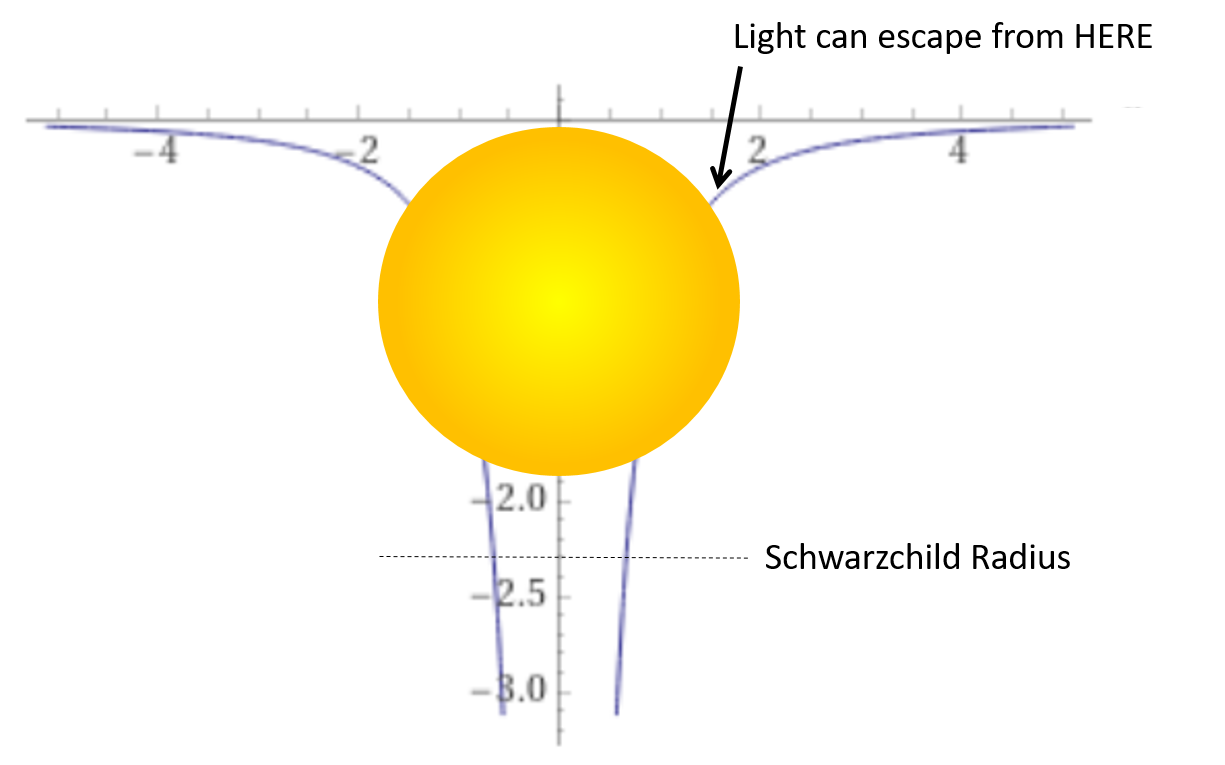
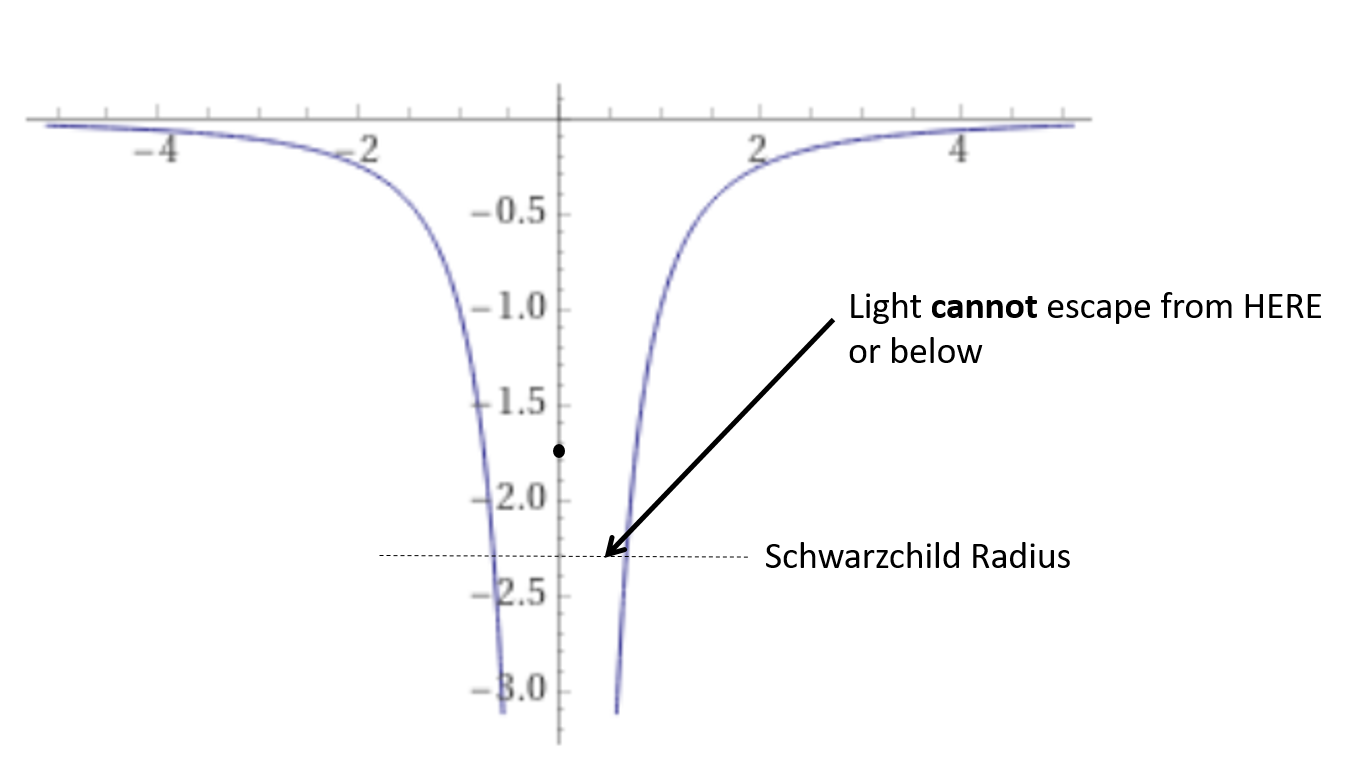
It seems like a picture might give the best answer. A $100M_{\odot}$ star and a $100M_{\odot}$ black hole generate identical gravitational potentials in the surrounding spacetime. But the star has a finite size, and that potential ends when it runs into the surface of the star, before the curvature gets extreme enough to trap any light trajectory. It's like venturing down a steep hill, but stopping before you get to the point where it is so steep you cannot climb back out.

Whereas, a black hole is a point object with zero size, so while it generates the same potential mathematically, a light ray can travel much closer to the source of mass, where the curvature is more extreme.

(To be clear, the gravitational potential curves shown are not mathematically correct. It is a conceptual picture)

Answer 7

The reason is density. Because as the density increases, you're more closer to the barycenter/center of gravity, which makes the gravity stronger because gravity is inversely proportional to the distance as gravity is a distant-dependent force like the van der walls force. Like at an 1 solar mass blackhole, the surface gravity or the gravity at the event horizon is 1.6 trillion g whereas for Sol the gravity at the surface is just 273 m/s because you're only 2.99 km away (which is the swarzchild radius of the sun) from the barycenter which is the singularity, note that as the swarzchild radius is inversely proportional to surface gravity or vice versa thus larger blackholes have less surface gravity .