Magnet falling through tube

Question

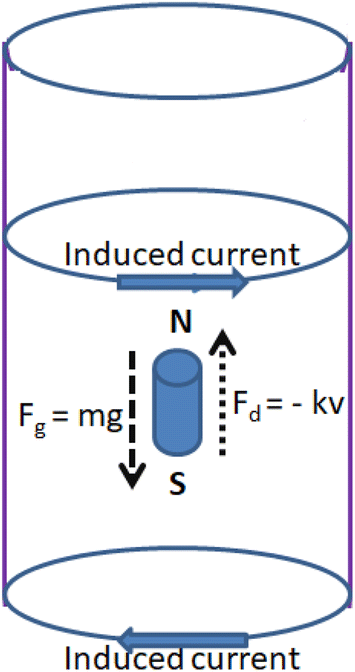
When you drop a magnet in a tube, the magnet is slowed down by induced Eddy currents due to the change in flux (repulsion from below and attraction from above).

We know that $\epsilon = -\frac{d\Phi}{dt}= IR$, where $\epsilon$ is the emf.

We know that if you use an aluminium tube instead of a copper tube (with the exact same dimensions), then the magnet will fall faster through the aluminium tube than the copper tube, because the aluminium tube has a higher resistance than the copper tube, thus the induced Eddy currents are weaker for a given $\epsilon$ and the effect is weaker, so the magnets will fall faster.

I understand this explanation, but at the same time, if the magnet falls faster, then the emf is greater right? Since the emf here is proportional to the velocity of the magnet (the drag force $F_d$ is also proportional to the velocity). If the magnet falls faster, then the flux change is greater and thus the emf is greater. So why doesn't a greater emf compensate for the greater resistance? Why does the magnet still fall faster through the aluminium tube than the copper tube?

Note: a terminal velocity will be reached in both cases. The terminal velocity for aluminium tube is higher than the terminal velocity for the copper tube.

Answer 1

Perhaps a simple analogy will help. Consider the terminal velocity of a rigid, uniform ball. As it accelerates downward, air resistance (the drag force) increases (since it depends on velocity), and terminal velocity is reached when the air resistance equals the weight of the ball.

Now consider a heavier ball of the same size. How does the terminal velocity compare to that of the lighter one? Since the ball is heavier, we might think the terminal velocity will be higher. But that would result in higher air resistance. Does that mean this heavier ball will fall slower than than the lighter one? Maybe they will fall equally fast?

The answer is no. Sure, the air resistance will be higher, but there will be a happy medium at a higher terminal velocity where both the air resistance and weight are higher than those with the lighter ball, and thus, cancel out. If the terminal velocity were lower than (or equal to) that of the lighter ball, the air resistance would be lower (or the same), and would not balance the higher weight. This is of course inconsistent with zero net force required for the ball to reach terminal velocity.

Back to your problem, in this experiment as it's usually done, air drag $F_d$ is completely negligible compared to the force $F_m$ on the magnet due to the induced magnetic field. But we don't have to neglect $F_d$ to arrive at the right conclusion. Crucially, note that at a given velocity, $F_m$ is greater for copper than for aluminum, since the induced currents and the resulting magnetic fields will be greater.

When terminal velocity is reached, $$F_g = F_m(v) + F_d(v)$$ where $F_g$ is weight. Since the weight is the same in both cases, we can write $$F_m^\text{Cu}(v^\text{Cu}) + F_d(v^\text{Cu}) = F_m^\text{Al}(v^\text{Al}) + F_d(v^\text{Al})$$ or $$F_m^\text{Cu}(v^\text{Cu}) - F_m^\text{Al}(v^\text{Al}) = F_d(v^\text{Al}) - F_d(v^\text{Cu}).$$ Suppose $v^\text{Cu}=v^\text{Al}$. The right hand side is then zero, but since $F_m^\text{Cu}(v) > F_m^\text{Al}(v)$, the above equation isn't satisfied, so $v^\text{Cu}\ne v^\text{Al}$.

Suppose $v^\text{Cu}>v^\text{Al}$. The right hand side is negative, but since $F_m^\text{Cu}(v^\text{Cu}) > F_m^\text{Cu}(v^\text{Al}) > F_m^\text{Al}(v^\text{Al})$, the left hand side is positive so the above equation still isn't satisfied. The only remaining possibility is $v^\text{Cu}<v^\text{Al}$.

Answer 2

Let me see if I can explain this without equations. To simplify things a little let's consider identical magnets falling through identically sized pipes of aluminum and copper in a vacuum. The only force that is going to resist the falling of the magnet is going to be the force from the induced eddy currents.

The internal resistance in the aluminum is greater than that in the copper. This means that the eddy currents from the falling magnet are going to be weaker in the aluminum than in the copper at the same velocity.

If we look at the terminal velocity conditions for both cases the forces acting on both magnets are going to be the same since the force from gravity and the resistance force from the induced eddy currents will be equal. In the aluminum tube the velocity needed to create the equivalent resistance force is going to be higher since the resistance in the material is higher than that of copper.