$\newcommand{\bl}[1]{\boldsymbol{#1}}
\newcommand{\e}{\bl=}
\newcommand{\p}{\bl+}
\newcommand{\m}{\bl-}
\newcommand{\mb}[1]{\mathbf {#1}}
\newcommand{\mc}[1]{\mathcal {#1}}
\newcommand{\mr}[1]{\mathrm {#1}}
\newcommand{\gr}{\bl>}
\newcommand{\les}{\bl<}
\newcommand{\greq}{\bl\ge}
\newcommand{\leseq}{\bl\le}
\newcommand{\plr}[1]{\left(#1\right)}
\newcommand{\blr}[1]{\left[#1\right]}
\newcommand{\vlr}[1]{\left\vert#1\right\vert}
\newcommand{\Vlr}[1]{\left\Vert#1\right\Vert}
\newcommand{\lara}[1]{\left\langle#1\right\rangle}
\newcommand{\lav}[1]{\left\langle#1\right|}
\newcommand{\vra}[1]{\left|#1\right\rangle}
\newcommand{\lavra}[2]{\left\langle#1\right|\left#2\right\rangle}
\newcommand{\lavvra}[3]{\left\langle#1\right|#2\left|#3\right\rangle}
\newcommand{\vp}{\vphantom{\dfrac{a}{b}}}
\newcommand{\Vp}[1]{\vphantom{#1}}
\newcommand{\hp}[1]{\hphantom{#1}}
\newcommand{\x}{\bl\times}
\newcommand{\ox}{\bl\otimes}
\newcommand{\ol}[1]{\overline{#1}}
\newcommand{\qqlraqq}{\qquad\bl{-\!\!\!-\!\!\!-\!\!\!\longrightarrow}\qquad}
\newcommand{\qqLraqq}{\qquad\boldsymbol{\e\!\e\!\e\!\e\!\Longrightarrow}\qquad}
\newcommand{\tl}[1]{\tag{#1}\label{#1}}
\newcommand{\hebl}{$\bl{=\!=\!=\!==\!=\!=\!==\!=\!=\!==\!=\!=\!==\!=\!=\!==\!=\!=\!==\!=\!=\!==\!=\!=\!==\!=\!=\!==\!=\!=\!==\!=\!=\!==\!=\!=\!=}$}$
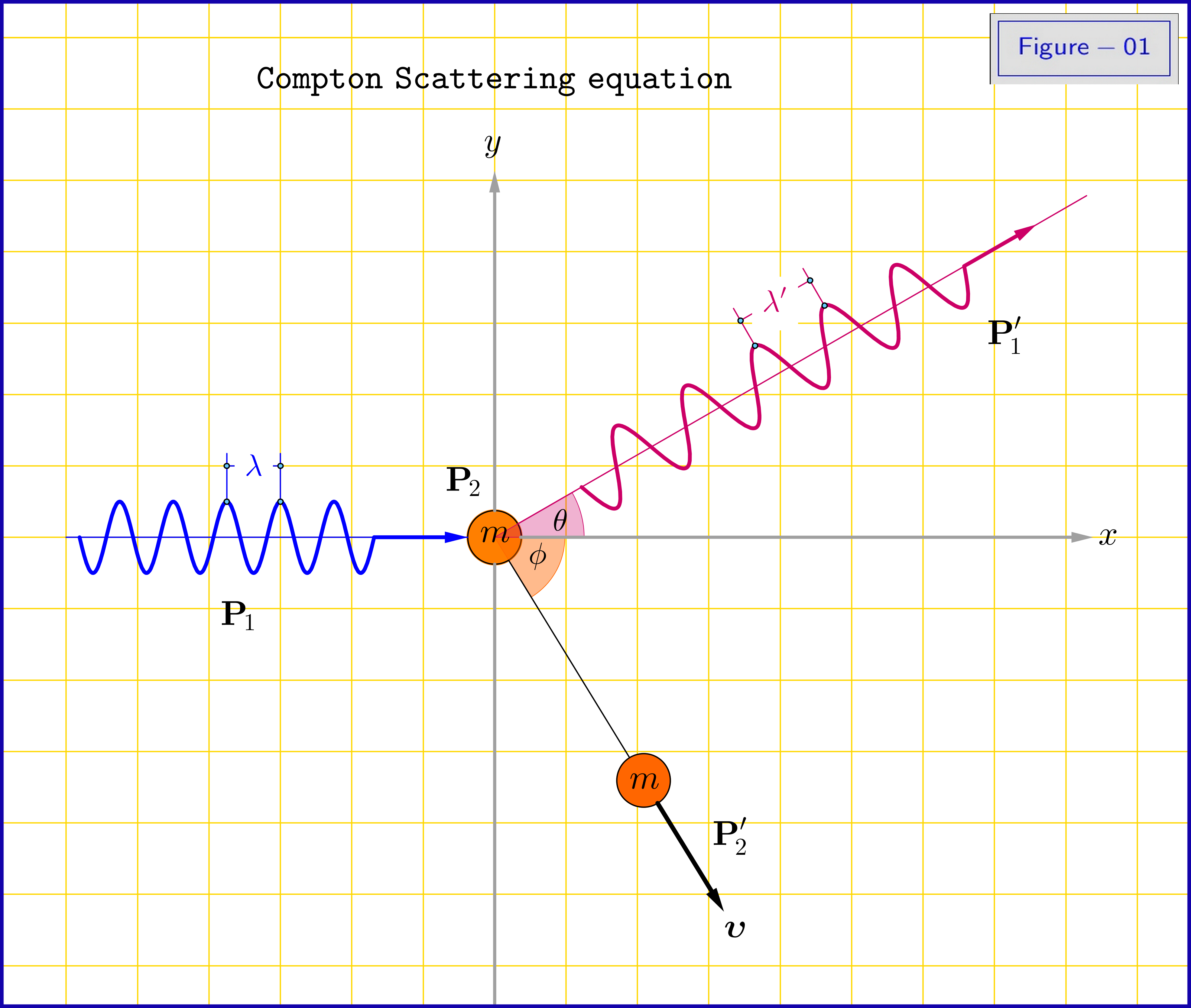
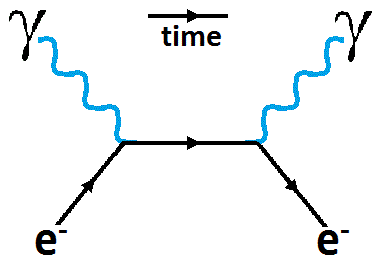
Compton scattering $\:\gamma\p A\bl\longrightarrow\gamma\p A\:$ occurs between a photon and a massive particle at least, it's not necessary for the particle to be electrically charge.
Note that the Compton scattering equation for the wavelength of a photon that scatters off a particle, initially at rest, of mass $\:m\:$ as shown in Figure-01 is
\begin{equation}
\boxed{\:\:
\lambda'\e \lambda\p\lambda_c\plr{1\m\cos\theta}\,,\qquad \lambda_c\e\dfrac{h}{mc}\e\texttt{Compton wavelength}\:\:\vp}
\tl{01}
\end{equation}