Supose two ideal magnets, close to each other and both at rest, with a north and a south poles both fixed and well defined. May we admit as well that the total electric charge of both is zero in the beginning. How can one explain the magnetic attraction between them having only the Maxwell's laws?
-
$\begingroup$ What is an “ideal magnet”? $\endgroup$– G. SmithCommented Feb 24, 2021 at 20:22
-
$\begingroup$ @G.Smith I'm not sure if the term exists, I meant like a continuous magnet with homogenuous "magnetic charge distribution" if this makes sense somehow $\endgroup$– hellofriendsCommented Feb 24, 2021 at 20:24
-
$\begingroup$ @G.Smith but...the attraction of positive and negative electrial charges can be derived from Gauss' law? $\endgroup$– hellofriendsCommented Feb 24, 2021 at 20:25
-
$\begingroup$ en.wikipedia.org/wiki/… $\endgroup$– G. SmithCommented Feb 24, 2021 at 20:29
3 Answers
No. The Maxwell equations describe how the electric and magnetic fields evolve given the charge density $\rho$ and current density $\mathbf J$. They do not describe the forces exerted on charged or magnetic material.
For that, you need an additional input like the Lorentz force model for charged point particles, or a model for the force on a magnetic dipole.
-
$\begingroup$ Lorentz force apparently can be derived from Maxwell's equations en.wikipedia.org/wiki/… $\endgroup$ Commented Feb 24, 2021 at 20:31
-
2$\begingroup$ @hellofriends No. That derivation takes as input that $\mathbf E = \mathbf F/q$ for a stationary charge. This is additional information above and beyond the Maxwell equations. $\endgroup$ Commented Feb 24, 2021 at 20:43
-
$\begingroup$ I think i finally got you. Even with Maxwell's description of the electric field these two laws are needed. Can we use them and the maxwell's equations to describe the attraction of magnets tho? $\endgroup$ Commented Feb 24, 2021 at 21:11
-
$\begingroup$ @hellofriends Well, you would need a model for what magnets are. You could imagine a permanent magnet as being made of little tiny current loops due to electrons whizzing around their nuclei (which is not a great model, but it's a start), in which case the Lorentz force model would give you the information that you need. $\endgroup$ Commented Feb 24, 2021 at 21:13
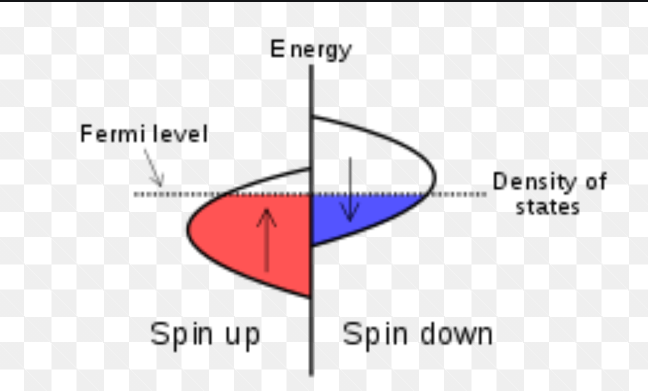
Ferromagnetism, which would be the physical state responsible for the magnetism of your magnets, cannot be understood with classical physics. It requires quantum mechanics to understand.
The reason is that the exchange interaction of quantum mechanics is responsible for ferromagnetism and this interaction cannot be explained without QM. In a magnet made of iron or nickel, the exchange interaction is responsible for splitting the energy d-band into a spin up and spin down bands. Normally, if you split the bands like this, they would have equal energies and placement relative to the Fermi Level. But below the Curie Temperature the exchange interaction lowers the energy spin up band and you have electrons from the spin down band move into the spin up band, thereby upsetting the equality of electron concentration between the two.
Here's an image to help visualize this:
-
$\begingroup$ so this phenomena would rearrenge the electrons producing an induced electric charge which would be the responsible for the attraction? $\endgroup$ Commented Feb 24, 2021 at 20:33
-
$\begingroup$ It would not be an induced electric charge. Since each electron acts like a tiny magnet with its own magnetic dipole moment (another thing that requires QM!), if you line up more of the electrons with spin in a certain direction, you will produce a global magnetic moment in the material in the same direction. $\endgroup$– CGSCommented Feb 24, 2021 at 20:37
-
$\begingroup$ fair enough, you kinda explained the origin of the magnetic field. But since the magnetic force (Lorentz) can't produce work this doesn't seem to be enough to discribe why would a magnet move towards the other $\endgroup$ Commented Feb 24, 2021 at 20:40
-
$\begingroup$ "Ferromagnetism [...] requires quantum mechanics to understand" OK, to keep focus assume electromagnets. $\endgroup$– my2ctsCommented Feb 24, 2021 at 20:52
-
$\begingroup$ @hellofriends Sorry, I should have read your question closer. Just as you cannot fundamentally explain why a proton attracts and electron (regardless of the exchange of virtual photons, which really does not get you closer to an answer), or why two masses attract one another, the attraction of the N and S poles of a magnetic (or the repulsion of like poles) is a physical phenomenon that has to be accepted as true. $\endgroup$– CGSCommented Feb 24, 2021 at 20:54
Consider the effect of a cylindrical magnet on a current loop, centered on and aligned along the z axis. Consider the z component of the magnetic field. It will cause a radially oriented force, perpendicular to z, on the loop. The loop will expand or shrink somewhat. There is no force component along z. For this to happen the magnet must produce a B field component perpendicular to z at the loop. This will attract or repel the loop towards or from the magnet. Since $$\mathbf \nabla \cdot \mathbf B = 0$$ such a component implies $$dB_z/dz \neq 0 ~ .$$ This makes the connection with the formula $$\mathbf F = \mathbf \nabla (\mathbf m \cdot \mathbf B) ~ .$$
However I have used the Lorentz force and this cannot be directly found from the Maxwell equations, let alone just from Faraday's law. Its derivation requires the extra physical assumption of the principle of least action. If we interpret the Maxwell equations as Euler-Lagrange equations applied to a suitable Lagrangian the energy-momentum tensor can be derived, the divergency of which contains the Lorentz force. This derivation relies on the, purely mathematical, Noether theorem.