Could an infinite number of photons fit into a finite space?

Question

As photons are quantum particles and basically waves in a quantum field, could an infinite number of photons exist in a closed space described by finite numbers? Does the answer to this apply to other fundamental particles as well?

Answer 1

In principle you could fit a very large number of photons into a finite volume but with a limit. Even though photons are waves, they have energy and from general relativity you can only have so much energy in a certain region up to a point where the energy density is so high that the region will collapse into a black hole. At this point the region will be infinitely dense and infinitely small.

So you probably could not fit an infinite number in a finite volume as the energy density will be infinite. This would also apply to fundamental particles as well (assuming they have no well defined volume) since they have mass and therefore energy.

Furthermore, if you were to continually put more photons/matter into it, the "stuff" inside the black hole (given a sufficient length of time) will gradually dissolve by radiating away the energy of the stuff that was there to begin with, once again meaning that no finite region can have infinite photons/particles.

To see more on this last part, click this link for more information about Hawking Radiation.

Answer 2

If the energy density becomes high enough electron-positron pairs will form. This limits the energy density of a photon gas. For example pairs can form in strong laser fields by multiphoton processes. It does not in principle require single photon energies of order $mc^2$. The so-called strong field Breit-Wheeler process happens of course at an energy scale much lower than that required for black hole formation. John Dvorak provided a Feynman diagram for such a multiphoton process. 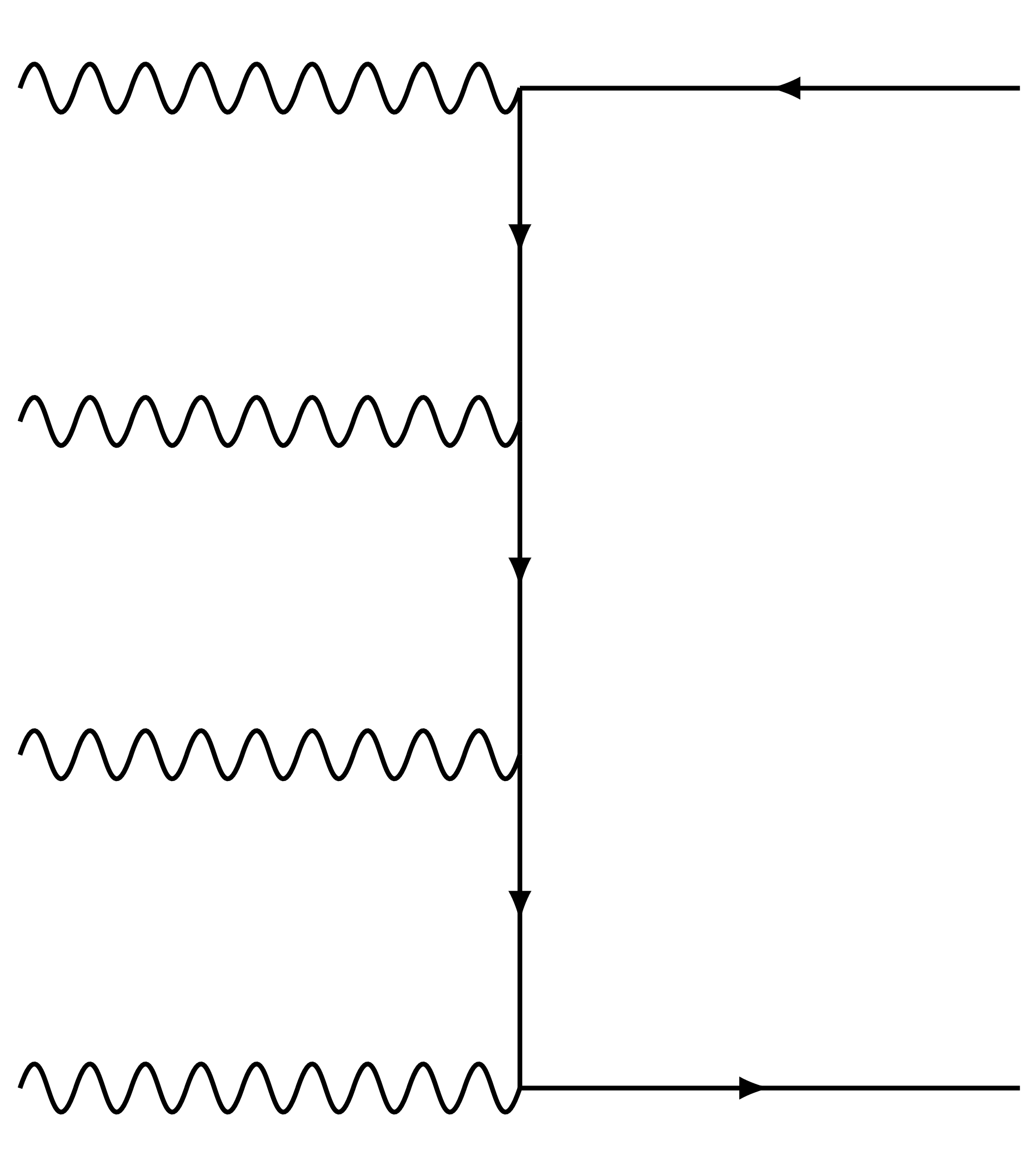Here four photons convert into a positron-electron pair, if the sum of their energies is large enough. If it is not then a process involving even more photons will be the leading vacuum instability.

https://www.researchgate.net/publication/225996098_Electron-positron_pair_creation_by_a_strong_tightly_focused_laser_field

https://www.sciencedirect.com/science/article/pii/S2468080X17300183

Answer 3

Let's use a spherical shell to confine the photons in a finite space. As a means to an end. The shell is made of incompressible material and reflects every photon (obeying Bose-Einstein statistics, so more photons can exist in the same state) inside elastically.
Let's fill the shell with photons. The photons must have a wavelength compatible with the radius of the shell. If the radius is very small (corresponding to a small volume of space) only high energy photons can enter, and if the shell has a larger volume also lower energy photons can enter (how we make them enter is of no importance; we can simply envision that the number of photons increases).
If the number of photons increases then, obviously, the energy contained in the shell increases ($E_{photon}=hf$). After enough photons have entered (with energies below the energy to create fermion-antifermion pairs, though they probably would recombine to recreate the two photons again; anyhow the energy would stay in the shell if we assume the box can't let the electron and anti-electron tunnel through) the huge accumulated energy of the photons (bosons) makes them collapse into a black hole.

There is no counter"force" which is present if we would put fermions in the box. This last phenomenon (degeneracy pressure, due to Fermi-Dirac statistics, isn't present in the case of photons) is what prevents neutron stars and white dwarfs to collapse into a black hole.
So, to summarize, a BH will form if you put enough photons in a volume of space (I'm not sure if the emerging Schwarzschild radius is the same as the radius of the shell). The size of the volume can have any finite size.

If instead, you put massive fermions into the shell, a BH will be formed too. But before that happens a neutron star and white dwarf will be formed due to the degeneracy pressure (if the volume of space in the shell is large enough). The degenerate pressure (as said, due to Fermi-Dirac statistics) of both will be overcome if more mass (fermions) is added after which gravity "wins" (It is thought that beyond 2.16 M☉ the stellar remnant will overcome the strong force repulsion and neutron degeneracy pressure so that gravitational collapse will occur to produce a black hole, from the Wikipedia article on neutron stars).

Answer 4

As you can see from the correct answer from Dr jh, if you try to somehow limit photons into a small region of space, energy density will rise and after a certain point, the ensemble of photons will collapse into a black hole, forming an event horizon. Photons are bosons, and theoretically any number of photons could occupy the same space.

I do feel though, that certain things need clarification:

1. at low energy levels, any number of photons (being bosons) could theoretically occupy the same volume of space

2. at high energy levels, you enter non-linear optics, and photon-photon interactions do happen

https://en.wikipedia.org/wiki/Nonlinear_optics

3. at high energy levels, photons' own gravitational effects (their own static gravitational field) becomes considerable, possibly leading to the formation of an event horizon

Do photons bend spacetime or not?

4. in our universe, space is expanding at an accelerated rate, and contrary to popular belief, space is expanding everywhere. The reason we do not experience it locally, is the dominance of gravity, the strong and the EM force over the expansion. In your example, expansion could affect photons in two ways. On the one hand, photons could get farther apart, and on the other hand, photons' wavelength could be stretched (lowering their energy level) in expanding space. Please note that this is one of the reasons we say that energy is not conserved at cosmological scales.

The de Broglie wavelength is redshifted by the expansion of space in the same way that the wavelength of light is redshifted.

Please note that at high energy levels there could be something called a BEC of photons (or geon) or at the energy levels required for the formation of an event horizon, there could form something called a Kugelblitz.

It can, it's just hard as you have to engineer it.

Why can't a gas of photons reach a Bose-Einstein condensate?

https://en.wikipedia.org/wiki/Kugelblitz_(astrophysics)

Kugelblitz is defined as a concentration of photons (like in your example), so that it's stress-energy forms an event horizon.

Answer 5

No. Because we are in a finite space, it gives an upper limit to the wavelength of the photons. Having an upper limit to the wavelength, we also have a lower limit to their energy, thus to their mass.

Having infinite particle with a lower mass bound, would mean that we have infinite mass in a finite volume. This is impossible. Q.E.D.