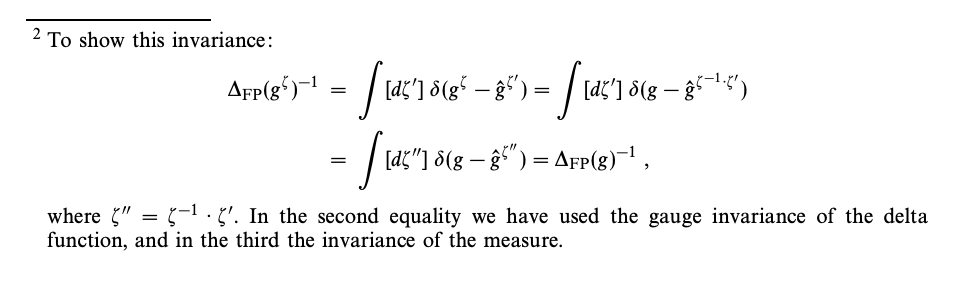Gauge invariance of Faddeev-Popov determinant in bosonic string theory

Question

I am, once again, going through an introduction to (bosonic) string theory, following the lecture notes by David Tong on the subject, and once again I am stumbling on technicalities around the Polyakov path integral formulation.

This time it is the claimed gauge invariance of the Faddeev-Popov determinant, defined in Tongs notes in eq. (5.1) on page 110 as:

$$\Delta[g]^{-1}=\int_G\mathcal{D}\xi\delta(g-g_0^\xi)\tag{5.1}$$

where, for simplification, $g$ and $g_0$ are lorentzian metrics on the zylinder and the integral is over "the Haar measure" on the group $G$ of diffeomorphisms and Weyl transformations. For $\xi$ the diffeomorphism $f$ and Weyl factor $\phi$, $g^\xi=\phi f^*g$ or something along those lines.

Tong claims that this expression is gauge invariant, that is $\forall \epsilon\in G$: $\Delta[g^\epsilon]=\Delta[g]$, and gives a short uncommented proof of it as:

$$\Delta[g^\epsilon]^{-1}=\int_G\mathcal{D}\xi\delta(g^\epsilon-g_0^\xi)=\int_G\mathcal{D}\xi\delta(g-g_0^{\epsilon^{-1}\xi})=\int_G\mathcal{D}\xi\delta(g-g_0^{\xi})=\Delta[g]^{-1}.\tag{p.111}$$

I guess the third equality uses the translation invariance of the Haar measure, but the second step simply seems wrong to me. I think it should be:

$$\int_G\mathcal{D}\xi\delta(g^\epsilon-g_0^\xi)=\int_G\mathcal{D}\xi\delta(g^\epsilon-g_0^{\epsilon\xi})=\int_G\mathcal{D}\xi\delta([g-g_0^\xi]^\epsilon)=\int_G\mathcal{D}\xi\frac{\delta(g-g_0^\xi)}{|\det\frac{\delta h^\epsilon}{\delta h}\vert_{h=0}|}.$$

If we were talking about a representation of a compact topological group it is clear that this determinant is $1$, but in this case I can't see it.

Moreover, there is indirect evidence that the Fadeev-Popov determinant is not gauge invariant: Apparently it can be written as the partition function of a $c=-26$ CFT, but the partition functions of CFT's are only Weyl-invariant for $c=0$ (or flat background metric which we can't assume since we are integrating over all background metrics).

The question is: am I overlooking something, and if yes, what? To be clear, I am convinced that treating this un-invariance correctly gives the right expression for the gauge fixed pathintegral anyway, but the presentation in Tongs notes seems flawed, even apart from all the assumptions made.

Remark: this would also clear up an earlier question of mine, since the un-invariance of the Faddeev-Popov determinant and that of the string measure would exactly cancel in $26$ dimensions, see my earlier question.

Answer 1

Let $Z[g]$ be the partition function of a conformal field theory with central charge $c$ on a genus $0$ surface, $F[g]=\ln Z[g]$ the "free energy". It is a standard result that \begin{equation} g^{ab}(p)\frac{\delta}{\delta g^{ab}(p)}F[g]\sim c\sqrt{|g|}R[g](p)\qquad(1) \end{equation} where $R[g]$ is the Ricci curvature and the proportionality constant is not zero and independent of $g$. In particular, eq. (1) implies that the partition function can't be Weyl rescaling invariant whenever $c\neq 0$ and the background is curved.

Firstly, the proof of gauge invariance given by Tong and Polchinski is, almost literally cited, this: \begin{equation} \Delta[g^\epsilon]^{-1}=\int\mathcal{D}\xi\delta(g^\epsilon-g_0^\xi)=\int\mathcal{D}\xi\delta([g-g_0^{\epsilon^{-1}\xi}]^\epsilon)=\int\mathcal{D}\xi'\delta([g-g_0^{\xi'}]^\epsilon)=\int\mathcal{D}\xi'\delta(g-g_0^{\xi'})=\Delta[g]^{-1}\qquad(2) \end{equation}

The point where i don't agree is the second to last equality in eq. (2): as is well known there should be a factor of $|\det({\frac{\delta h^\epsilon}{\delta h}\vert_{h=0}})|^{-1}$ appearing. If we were talking about a representation of a compact group I would agree that this is always $1$, but, since we are including Weyl rescalings, the group we are considering is far from compact. In particular consider the case when $\epsilon$ is a Weyl rescaling $h^\epsilon=\phi h$, then we have to determine $\det('\text{multiplication with }\phi')$, which I highly suspect to not be $1$ for general $\phi$ (even when regularized appropriately).

Secondly, assume that we are on a cylinder such that $\exists \epsilon:g=g_0^\epsilon$. Then following Tong almost word by word we find that \begin{align*} \Delta[g]^{-1}&=\int\mathcal{D}\xi\delta(g_0^\epsilon-g_0^\xi)=\int\mathcal{D}\xi\delta(g_0^\epsilon-(g_0^\epsilon)\xi)\\ &=\int\mathcal{D}\xi\delta(2w(g_0^\epsilon)_{ab}+\nabla_{(a}\nu_{b)})=\ldots\\ &=Z_{\text{bosonic ghosts}}[g_0^\epsilon] \end{align*} so that at the end of the day we can write the Fadeev-Popov determinant as the partition function of the ghost CFT: \begin{equation} \Delta[g]=Z_{\text{gh}}[g]\qquad(3) \end{equation} where the right hand side, as discussed above, is not gauge invariant: Let $\epsilon_\phi$ be the Weyl rescaling by $1+\phi$, gauge invariance must imply that $\frac{\delta \Delta[g^{\epsilon_\phi}]}{\delta \phi(p)}\vert_{\phi=0}=0$, but according to eq. (1) and (3) we have \begin{align*} \frac{\delta \Delta[g^{\epsilon_\phi}]}{\delta \phi(p)}\vert_{\phi=0}&=\frac{\delta Z_{\text{gh}}[g^{\epsilon_\phi}]}{\delta \phi(p)}\vert_{\phi=0}=\frac{\delta Z_{\text{gh}}[g+\phi g]}{\delta \phi(p)}\vert_{\phi=0}\\ &=\int\mathrm{d}q\,\frac{\delta Z_{\text{gh}}[g]}{\delta g^{ab}(q)}\frac{\delta \phi(q) g^{ab}(q)}{\delta \phi (p)}\vert_{\phi=0}=\int\mathrm{d} q\,\frac{\delta Z_{\text{gh}}[g]}{\delta g^{ab}(q)}g^{ab}(q)\delta(p-q)\\ &=Z_{\text{gh}}[g]g^{ab}(p)\frac{\delta}{\delta g^{ab}(p)}F_{\text{gh}}[g]\sim \Delta[g]c\sqrt{|g|}R[g](p) \end{align*}

So, since the ghost CFT in this case has $c=-26\neq0$ and $g$ in general might have non zero curvature we find that the Fadeev-Popov determinant can't be gauge invariant.

\newpage Finally, I want to remark that this is actually not a problem for our considerations, but makes it possible in the first place: \begin{align*} Z_{\text{String}}&=\int\mathcal{D}gZ_{\text{Polyakov}}[g]=\int\mathcal{D}g\Delta[g]\int\mathcal{D}\xi\delta(g-g_0^\xi)Z_{\text{Polyakov}}[g]\\ &=\int\mathcal{D}\xi Z_{\text{gh}}[g_0^\xi]Z_{\text{Polyakov}}[g_0^\xi] \end{align*}

The combination $Z_{\text{gh}}[g_0^\xi]Z_{\text{Polyakov}}[g_0^\xi]$ has a conformal anomaly given by $c=D-26$, so it is gauge invariant if and only if $D=26$! In that case we can drop the integration over the gauge group and the associated infinite but constant factor to get \begin{equation*} Z_{\text{String}}=Z_{\text{gh}}[g_0]Z_{\text{Polyakov}}[g_0] \end{equation*} which is our desired result.

Answer 2

I think it might be useful to look in Polchinski: in a footnote on page 87 of Vol. 1 he addresses the gauge invariance of the FP determinant. I've attached a screenshot here for convenience:

Essentially Polchinski claims the second line of what you've shown is given by gauge invariance of the delta functional. He doesn't actually prove that statement so you'd have to take his word for it but hopefully that helps!

Answer 3

This doesn't answer the question, but kind of solves the problem. There is a slightly different definition of the Fadeev-Popov determinant that doesn't require to show that the $\delta$ function is invariant but gets the job equally done. Namely we apply the gauge $\xi$ on the metric integrated over and not on the fiducial metric. $$\Delta[g]^{-1}=\int_G\mathcal{D}\xi\,\delta(g^\xi-g_0)\,.$$ This works because $g^\xi = g_0$ has the same solutions as $g = g_0^{\xi^{-1}}$, so since you integrate over all $\xi$, the $\delta$ function will hit the same zeros.

This definition solves the issue because, assuming that the Haar measure is invariant, one has

$$ \begin{aligned} \Delta[g^\zeta]^{-1}&=\int_G\mathcal{D}\xi\,\delta(g^{\zeta\,\xi}-g_0) \\&= \int_G\mathcal{D}(\zeta^{-1}\xi')\,\delta(g^{\zeta\,\zeta^{-1}\xi'}-g_0) \\ &=\int_G\mathcal{D}\xi'\,\delta(g^{\xi'}-g_0) = \Delta[g]^{-1}\,. \end{aligned} $$

As for the second part: true, the partition function is not well defined if $c$ is non zero. But the reason for that is not an issue of the FP determinant (not its Haar measure, nor the $\delta$ function). It's an issue of the field measure that appears in the rest of the path integral, namely the $\mathcal{D}X^\mu\mathcal{D}g$. When $c\neq 0$ you have an anomaly and that is known to lead to a non-invariance of the field measure under gauge transformations.