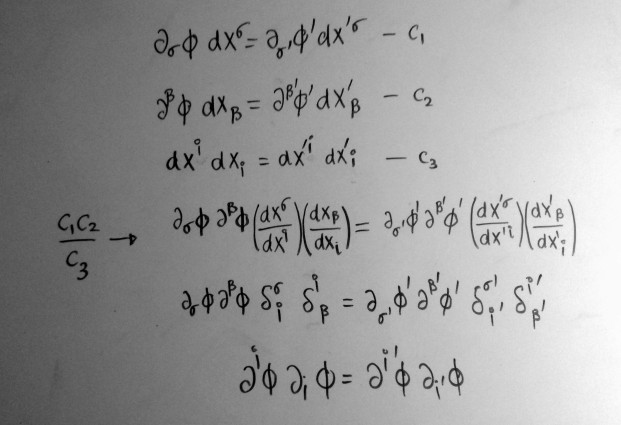Is this a right approach to show that $\partial_{\mu} \phi \partial^{\mu} \phi $ is Lorentz Invariant?

Question

When trying to convince myself that $\partial_{\mu} \phi \partial^{\mu} \phi $ is Lorentz Invariant, I stumbled upon this approach:

The last equation should read - $\partial_{i} \phi \partial^{i} \phi = \partial_{i^{'}} \phi^{'} \partial^{i^{'}} \phi^{'} $

Here since $C_1$, $C_2$ and $C_3$ are just scalars, it permits us do something like $\frac{C_1 C_2}{C_3}$. And this shows that $\partial_{\mu} \phi \partial^{\mu} \phi $ is Lorentz Invariant.

Does this seem logical to do this and prove it this way? I understand that there exist other better methods to show the same, I am just wondering if this method is consistent.

** $dx$ and $dx'$ are related by the Lorentz Transform.

Answer 1

It's not really consistent. You're manipulating symbols in a way that doesn't make sense, especially when you bring together $c_1$, $c_2$ and $c_3$ in $c_1 c_2/c_3$. Just start by showing how $$\frac{\partial}{\partial x^\mu} \phi$$ transforms under a Lorentz transformation $x \to x' = \Lambda \cdot x$. Finally use a key fact you know about the matrix $\Lambda$, namely $\Lambda^T \cdot \eta \cdot \Lambda = \eta$.