A tricky question. Here is the famous graph of the running of the three coupling constants in the standard model: http://www-ekp.physik.uni-karlsruhe.de/~deboer/html/Forschung/unification_eng.eps .
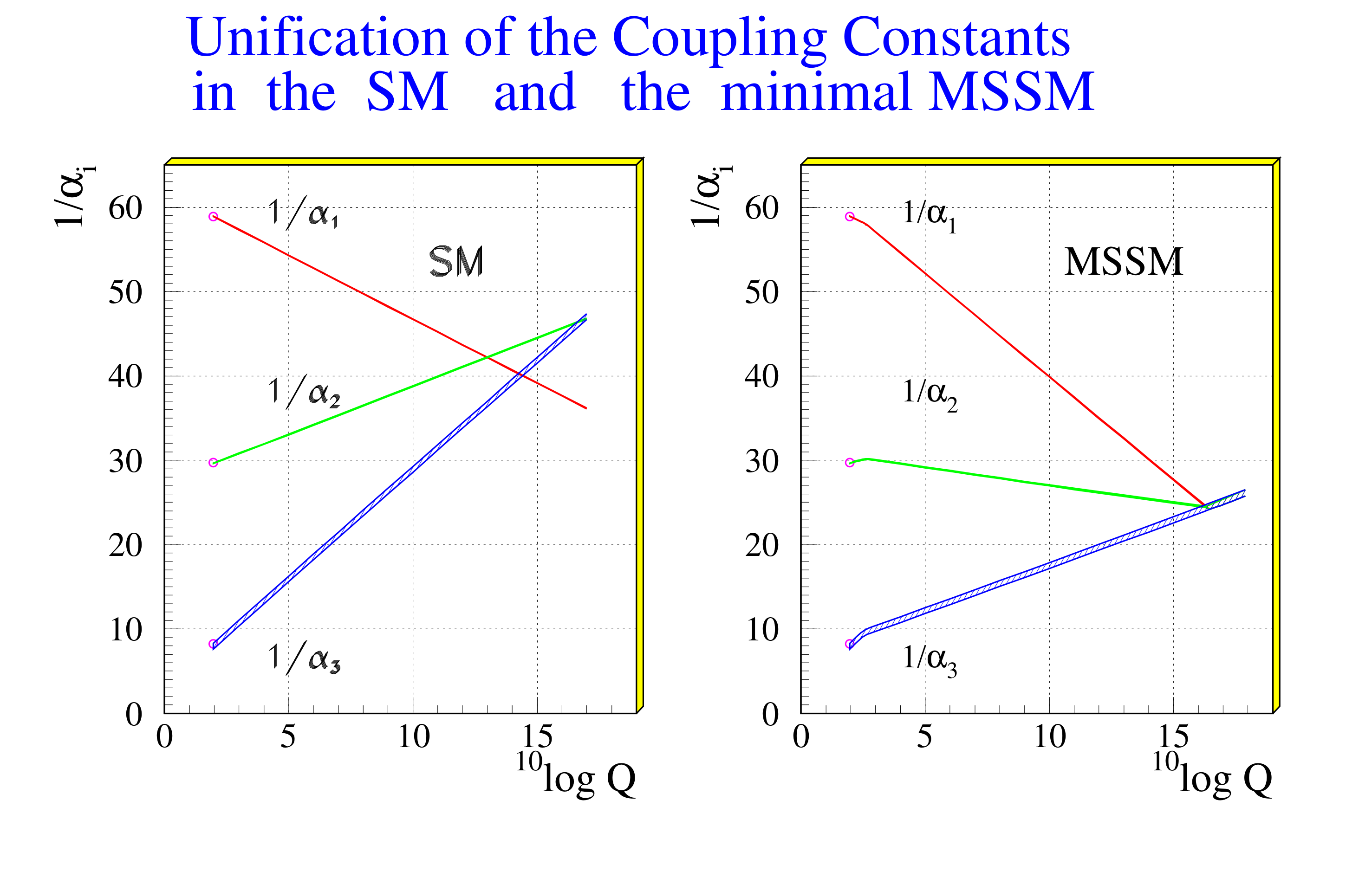
The graph shows, in its top curve, the running of the coupling constant alpha_1$\alpha_1$. This is the coupling of the weak hypercharge coupling constant for the weak hypercharge group U(1)_Y$\mathrm{U}(1)_{Y}$, which is one of the three gauge groups of the standard modelStandard Model of particle physics.
But there is a tricky detail. In that curve, alpha_1$\alpha_1$ is multiplied with 5/3$5/3$. This factor 5/3$5/3$ comes from the assumption that GUTs are valid. The factor ensures that the various group traces of U(1)_Y$\mathrm{U}(1)_Y$, SU(2)$\mathrm{SU}(2)$ and SU(3)$\mathrm{SU}(3)$ are normalized in the correct way when they form the SU(5)$\mathrm{SU}(5)$, SO(10)$\mathrm{SO}(10)$ or any other grand unification gauge group.
In the case that grand unification is wrong, the factor 5/3$5/3$ cannot be deduced. Which factor would be natural in this case?
Clarification added, after remarks by Lubos Motl: it is assumed in the question that the usual definition of the weak hypercharge is used, Y_W=2(Q-T_3)$Y_W = 2 (Q - T_3)$, in which left-handed quarks have hypercharge 1/3$1/3$.
