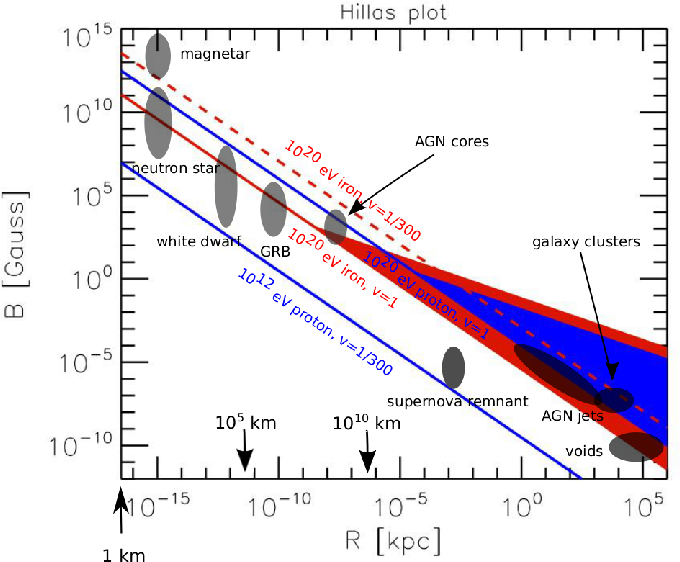
We can make a reasonable guess by taking a look at the Hillas criterion. The gist of this criterion is that the maximum energy of the particle, $E$, is limited by the size of the accelerator, $R$ ($\sim R_L$ the Larmor radius), and the strength of the magnetic field, $B$. The relation gives $$ E_{max}=10^{18}\,Z\frac vcB_{\mu G}R_{kpc}\,{\rm eV} $$$$ E_{max}=10^{18}\,Z\beta B_{\mu G}R_{kpc}\,{\rm eV} $$ where $B_{\mu G}$ is the magnetic field measured in micro-Gauss, $R$ measured in kiloparsecs, $v$$\beta=v/c$ the velocity of the particleaccelerator site (i.e., the shock wave), and $Z$ its atomic number. This relation is best understood by looking at it:
The blue lines represent the maximum energy of a proton within different velocitiesaccelerator sites ($v=c/300$ and$\beta=1/300$ is representative of a non-relativistic shock $v=c$(e.g. supernova remnant shock) whereas $\beta=1$ is representative of the relativistic shocks (e.g. AGN jets)), the readred for iron nuclei. The gray blobs in the plot are characteristic of the astronomical objects magnetic field sizes and radii. Any class of objects above the diagonal line will be able to accelerate the proton/iron nuclei to the marked energies.
Clearly, from the chart, it requires a huge magnetic field ($10^{15}$ Gauss) to accelerate iron to $10^{20}$ eV, hence the magnetar being the only candidate. As for protons, active galactic nuclei (AGN) and their jets are able to accelerate a proton to $10^{20}$ eV with seeming ease, same with galaxy clusters.
So from a heuristic stand-point, the idea that our UHECR's being heavy nuclei appears to be ruled out (note that this criterion has not completely eliminated it or is proof that it's not heavy nuclei, just that it's not likely; and as dmckee says, we get so few at that energy it's just not worth the money to put the equipment to discern it).

 (
(