The general energy balance equation for open systems is as follows:
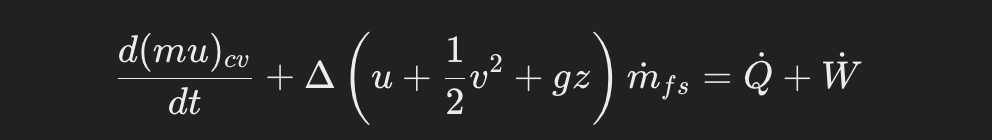
$$\frac{\mathrm{d}(mu)_{cv}}{\mathrm{d}t} + \Delta \left(u + \frac{1}{2} v^2 + gz\right)\dot{m}_{fs} = \dot Q + \dot W,$$
Andand the Reynold's Transport Theorem applied on energy is as follows:
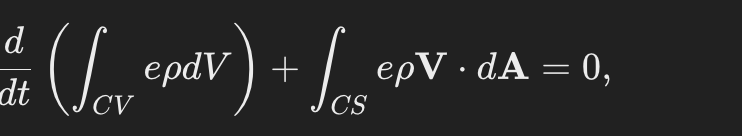
$$\frac{\mathrm{d}}{\mathrm{d}t}\left(\int_{CV} e \rho \ \mathrm{d}V\right) + \int_{CS} e \rho \mathbf{V} \cdot \mathrm{d}\mathbf{A} = 0.$$
But, if you put e = u + 1/2v^{2} +gz$e = u + \frac{1}{2}v^{2} +gz$ into the RTTReynold's Transport Theorem equation, you would get d/dt(m*(u + 1/2v^{2} +gz)
$$\frac{\mathrm{d}}{\mathrm{d}t}\left[m \cdot \left(u + \frac{1}{2}v^{2} +gz \right)\right]$$ for the control volume term as well.
I don't understand how to mathematically go from the RTTReynold's Transport Theorem equation to the General Energy Balance Equationequation. What assumptions do we take and where am I misunderstanding (if I am?)
Please pardon the poor formatting, I'm new here.?
