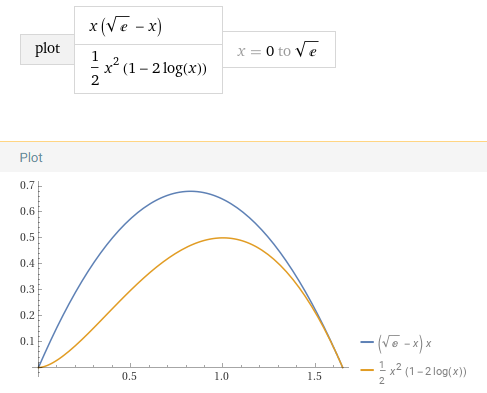
Let think I am studying some simple system (this is a thought experiment), where I have two classic objects, and the position versus time plots follows two curves (I will left out physics constants, to keep focus in the math I am having trouble to understand): one parabolic path as a reference given by: $$g(t) = t\,(\sqrt{e}-t)$$ and another path that is less steep, grows slowly at the beginning and falls later like the parabolic path, and I believe is always below the parabolic example, given this behavior by: $$f(t) =\begin{cases}0,\quad t=0 \\ \dfrac{t^2}{2}\left(1-2\ln(t)\right),\, t>0\end{cases}$$

At first, as you could see in the plot both looks quite "innocent":

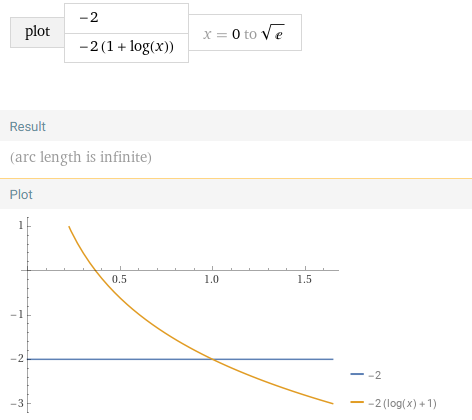
But if I go to their speed profiles, then something weird could be noticed: while $g'(x) = \sqrt(e)-2t$ so is always linearly losing speed, but instead for the other curve: $$f'(t) = -t\,\ln(t^2)$$ which is also losing speed, but at the beginning, instead of showing a less steep start, it has an INFINITE SPEED!!!, so it doesn't matter how many constants I am avoiding, there will be always an interval near $t=0$ when it is starting to move faster the $c$ the speed of light!!

Here the speed profile:

Here the acceleration profile, showing $f(t)$ diverges at $t=0$:

I think I have some conceptual misunderstanding here (so I hope you could explain what I am understanding wrong - but if the maths' problem still holds when corrected, please focus in explain it also), but this is kind of saying that a smooth start from rest position is impossible, which I really doubt, maybe I am mistaken, but daily experience intuition tells that not everything start moving violently, like when I slightly blow over a ping-pong ball.

Or maybe just the behavior of $f(t)$ is aphysical, in this case hope you explain why with detail (but hopefully, in simple terms). I think is quite counter-intuitive that a smoothest start leads to unachievable speeds, I quite lost here.