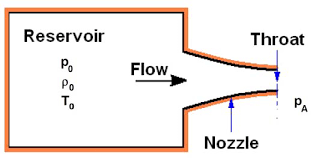
When a compressible (say, ideal gas) fluid and an incompressible fluid (when flowing, the divergence of velocity is zero) flow through a adiabatic converging nozzle, will the exit velocity of the compressible fluid be higher than that of the incompressible fluid?. Let's assume the same initial conditions for both the fluids and the fluid acceleration take place across the same pressure difference. Let's also assume that the pressure ratio is less than the critical pressure ratio for compressible flow (so no sonic flow) and that the flow is isentropic.
It's my understanding that for incompressible flow the Kinetic Energy (KE) increases (per unit mass) due to $dh = -VdP$ work, where the V is a constant and there is no change in internal energy, and no PdV work. For compressible flow, there is expansion of the gas as it flows through the nozzle, so there is PdV work by the gas is equal to the change in internal energy dU, ie $dU = -PdV$. Again, the change in the KE is
$dh = dU + PdV +VdP = VdP$ as dU = - PdV but
but here V is not a constant but is given as a function of pressure ie $V(P)$. and there is a change in internal energy (temperature) of the fluid from inlet to exit of the nozzle
Is this correct?