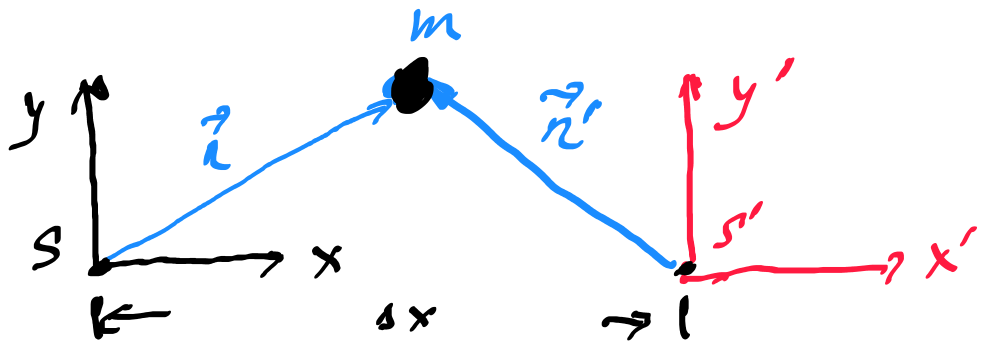
Lets look at this example
The particle energy in S frame is
$$E_s=\frac 12 m \mathbf v_s\cdot \mathbf v_s+m\,g\,y=\text{const.}$$
where $~\mathbf v_s=\frac{d}{dt}\mathbf R~$
The particle energy in S' frame is
$$E_s'=\frac 12 m \mathbf v_s'\cdot \mathbf v_s'+m\,g\,y'$$
where
$$\mathbf v_s'=\frac{d}{dt}\begin{bmatrix} R'_x+\Delta x \\ R'_y\\ \end{bmatrix}$$ thus, the particle energy $~E_s'~$ is conserved (constant) only if $~\Delta x=v_x\,t~$ or $~\Delta x=~$ constant, where $~v_x~$ constant, in this case is also the energy different (relative$~\Delta E=E_s'-E_s~$ relative energy) conserved.
is the energy invariant ? invariant mean that $~E_s=E_s'~$ this is not the case.
but if $~\Delta x~=$ const. and $~\mathbf R=\mathbf R'~$ then the energy $~E_s=E_s'~$ (invariant)
Fazit
the "frames energies" can be
- conserved and invariant
- only conserved
- only invariant
- neither conserved neither invariant