Given 6 points that are connected with each other with a resistor of resistance $R$, find the resistance between any two points. (Answer: $R/3$)
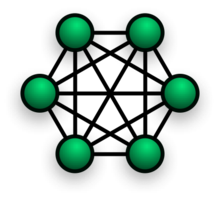
(allAll the conducting wires have the same resistance $R$.)
So I know that such a wording immediately implies that these 6 points are absolutely identical, which makes it possible to apply symmetry arguments that will help in reducing the network to a more simplesimpler one.
That is, after choosing any two points in the network, we can remove the resistors which are connected between the otherremaining four points, as they remained will still be identical, and they keep the symmetry, thusso we can change betweenswap any of them and the network will remain the same. Thus, we can remove the resistors which are connected between these other four points, since the points are identical.
However, we can alsoalso swap the two chosen points, and the system will still remain the same. So why can't we also remove the resistor between the two chosen points?
I'm told of the following analogy: theThe system of these 6 points is like a system of 6 absolutely similar balls painted, say, in white. By choosing two points, we paint them in black, thus the system loosesloses some symmetry level, but its certain elements are still symmetrical under certain dispositionsrearrangements. But
Specifically, any two of the white balls can be swapped without changing the system in any way, so all the white balls are identical and we can ignore any resistors between them. But swapping the two blackblack balls still won't change the system, so why can't we follow the same logic and removeignore the resistor between the two chosen pointsthem as well?
I'll generalize the question a little bit: - why we don't care of other symmetries in the system?why don't we care about other symmetries in the system?
(I'm looking forward a simple explanation, without involving advanced math, as I'm just a self-taught beginner and meanwhile II'm only familiar with calculus. So I try to avoid matrices and anything advanced which students learn in advanced courses of electronics. I just want to get the idea and the concept itself).)
