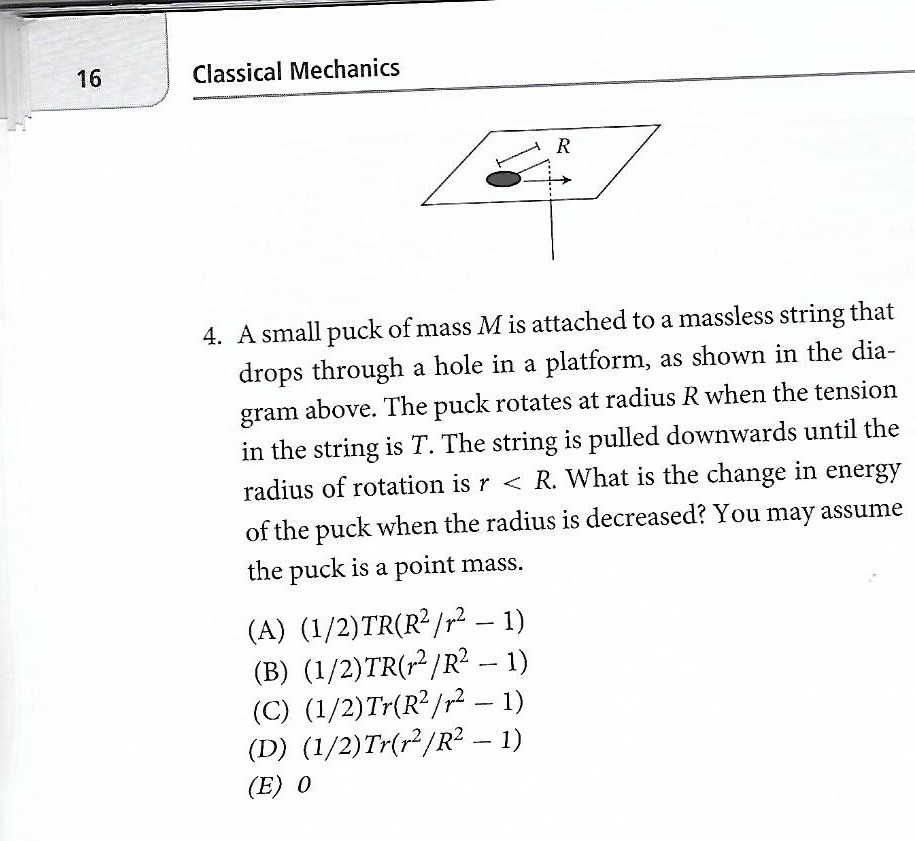
I was studying for the GRE and came across the problem below.
A small puck of mass M$M$ is attached to a massless string that drops through a hole in a platform, as shown in the diagram above. The puck rotates at radius R$R$ when the tension in the string is T$T$. The string is pulled downwards until the radius of rotation is r<R$r<R$. What is the change in energy of the puck when the radius is decreased? You may assume the puck is a point mass.
The book proceeds to solve this problem by calculating the velocity at R$R$ using the given central force and then calculating the velocity at r using conservation of angular momentum before finally calculating the change in energy as the difference in kinetic energy (the answer is A). This is all fairly straightforward. However, isn't there usually also a reduction in potential energy associated with a reduced radius of orbit? I'm not sure how one would go about calculating the potential energy associated with a force like this. I'm not sure if it is even a conservative force. If not, is there a potential energy associated with nonconservativenon-conservative forces?