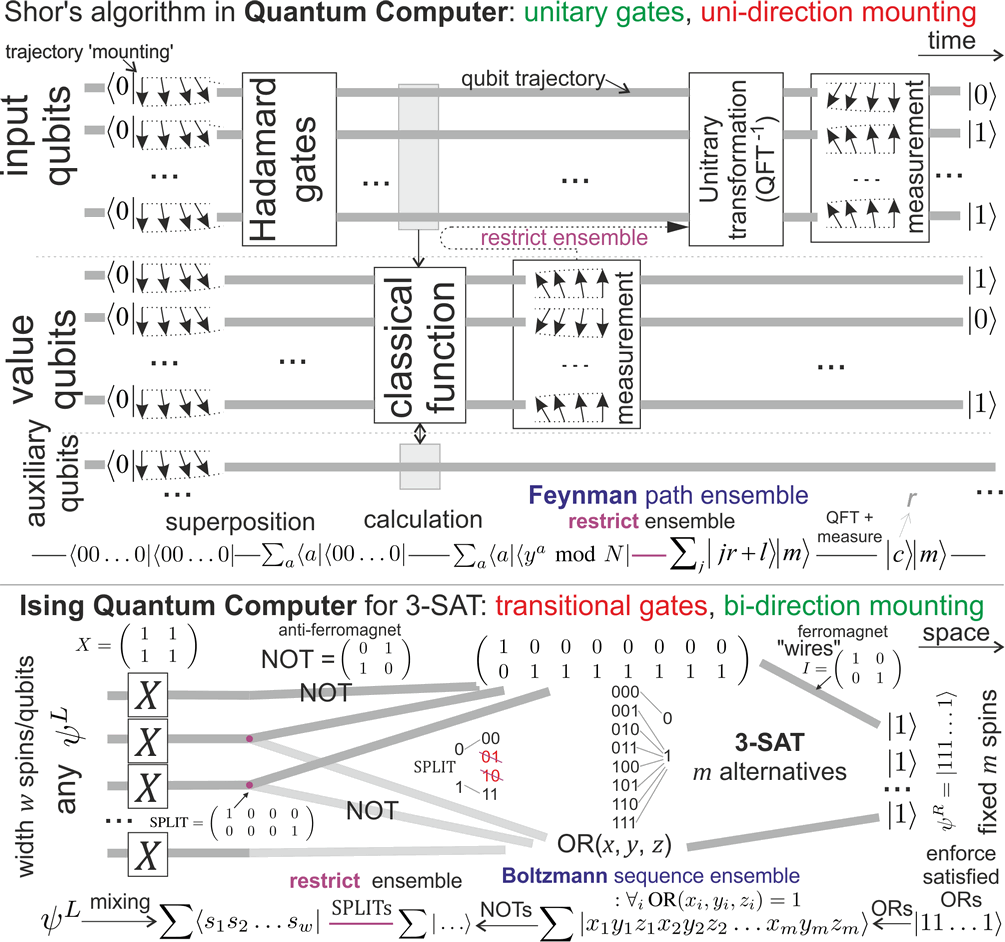
Quantum mechanics is equivalent with Feynman path ensemble, which after Wick rotation becomes Boltzmann path ensemble - and e.g. Ising model is a basic condensed matter model, which is assumed to use Boltzmann ensemble of e.g. sequences of spins - in spatial direction instead of temporal in QM.
Such spatial realization of Wick-rotated quantum mechanics seems to allow to violate Bell-like inequalities, so a natural next question is if we could build Wick-rotated quantum computers in Ising-like systems? For example to be "printed" on a surface, solving encoded problem if assuming Boltzmann ensemble among sequences?
Notice that Wick-rotated QC is different from adiabatic QC - the latter minimizes Hamiltonian, having huge problem with usually exponentially growing number of local minima. The former is closer to Shor - exploits path ensemble, should not have this optimization problem (?)
While quantum computers use unitary gates: with eigenspectrum in complex unitary circle, such Wick-rotated gates would have real eigenspectrum.
Hadamard gate $H$ is used to get initial superposition in quantum computers, below mixing gate $X$ can be used to get (Boltzmann) ensemble in Wick-rotated computers:
$$H=\frac{1}{\sqrt{2}} \left(\begin{array}{cc}1 & 1 \\ 1 & -1 \\ \end{array} \right) \qquad\qquad X= \left(\begin{array}{cc}1 & 1 \\ 1 & 1 \\ \end{array} \right) $$
In theory, controlled e.g. NOT, X should be also possible, the question is what could be realized e.g. in Ising-like system?
While in quantum computers we can only fix initial amplitude in the past, a big advantage of such spatial realization is that we could fix amplitudes in both directions (left and right), what might allow e.g. to solve 3-SAT (NP-complete, end of this arxiv).
In quantum subroutine of Shor's algorithm below, we prepare ensemble (past direction) of all inputs, calculate classical function and measure its value (future direction) - restricting the initial ensemble to inputs giving the same value of classical function - period of such restricted ensemble (found with QFT) gives a hint for the factorization problem.
Analogously for Boltzmann path ensemble for 3-SAT below, but in spatial realization we can also fix values from second direction (right) - restriction (in splits) becomes to inputs satisfying all the alternatives:
Which Wick-rotated gates could be realized in Ising-like systems?
Assuming we could build e.g. above 3-SAT setting, would it work? In other words - is Boltzmann sequence ensemble a perfect assumption, or only an approximation?
Is there a literature for Wick-rotated quantum computers, gates?