The picture below is a screenshot of Srednicki's QFT textbook.
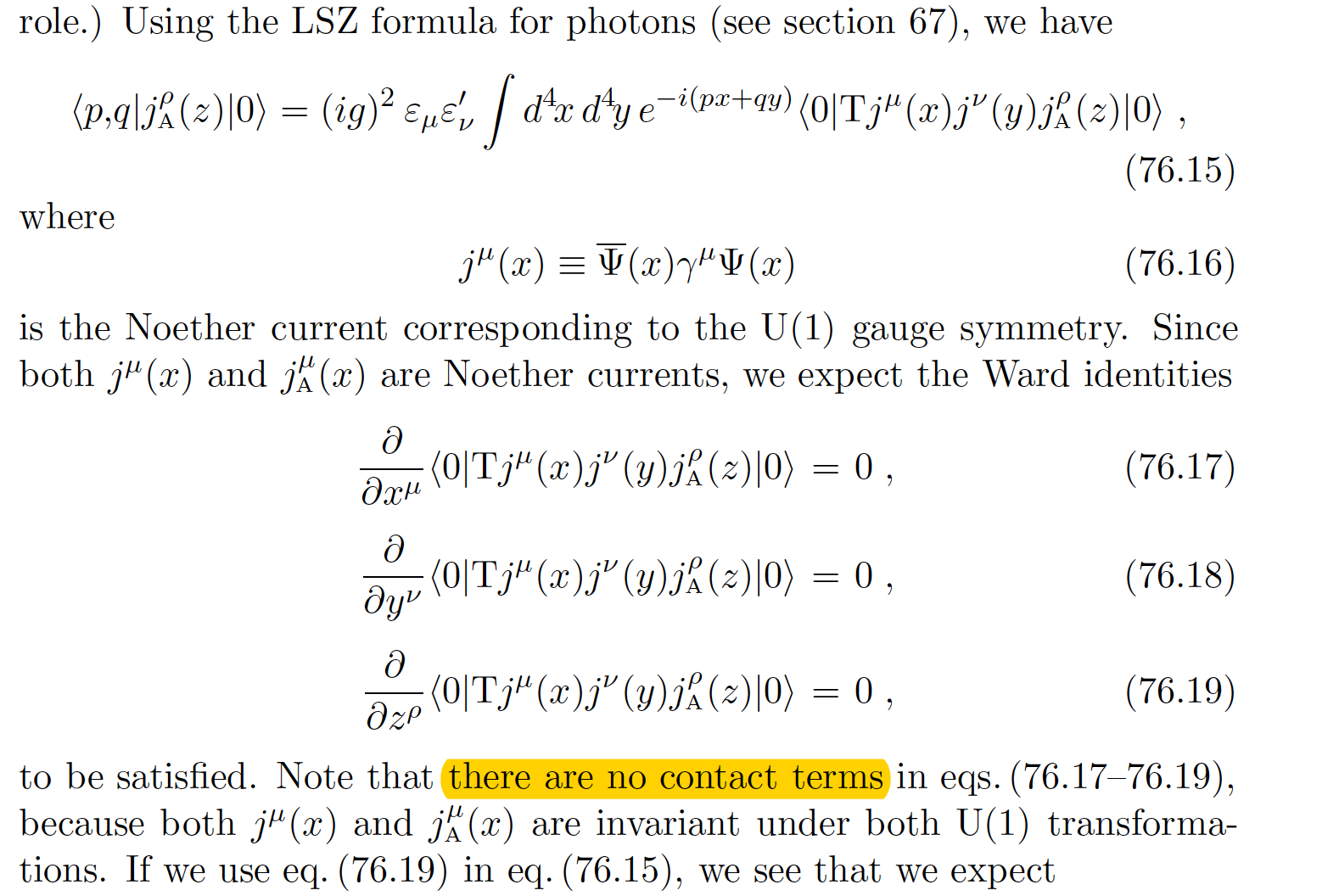
$j^{\mu}$ is the current associated with the $U(1)$ gauge symmetry; $j_{A}^{\mu}$ is the current associated with the axial $U(1)$ global symmetry (which is going to be proved anomalous).
I understand that both $j^{\mu}$ and $j_{A}^{\mu}$ are both invariant under both $U(1)$ gauge symmetry and axial $U(1)$ symmetry, but I don't understand why this will lead to the vanishing of contact terms in the Wad identities (i.e. the right-hand side of eqs.(76.17-76.19) are zero)? Hope someone can give me an answer. Thank you!