Most of the time the normal force doesn't do any work because it's perpendicular to the direction of motion but if it does do work, would it be conservative or non-conservative?
For example, consider athe following block on a friction-lessincline system where the incline which is itself on a friction-less surface. Now as the block moves down, the normal force that acts on the block by the incline doesn't do any workitself starts moving in the opposite direction. Here, but the normal force acting on the incline by the block does do work (because the incline itself starts moving).
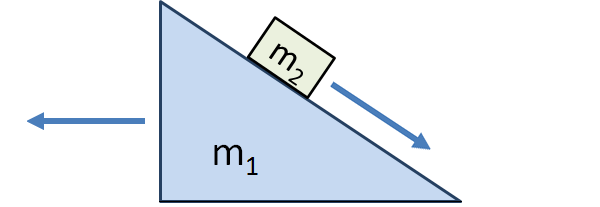
This question was on some website (it asked something about the final velocities of the block and the incline) and they solved this using mechanical energy and momentum conservation which confused me as I couldn't understand why the normal force here is conservative.
So, is the normal force always conservative or is this only in some cases? And if yes, how do we know for sure?
- Is the normal force always conservative or is this only in some cases?
- If yes, how do we deduce that like in the above example?
- Also, if it is conservative, what would be its corresponding potential energy function?
