At time $t = 0$, a one-dimensional free wave packet for a particle of mass $m$ takes the form:
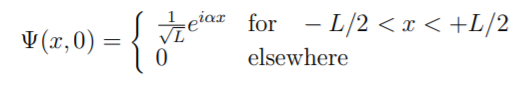$$ \Psi(x,0) = \begin{cases}
\frac{1}{\sqrt{L}}e^{i\alpha x} & \text{for } -L/2 < x < +L/2 \\
0 & \text{elsewhere}
\end{cases} $$
where $\alpha$ is a real constant. I need to find momentum amplitude $A(k)$ for this wave packet. And write the expression of the time-dependent wavefunction.
I know we can write a free wave packet as a linear combination of plane waves
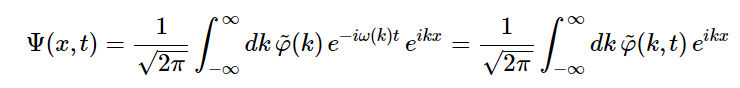$$ \Psi(x,t)
= \frac{1}{\sqrt{2\pi}}\int_{-\infty}^{+\infty}dk\,\tilde{\varphi}(k)e^{-i\omega(k)t}e^{ikx}
= \frac{1}{\sqrt{2\pi}}\int_{-\infty}^{+\infty}dk\,\tilde{\varphi}(k,t)e^{ikx} $$
And we chose the amplitude for the different plane waves as a Gaussian
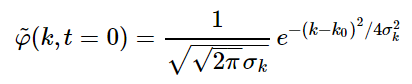$$ \tilde{\varphi}(k,t=0) = \frac{1}{\sqrt{\sqrt{2\pi}\sigma_k}}e^{-(k-k_0)^2/4\sigma_k^2} $$
and further, I can use the fact that
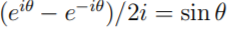$(e^{i\theta}-e^{-i\theta})/2i=\sin\theta$
But apart from these peicespieces, I have no idea how to proceed.
