In your figure 1 let's define a unit vector $\hat k$ to represent an anticlockwise rotation.

So the angle as drawn is $\theta \hat k$, the angular velocity is $\dot \theta \hat k$ and the angular acceleration is $\ddot \theta \hat k$.

The torque is $mgd \sin \theta \, (-\hat k) = - mgd \sin \theta \, \hat k $ and from that you get your equation $$I\ddot \theta \hat k =- mgd \sin \theta \, \hat k \Rightarrow \ddot \theta =- \dfrac{mgd \sin \theta}{I}$$

In your figure 2 let's define a unit vector $\hat K$ to represent a clockwise rotation.

So the angle as drawn is $\theta (-\hat K)=-\theta \hat K$, the angular velocity is $\dot \theta (-\hat K)=-\dot\theta \hat K$ and the angular acceleration is $\ddot \theta (-\hat K)= - \ddot \theta \hat K$.

The torque is $mgd \sin \theta \, \hat K$ and from that you get your equation $$-I\ddot \theta \hat K =mgd \sin \theta \, \hat K \Rightarrow \ddot \theta =- \dfrac{mgd \sin \theta}{I}$$

If your diagrams were showing the positions at maximum excursion at time equal to zero and the amplitude of the motion was $\Theta$ then for figure 1 the initial angular displacement is $\Theta \hat k$ and that for figure 2 is $\Theta (-\hat K)= -\Theta \hat K$ which is consistent with the fact that $\hat k = -\hat K$.

You would made life easier by usng the mirror image of figure 1 when drawing figure 2 which is the same as looking at the figure 1 pendulum from the other side ie looking at figure 1 from "inside" the computer screen.

I have rewritten my answer to address the concerns made in comments by @juancarlosvegaoliver.

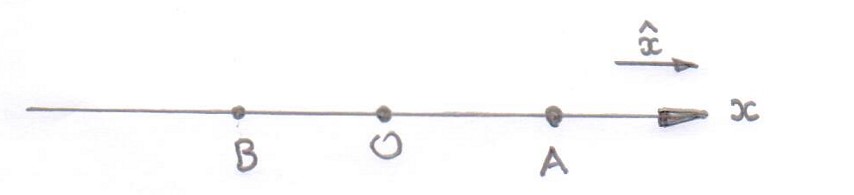
Instead of it being a rotation let it be simple harmonic motion in one dimension along the $x$-axis (vales increasing from left to right) and then I will show its relevance to the question asked.

The displacement from $O$ is $\vec x = x \hat x$ where $x$ is the component of the displacement in the direction of $\hat x$.
The velocity is $\dot x \hat x$ and the acceleration is $\ddot x \hat x$.
The force is $-k\vec x = -kx \hat x$ and $-kx$ is the component of the force in the direction of $\hat x$.

At a position like $A$ the displacement $\vec x$ in the direction of $\hat x$ and the direction of the (restoring) force is in the direction of $-\hat x$.
At position $B$ the displacement is in the direction of $-\hat x$ and the direction of the (restoring) force is in the direction of $+\hat x$.

So using $\vec F = m\vec a \Rightarrow -kx\hat x = m\ddot x \hat x \Rightarrow \ddot x = -\frac km x$ for all values of $x$ whether positive or negative.

Switching the direction of the unit vector to $\hat X = - \hat x$ makes no difference because now $\vec x = -x \hat X = -x \,(-\hat x) = x \hat x$ and $\vec a = -\ddot x \hat X = -\ddot x \,(-\hat x) = \ddot x \hat x$.
$\vec F = +kx\hat X = +kx (-\hat x) = -kx \hat x$.
For example, $2 \hat X = -2 \hat x$ and both give the position as $x=-2$

Having the $x$ axis pointing from right to left only results in a change of sign so, for example a position of $x=-2$ would now be $x=+2$.

Diagram 1 is the same as my diagram except that now $\vec x = x\hat x$ etc is replaced by $\theta \hat k$ etc and $\vec F - -k x \hat x$ is replaced by $\vec \tau = - mgd \sin \theta \,\hat k$.
The direction of $x$ increasing is to the right is replaced by the direction of $\theta$ increasing is anticlockwise.

Diagram 2 is just a reversal of the unit vector such that the new unit vector $\hat K = - \hat k$ with the direction of increasing $\theta$ still anticlockwise.
I have shown for the one dimension motion reversing the direction of the unit vector does not change anything and it is the same for the example with rotation.
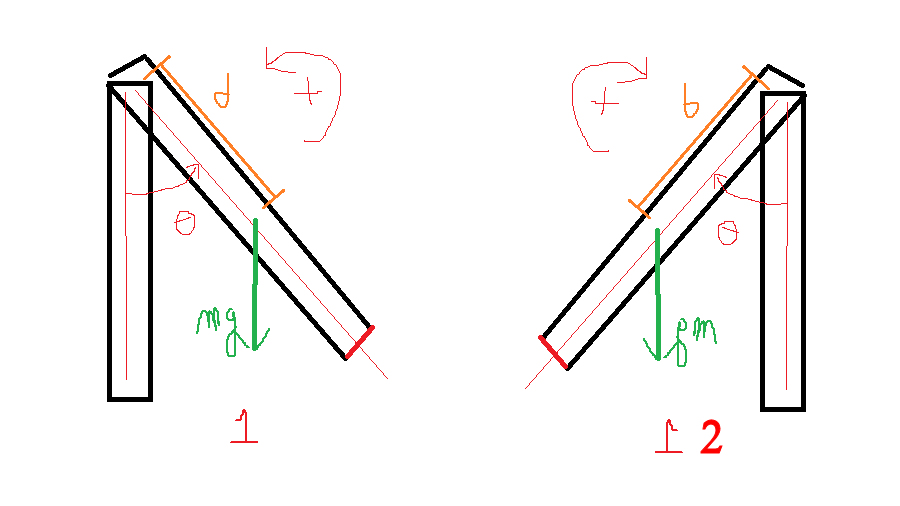
$\vec \theta = -\theta \hat K = -\theta \,(-\hat k) = \theta \hat k$ and $\vec \alpha = -\ddot \theta \hat K = -\ddot \theta \,(-\hat k) = \ddot \theta \hat k$.
$\vec \tau = +mgd\hat K = +mgd (-\hat k) = -mgd \hat k$.

In your figure 1 let's define an angle unit vector $\hat \theta_{\rm a}$ in the anticlockwise direction.

So the angle as drawn is $\theta \hat \theta_{\rm a}$, the angular velocity is $\dot \theta \hat \theta_{\rm a}$ and the angular acceleration is $\ddot \theta \hat \theta_{\rm a}$.

The torque is $mgd \sin \theta \, (-\hat \theta_{\rm a}) = - mgd \sin \theta \, \hat \theta_{\rm a} $ and from that you get your equation $$I\ddot \theta \hat \theta_{\rm a} =- mgd \sin \theta \, \hat \theta_{\rm a} \Rightarrow \ddot \theta =- \dfrac{mgd \sin \theta}{I}$$

In your figure 2 let's define an angle unit vector $\hat \theta_{\rm c}$ in the clockwise direction.

So the angle as drawn is $\theta (-\hat \theta_{\rm c})=-\theta \hat \theta_{\rm c}$, the angular velocity is $\dot \theta (-\hat \theta_{\rm c})=-\dot\theta \hat \theta_{\rm c}$ and the angular acceleration is $\ddot \theta (-\hat \theta_{\rm c})= - \ddot \theta \hat \theta_{\rm c}$.

The torque is $mgd \sin \theta \, \hat \theta_{\rm c}$ and from that you get your equation $$-I\ddot \theta \hat \theta_{\rm c} =mgd \sin \theta \, \hat \theta_{\rm c} \Rightarrow \ddot \theta =- \dfrac{mgd \sin \theta}{I}$$

If your diagrams were showing the positions at maximum excursion at time equal to zero and the amplitude of the motion was $\Theta$ then for figure 1 the initial angular displacement is $\Theta \hat \theta_{\rm a}$ and that for figure 2 is $\Theta (-\hat \theta_{\rm c})= -\Theta \hat \theta_{\rm c}$

You would made life easier by usng the mirror image of figure 1 when drawing figure 2 which is the same as looking at the figure 1 pendulum from the other side ie looking at figure 1 from "inside" the computer screen.

In your figure 1 let's define a unit vector $\hat k$ to represent an anticlockwise rotation.

So the angle as drawn is $\theta \hat k$, the angular velocity is $\dot \theta \hat k$ and the angular acceleration is $\ddot \theta \hat k$.

The torque is $mgd \sin \theta \, (-\hat k) = - mgd \sin \theta \, \hat k $ and from that you get your equation $$I\ddot \theta \hat k =- mgd \sin \theta \, \hat k \Rightarrow \ddot \theta =- \dfrac{mgd \sin \theta}{I}$$

In your figure 2 let's define a unit vector $\hat K$ to represent a clockwise rotation.

So the angle as drawn is $\theta (-\hat K)=-\theta \hat K$, the angular velocity is $\dot \theta (-\hat K)=-\dot\theta \hat K$ and the angular acceleration is $\ddot \theta (-\hat K)= - \ddot \theta \hat K$.

The torque is $mgd \sin \theta \, \hat K$ and from that you get your equation $$-I\ddot \theta \hat K =mgd \sin \theta \, \hat K \Rightarrow \ddot \theta =- \dfrac{mgd \sin \theta}{I}$$

If your diagrams were showing the positions at maximum excursion at time equal to zero and the amplitude of the motion was $\Theta$ then for figure 1 the initial angular displacement is $\Theta \hat k$ and that for figure 2 is $\Theta (-\hat K)= -\Theta \hat K$ which is consistent with the fact that $\hat k = -\hat K$.

You would made life easier by usng the mirror image of figure 1 when drawing figure 2 which is the same as looking at the figure 1 pendulum from the other side ie looking at figure 1 from "inside" the computer screen.