Suppose we haveWhen you fill a thin cylindrical glass of radius $r$ and height $h$. We fill it progressively with water or some other liquid, and we stop when no more liquid could be added without overflowing. For simplicity we assumewater forms a concave $r$ is much greater than the capillary length.meniscus with constant contact angle $\theta$ (typically $\theta=20^\circ$ for tap water):
What is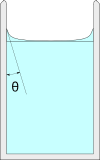
Once you reach the top of the glass, the water-air interface becomes convex and water rises up to a height $h'$ of liquid$\Delta h$ above the edge of the glass? Does it depend on, allowing you to fill the shape or widthglass beyond the naive capacity $\pi r^2 h$:
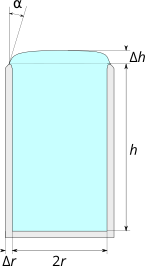
So when getting myself a glass of water, I came to wonder exactly how much this increases the edgecapacity of a glass, and what physical constants are involved.
My intuition would be that for a very large glass, $\Delta h$ converges to a constant so that the effective water capacity of the glass? grows like $\pi r^2 (h+\Delta h)$ (under the assumptionto make things simple I'm assuming that the edgeglass is small w.r.t.very thin: $r$$\Delta r\ll r$).
Feel free to add anything interesting about Perhaps such a constant depends on the precise shape of the liquid boundary (anglerim of contact with edgethe glass. But if not, typical curvatureperhaps it is a constant multiple of the capillary length?
So, differential equation characterizing itswhat can we say about $\Delta h$, the "rim contact angle" $\alpha$, or the shape...) of the water-air interface when the glass is filled at maximum capacity?
