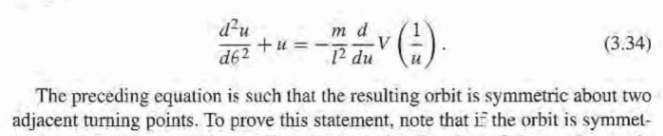In the book of Classical Mechanics by Goldstein, at page 88, it is given that:
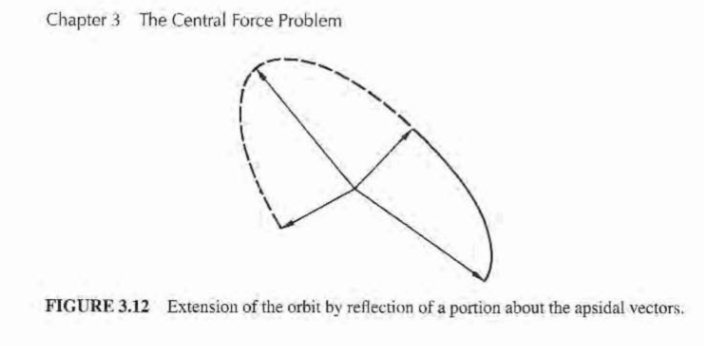
$$ \frac{d^{2} u}{d t^{2}}+u=-\frac{m}{l^{2}} \frac{d}{d u} V\left(\frac{1}{u}\right) . $$ The preceding equation is such that the resulting orbit is symmetric about two adjacent turning points. To prove this statement, note that i= the orbit is symmet-
However, the orbit might not be bounded, so there might not be two turning point; just one. In such a case, how can we argue that the orbit equation always has two turning points and it is symmetric about both axes?