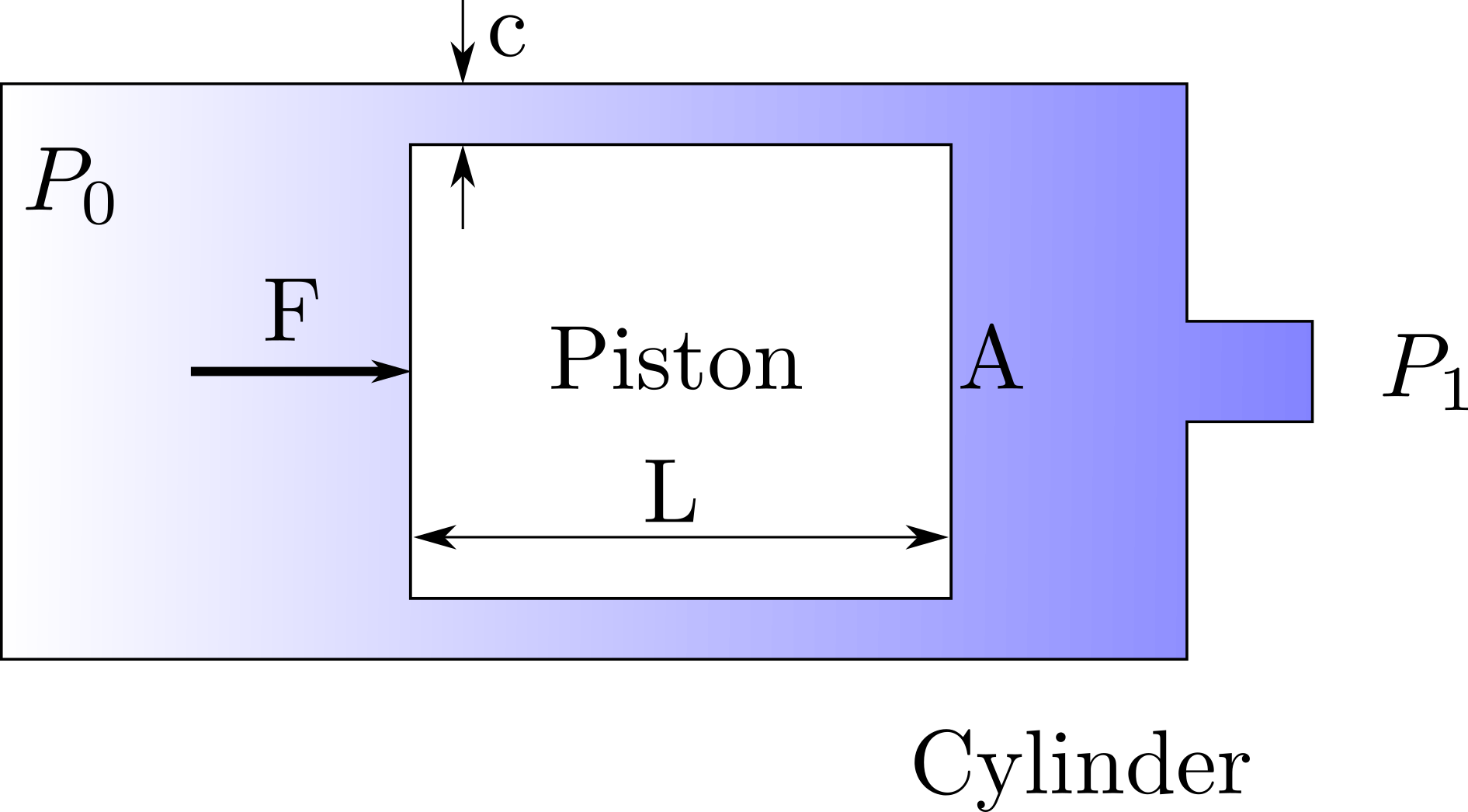
Assuming that we have a pneumatic cylinder-piston with arbitrary but known surface area A, a known clarence c, and a known length L. What is the best model to describe the total steady state-state force applied on the piston considering the leakage? (the force required to keep the piston in place)
What I'm looking for is an equation in the form of $F=f\left(P_1,A, L, c\right)$. In extreme cases:
- if $c\rightarrow 0$ then $F=P_1A$ is just hydrostatic pressure
- if c is big then $F\approx \frac{1}{2}\rho\nu^2C_DA$ is just air drag
I can calculate leakage from empirical or theoretical models but I don't know how to proceed from there.