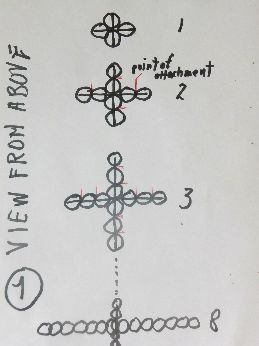
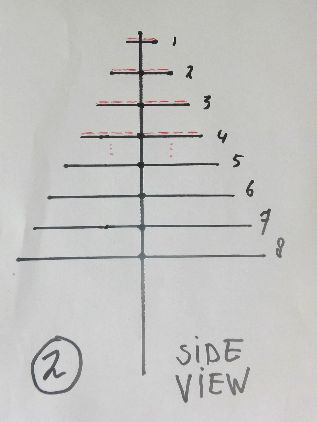
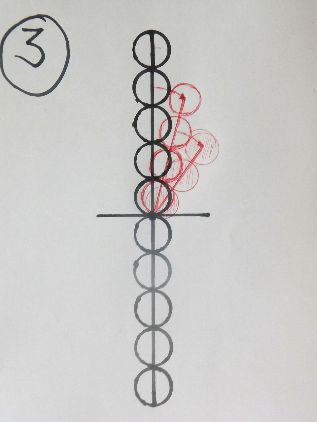enter preformatted text hereImagine Imagine, when no wind is blowing, rain is falling on an ideal tree: It has a one-dimensional vertical rigid trunk with one-dimensional single rigid branches perpendicular to the trunk (see picture 1, the side view) with spherical leaves such that in the upper part of the tree four of them can be put to the trunk just touching each other (see picture 2, the upper view). Without rain, the leaves are in horizontal position but they can rotate downward around the point of attachment to the branches when it rains. Each leave has a mass $m_{leave}$, and a "spring constant" $k_{leave}$, which tries to pull it back in horizontal position when rotating.
Suppose the tree is formed as in picture 1 and has eight layers of branches. Each layer consists of four perpendicular branches (see picture two, the upper view). From the top, each layer of branches is rotated such that the leaves from the layer above touch the leaves that are in the same position (see picture 3). The leaves have friction with the rain (otherwise, no energy could be transmitted to the leaves).
When it's not raining, seen from above, you can't see through the leaves and see part of the ground the tree is standing on.
When it starts to rain the leaves will rotate into the equilibrium state, with as a consequence the leaves will cover each other less.
I think it's a very difficult problem, but if the rain [carrying a moment density of $\rho(\frac{kg}{(sec)(m)^2})$] increases, how long does it stay dry under the tree, and if so, where?