I met a Hamiltonian containing the derivative of the Dirac delta potential:
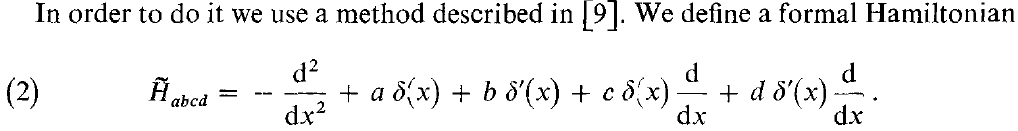
In order to do it we use a method described in [9]. We define a formal Hamiltonian $$ \tag{2}\tilde{H}_{abcd}=-\frac{{\rm d}^2}{{\rm d}x^2}+a\delta\left(x\right)+b\delta'\left(x\right)+c\delta\left(x\right)\frac{{\rm d}}{{\rm d}x}+d\delta'\left(x\right)\frac{{\rm d}}{{\rm d}x} $$
It is surprising to see terms like $b \delta'(x)$. How to, how should one interpret $ \delta'(x) $ $ \delta'(x)$?
