From the wikipedia article, for a Gaussian integral of an analytic function we have that
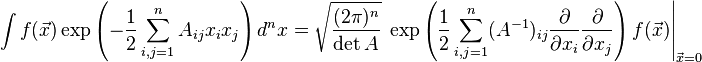
This is equivalent to the Wick theorem when f(x)$f(x)$ is a polynomial.
Now I'm trying to obtain a similar formula when there is a linear term in the Gaussian (ie the Gaussian has a nonzero mean).
My guess is that $$ \int f(x) \exp \left( - \frac{1}{2} x^T A x + B^T x \right) d^n x = \\ = \sqrt{\frac{(2 \pi)^n}{\det A}} \exp \left[ \frac{1}{2} \left( B^T + \frac{d}{dx_i} \right) A^{-1} \left( B + \frac{d}{dx_j} \right) \right] f(x) \bigg|_{x=0} $$$$ \int f(x) \exp \left( - \frac{1}{2} x^T A x + B^T x \right) d^n x = \\ = \sqrt{\frac{(2 \pi)^n}{\det A}} \exp \left[ \frac{1}{2} \left( B^T + \frac{\partial}{\partial x_i} \right) A^{-1} \left( B + \frac{\partial}{\partial x_j} \right) \right] f(x) \bigg|_{x=0} \tag{2}$$
but I can't prove it. Is this equation correct? How can I prove it?
