Why to use radius of gyration? [closed]

Question

I know the definition of radius of gyration. But don't know why does it mean? What are the importances of it? Is it similiar to centre of mass?

Answer 1

Radius of gyration is a geometric property of a rigid body like the center of mass. If you where to replace a planar body with a thin circular ring of radius $r$, you would choose $I=m r^2$ if you wanted the ring to have the same dynamic response as your rigid body.

Additionally, you can use it to find out the distance $\ell$ of the center of percussion from a pivot by $$\boxed{ \ell = c + \dfrac{r^2}{c}} $$ where $r$ is the radius of gyration and $c$ the distance of the center of mass from the pivot. I like the equation above much better that others found online (like http://www.real-world-physics-problems.com/center-of-percussion.html) because it eliminates the mass properties and simplifies the problem to a geometry problem. All $\ell$, $c$ and $r$ are geometric quantities.

There is another cool thing about figuring out the radius of gyration. You can use geometry to estimate the response of a rigid body to an impulse.

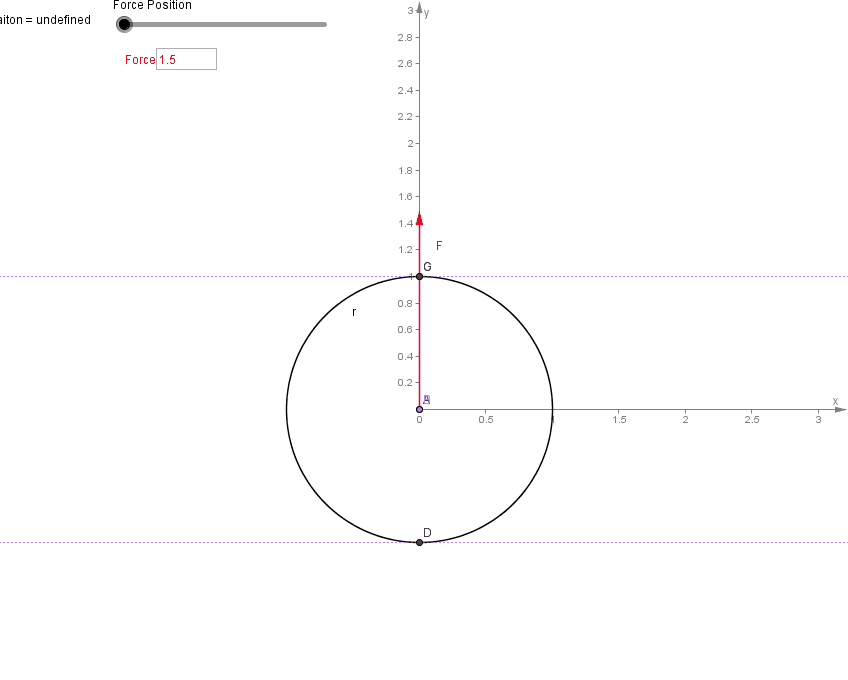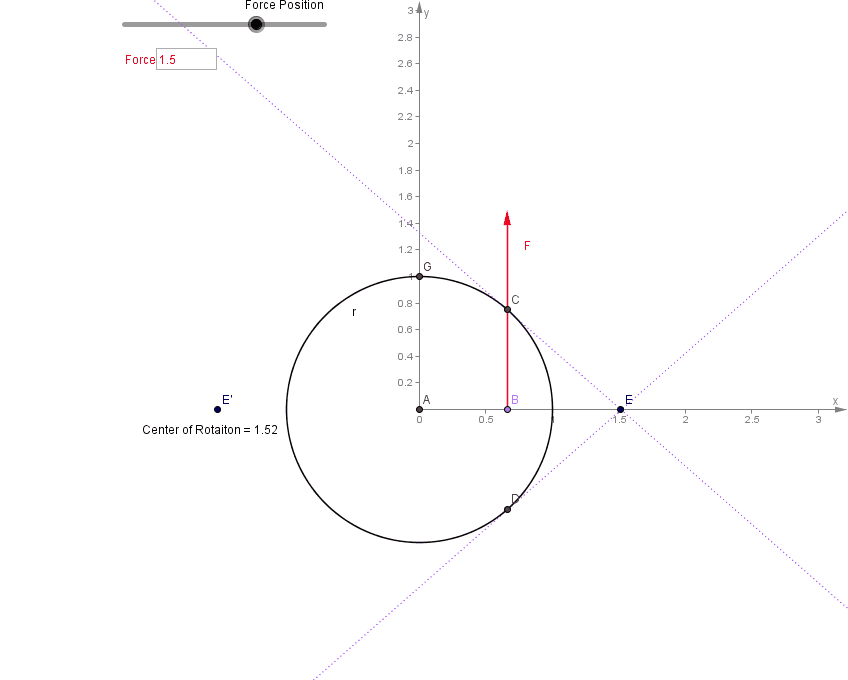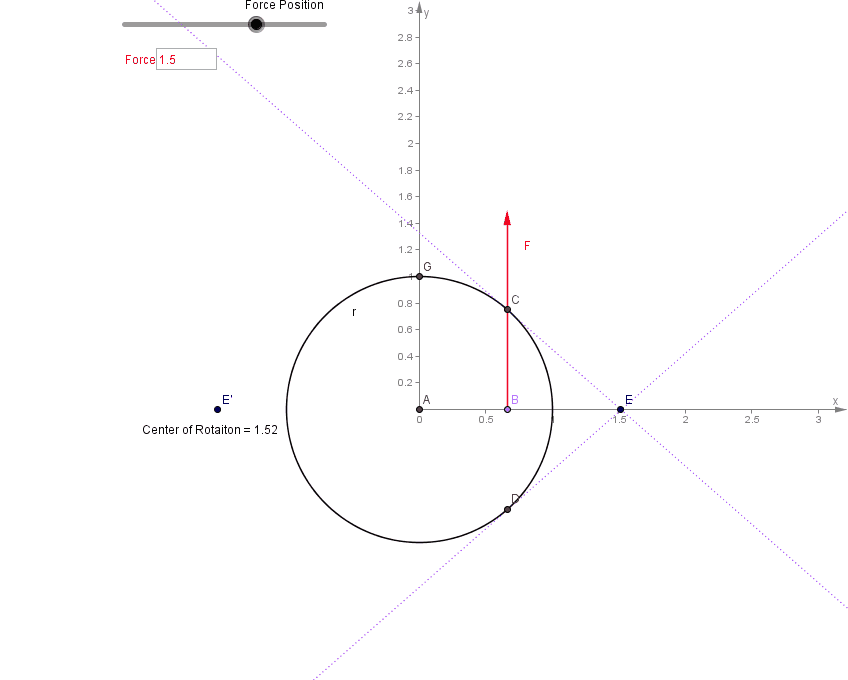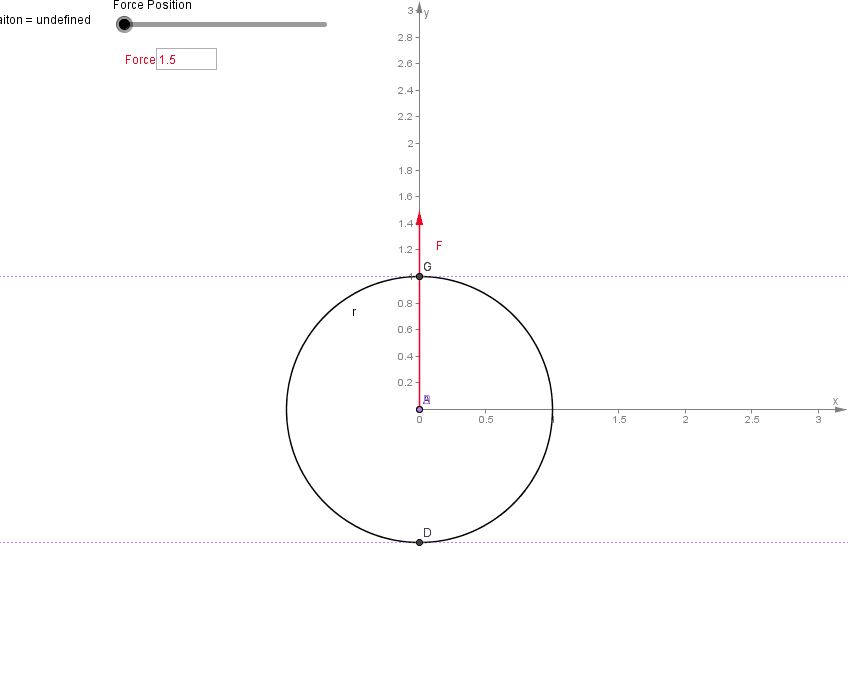
Draw a circle around the center of mass with radius $r$ and draw the line BC through which an impulse (or instantaneous force) is applied. Draw the tangents where the like of action intersects the circle and where they meet is point E. The mirror of this point (point E' below) is where the center of rotation is.

Obviously when the applied force goes through the center of mass, the center of rotation is at infinity (and thus the body translates only). The why the above is true is rather complex, but it involves something called projective geometry.