How do I deduce the Axis of Rotation of objects while solving rolling,angular momentum questions?

Question

A uniform rod of length $L$ lies on a smooth horizontal table. A particle moving on the table strikes the rod perpendicularly at an end and then stops. Find the distance travelled by the centre of the rod by the time it rotates by a right angle.

In the above the question,I use $I$ of the rod after the collision as $ML^2/12$ rather than $ML^2/3$ thus clarifying that the rod rotates about its $com$ rather than the end where the collision takes place.

Now in another situation,lets say the rod was standing erect on a table and the particle hits the rod at a distance of $L/4$ from the centre of the rod. Now in this case,i am sure the axis used will be at a distance from $com$ rather than $com $ itself. If i am wrong in this question than there are surely many cases in rolling where the objects rotate not at their com but a distance from it with respect to the mass of the particle which striked them and where.

In such cases,how do i decide the axis of rotation? Like in the first question itself,why wasnt the axis the end of rod but was its com?

Answer 1

Unless a pure torque is applied, a rigid body will never rotate about the center of mass only. But you are correct to consider the MMOI about the center of mass $I = \frac{m}{12} \ell^2$ as this will define the rotational motion.

• Motion of the center of mass $$ \boldsymbol{v}_C =\frac{1}{m} \boldsymbol{p} $$ where $\boldsymbol{p}$ is the aquired linear momentum after the impact
• Motion about the center of mass $$ \boldsymbol{\omega} = \mathrm{I}^{-1} \boldsymbol{L}_C$$ where $\boldsymbol{L}_C = \boldsymbol{r} \times \boldsymbol{p}$ is the angular momentum about the center of mass after the impact.

Axis of Rotation

If you know the motion of a point A on a rigid body ($\boldsymbol{v}_A$, $\boldsymbol{\omega}$) then the axis of rotation has the following properties

• Magnitude of rotation $$\omega = \| \boldsymbol{\omega} \|$$
• Direction of rotation $$\boldsymbol{z} = \frac{1}{\omega} \boldsymbol{\omega}$$
• Closest point on the axis of rotation $$ \boldsymbol{r}_{\rm rot} = \boldsymbol{r}_A + \frac{ \boldsymbol{\omega} \times \boldsymbol{v}_A}{ \omega^2 } $$

_{Where bold symbols are vectors, italic symbols are scalar, $\cdot$ is the dot product and $\times$ the vector cross product.}

The planar expansion is

$$ \boldsymbol{r}_{\rm rot} = \pmatrix{x_A \\ y_A} + \frac{1}{\omega} \pmatrix{-\dot{y}_A \\ \dot{x}_A } $$

_{$(x_A,\,y_A)$ is the position of A and $(\dot{x}_A,\,\dot{y}_A)$ the velocity of A, and $\omega$ the rotational speed of the body.}

Proof:

Expand $\boldsymbol{\omega} \times \boldsymbol{v}_A$ when the velocity of A is defined from the rotation as $\boldsymbol{v}_A = \boldsymbol{\omega} \times( \boldsymbol{r}_A - \boldsymbol{r}_{\rm rot}) = -\boldsymbol{\omega} \times \boldsymbol{r}$ where $\boldsymbol{r} = \boldsymbol{r}_{\rm rot} - \boldsymbol{r}_A$

$$ \require{cancel} \begin{aligned} \boldsymbol{\omega} \times \boldsymbol{v}_A & = \boldsymbol{\omega} \times ( -\boldsymbol{\omega} \times \boldsymbol{r}) \\ & = -\boldsymbol{\omega} (\cancelto{0}{ \boldsymbol{\omega} \cdot \boldsymbol{r}}) + \boldsymbol{r} ( \boldsymbol{\omega} \cdot \boldsymbol{\omega} ) = \boldsymbol{r}\, \omega^2 \end{aligned} $$

Axis of Momentum

Similarly given the momentum at a point A on a rigid body ($\boldsymbol{p}$, $\boldsymbol{L}_A$) the percussion axis is defined as

• Magnitude of momentum $$p = \| \boldsymbol{p} \|$$
• Direction of rotation $$\boldsymbol{e} = \frac{1}{p} \boldsymbol{p}$$
• Closest point on the axis of percussion $$ \boldsymbol{r}_{\rm per} = \boldsymbol{r}_A + \frac{ \boldsymbol{p} \times \boldsymbol{L}_A}{ p^2 } $$

Planar Case

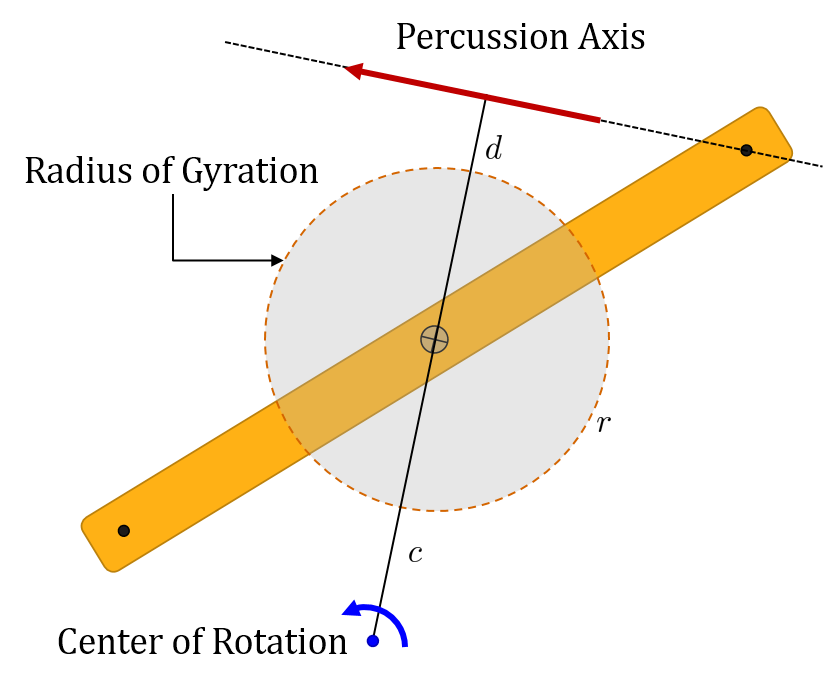
There is special relationship in the planar cases. Consider the diagram below. The percussion axis is a perpendicular distance $d$ from the center of mass. The object has radius of gyration $r$. The center of rotation is on the other side of the center of mass at a distance $c$.

$$ \boxed{ c = \frac{r^2}{d} } $$

Example

A thin vertical rod of length $\ell$ and mass $m$ is punched/kicked at a location $d = \frac{1}{4} \ell$ above the center of mass. Find the center of rotation, and determine if end of the rod is going separate from the ground.

The mass moment of inertia is $I = \frac{m}{12} \ell^2$ so the radius of gyration is $r = \sqrt{ \frac{I}{m} } = \frac{\ell}{2 \sqrt{3}} $

The pivot location is $c = \frac{r^2}{d} = \frac{ \frac{1}{12} \ell^2}{ \frac{1}{2} \ell} =\frac{1}{6} \ell $ from the center of mass. From the end this is $\frac{\ell}{2} - \frac{\ell}{6} = +\frac{\ell}{3}$.

Since the center of rotation is above the end of the rod, the rod is going to loose contact with the ground, and not just slide along the ground.

---

References:

• "Why does Torque exist?" : https://physics.stackexchange.com/a/242748/392
• "Calculate force between rotating objects" : https://physics.stackexchange.com/a/350684/392
• "Why to use radius of gyration?" : https://physics.stackexchange.com/a/159035/392
• "Physical meaning of the moment of inertia about an axis" : https://physics.stackexchange.com/a/280023/392

Answer 2

In classical mechanics, all external forces apply to the center of mass of rigid bodies independent of the point of application of the force. That induces a translation of the body. The rotations are induced by the sum of the torques r x F = I $\alpha$ from the point of application referenced to the center of mass. That is all what you need for the rod flat on the table.

As for your example of the standing rod, the gravity applies at the center of mass and induces no torque but friction may induce a "negative" torque in opposition to the movement due to the collision. In that case, with the competition between static and kinetic friction, you may reach a case where there is more translation or more rotation depending on the point of application of the external force along the rod (or the conservation of angular momentum induced by the collision
r x p = L).

Now, if you fix the center of rotation on the table with a standing rod, it is obviously different since everything will be a torque referenced to the point of rotation at the table. In that case, gravity applies at the center of mass of the rod and applies a torque since there is no translation. But I think you introduce friction in your discussion which is misleading when doing everything mentally. Sorry for the lack of equations in my answer but I can elaborate if you want to!