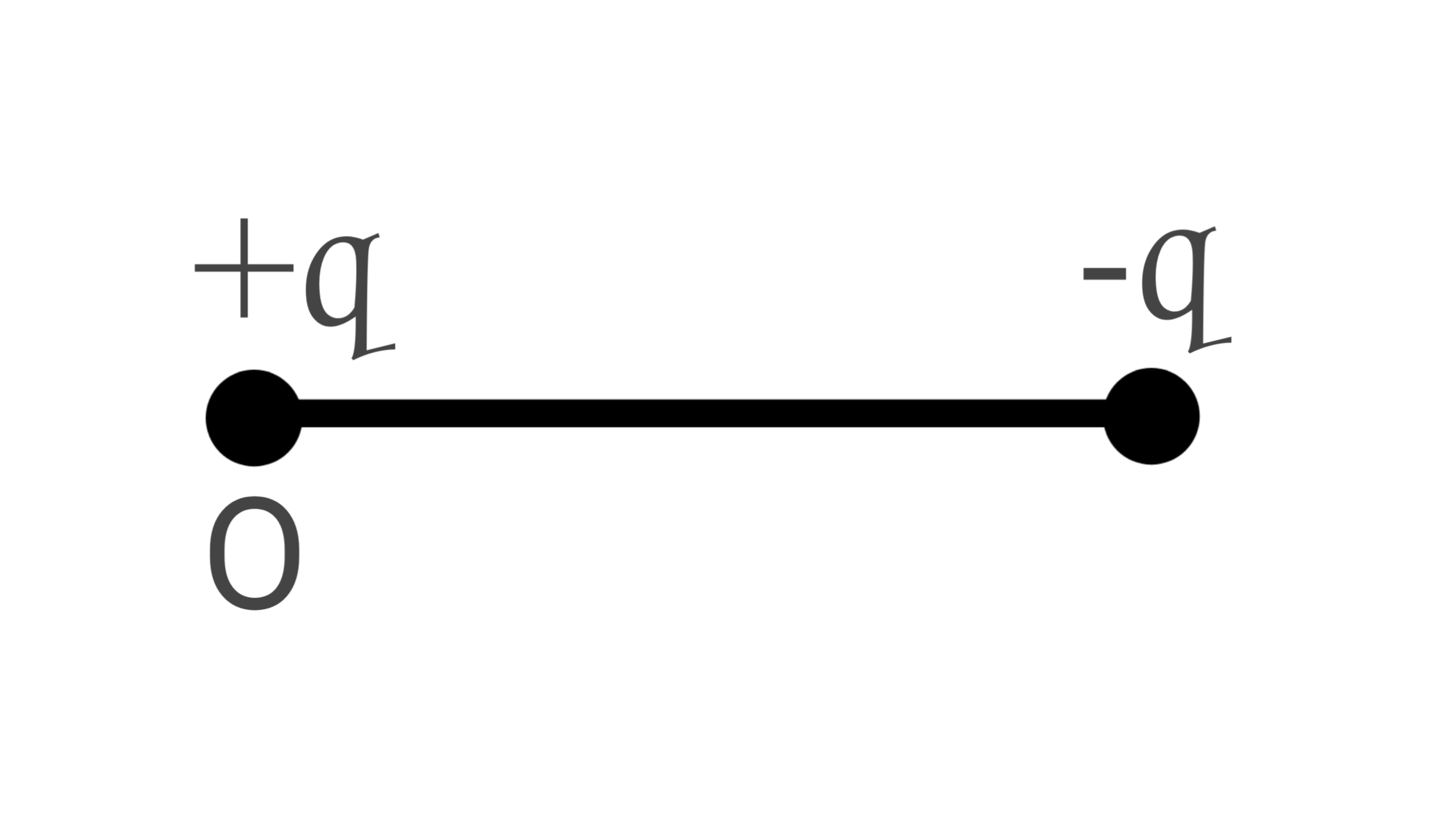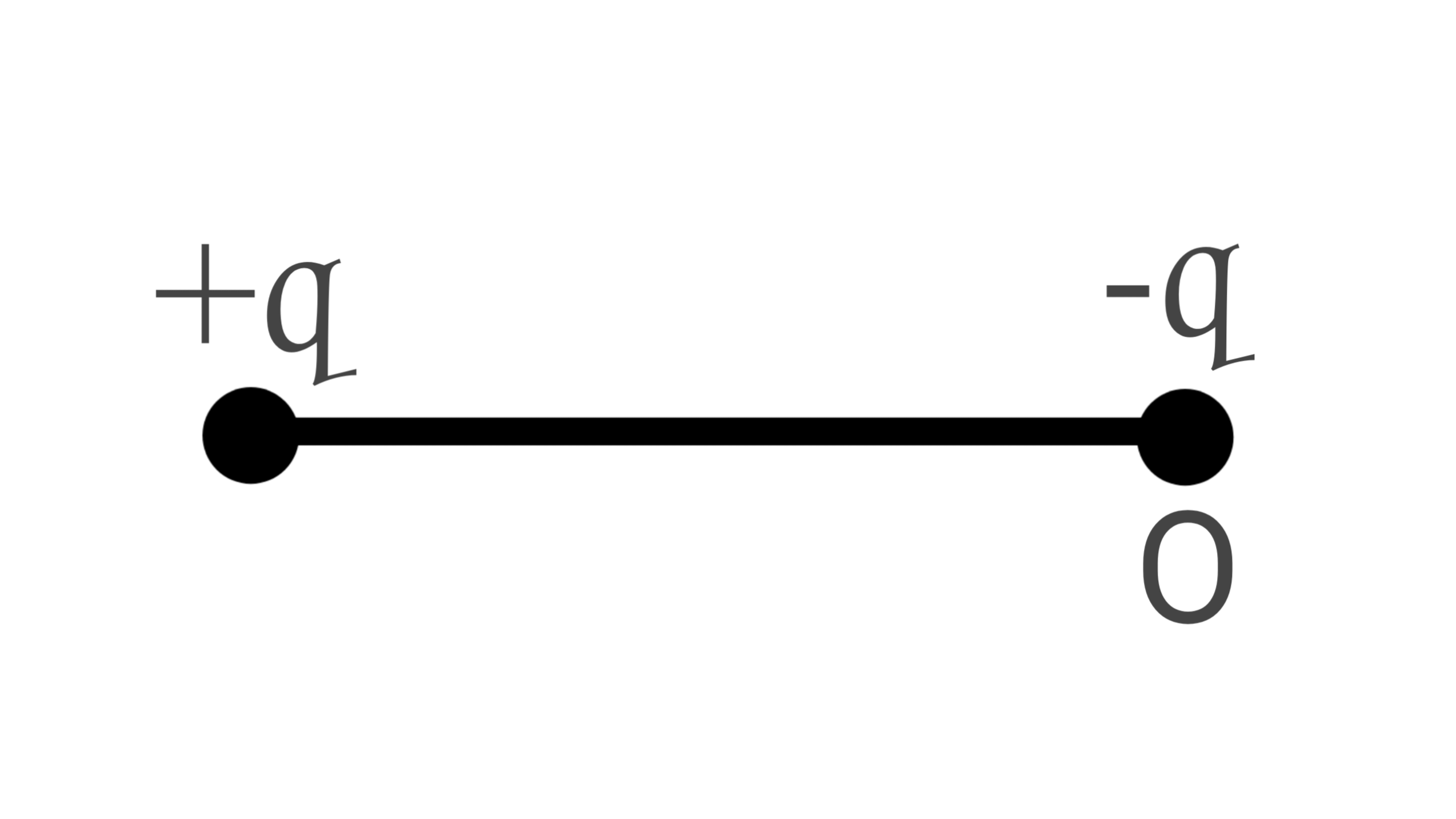An electric dipole moment is defined as $p = q\times d$ (for two point charges $\pm q$ separated by a distance $d$).
There are two separate issues here. (1) Why does it make sense to consider a dipole moment as a vector? (2) Given that it's a vector, why does it make sense to say that it points in this particular direction, rather than the opposite direction.
Intuitively, it makes sense to define a dipole as a vector because when we put it in a field, it aligns itself with the field like a little arrow. Fundamentally, we treat things as vectors when they transform as vectors. We have monopoles, dipoles, quadrupoles, ... Monopoles (electric charges) don't change under rotation, so they're scalars. Dipoles reverse themselves under 180 degree rotation, so they're vectors. Quadrupoles reverse themselves under 90 degree rotation, so they're tensors.
This is purely a matter of convention. According to the usual convention, the potential energy of an electric dipole is $-\mathbf{p}\cdot \mathbf{E}$. Historically, whoever first defined the dipole moment could have defined it with the opposite sign. Then the energy would have been $+\mathbf{p}\cdot \mathbf{E}$. The sign would also have been reversed in every other equation, e.g., $\boldsymbol{\tau}=\mathbf{p}\times \mathbf{E}$ would have become $\boldsymbol{\tau}=\mathbf{E}\times \mathbf{p}$.
There are many, many arbitrary choices of sign like this in physics. If Ben Franklin had made the opposite choice for the sign of the charge of cat fur rubbed on glass (or whatever it was he used as a standard), then we'd say today that electrons had positive charge. The direction of the magnetic field is also arbitrary and could have been defined as pointing the opposite way (in which case some of the signs in Maxwell's equations would flip).
I don't agree with Ben Crowell. I think that the reason that the moment is directed from negative to positive is because of the definition of moment:
$$ \mathbf{p} = \sum\nolimits q*\mathbf{d} $$ q: charge, d:distance from the origin of coordinates to the carge
If you have a negative and positive charge, this relation gives you the direction from negative to positive.
Here is why the electric dipole moment vector is always directed from negative to positive charge mathematically. (And you can have a dipole moment without having two opposite charges as well.)
When we study the multipole expansion of electric potential for any general electric charge distribution, the definition of electric dipole moment comes out to be
$$ \vec{p} = \int_V \vec{r} \, \rho(\vec{r}) \, dV $$ for continuous charge distributions as $\rho(\vec{r})$. Other symbols have the usual meaning.
Similarly for discrete charge distributions, the definition is $$ \vec{p} = \sum_i \vec{r}_i q_i \,. $$
From the above formula, it is evident that a pure monopole is a single charge at origin. If it's not at origin it can have a dipole moment too.
Next dipole moments are independent of origin if the net charge in the system is zero. Consider the system given below:
1.
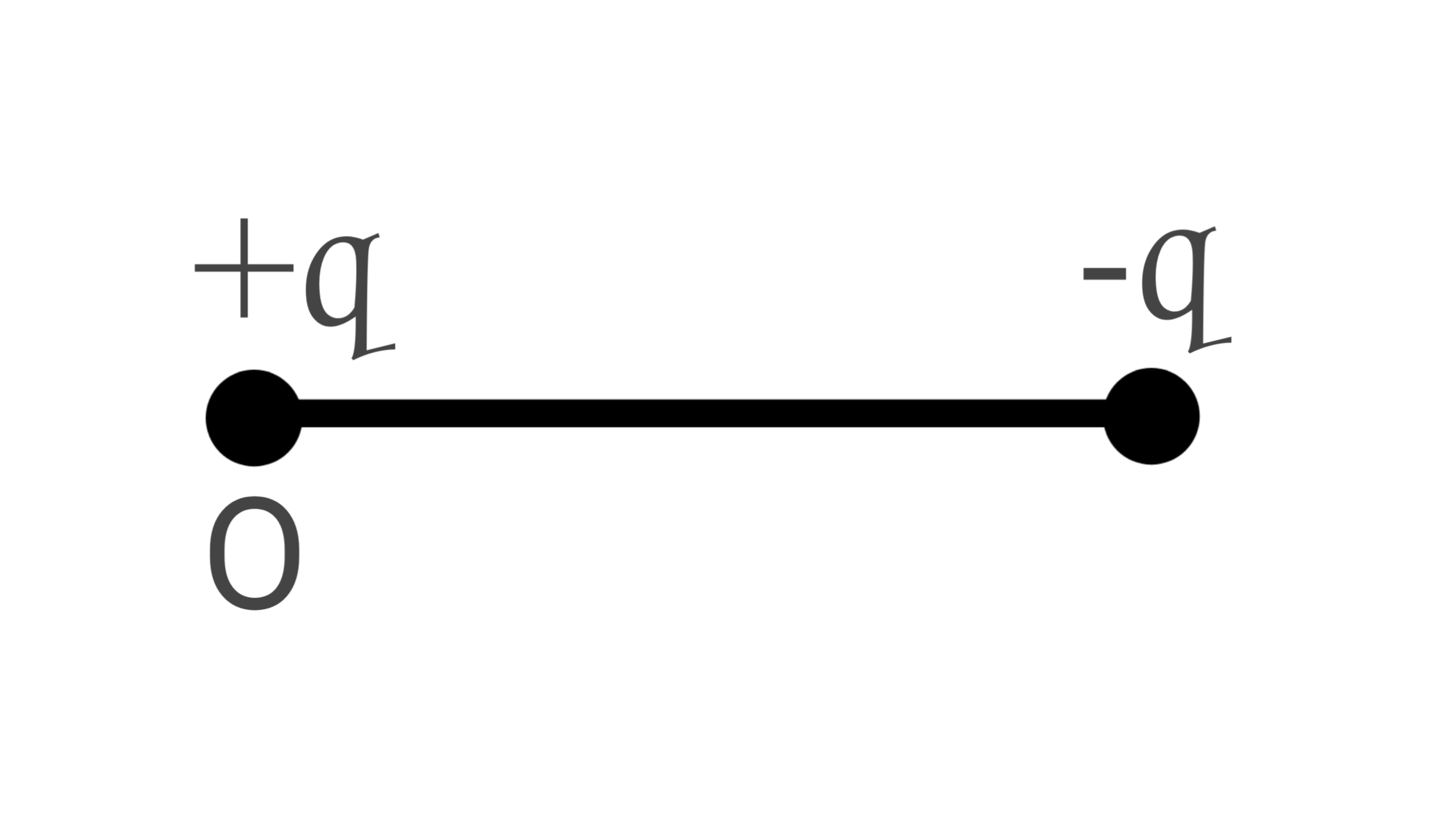
Here we have two charges $+q$ and $-q$ separated by a distance $d$. Net charge in the system is zero. Now if we choose them to be on $X$ axis with the positive charge at the origin. Then the dipole moment of the system is $ -q d \hat{i} $. Obviously this is a vector with direction from the negative to positive charge.
Now if we shift the coordinate system, so that the negative charge is now at origin, then
the dipole moment is $qd(-\hat{i})$. Again the same result with same direction.
One last case: Origin lies exactly in the middle:
Here also the dipole moment is $ -q\frac{d}{2}\hat{i} + q\frac{d}{2}(-\hat{i}) = -q d \hat{i}.$ So no matter where you choose your origin you will get the same vector as long as the net charge of the system is zero. If it's not zero then the dipole moment is origin dependent.
And that's why at the elementary level we were taught that the dipole moment is always directed from negative to positive charge.
There is no particular way of describing the direction of dipole rather it is hypothetic.But one should know the fact that if lenth is taken as 2L,means it has a specific origion(No matter for ease it is taken).Now as possible,draw a graph of dipole and its axial line co-insided with the X-axis.Therefore we will find the -ve charge takes the position on left of the origion shows charge is also negative.Now leave all the quadrants except when all trig.functions are +ve..i.e Quad.1st....Draw a point on axis on this quadrant,now predict the direction,we say it is from centre 'O' towards that point.i.e we are supposing its direction from origion to positive....i.e from left to right if direction is obtained from the pont left of origion to the point under consideration,we have to follow same rules.Same is in dipole direction i.e from -ve to +ve......Other all stories are hypothetical...
The dipole moment is in the direction in which unit test charge moves when placed on axial line of the dipole.
