How much weight can you put on a bike tire? What does it depend on?
$\begingroup$
$\endgroup$
4
-
$\begingroup$ theguardian.com/environment/ethicallivingblog/2009/aug/06/… $\endgroup$– Count IblisCommented Apr 10, 2015 at 19:55
-
4$\begingroup$ Depends on the bike tire. They aren't all the same. $\endgroup$– Jimmy360Commented Apr 10, 2015 at 20:03
-
1$\begingroup$ Once you put enough weight on it, they all will look pretty much the same, mind you... $\endgroup$– Jon CusterCommented Apr 10, 2015 at 20:18
-
$\begingroup$ Depends strongly on the pressure ... $\endgroup$– garypCommented Apr 10, 2015 at 20:53
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
The answer depends on the size of the tire, the pressure to which you inflate it, and how much deformation you are willing to tolerate.
Just to illustrate, I will take a road ("racing") bike as an example - using rough numbers so you see how the math is done. The tire might be just 20 mm wide, and inflated to 8 bar. Adding weight to the tire will barely increase the pressure inside, but it will increase the contact area - mostly the length of the contact patch. The tire will look a little bit "flat":
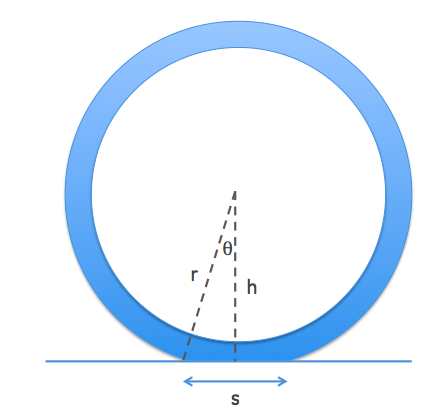
We can compute the area of the patch, and the degree of flattening, as a function of the contact angle $\theta$ in the above diagram:
$$\sin\theta = \frac{s}{2r}$$
The degree of flattening is given by
$$f = r - h = r(1-\cos\theta)$$
For example, a 100 kg person might load each wheels with 50 kg (500 N). At a pressure of 8 bar (8 kgf / cm2), you need a contact area of about 50/8~6 cm2 to support that much weight; this means the patch would be 3 cm long (it is 2 cm wide). For a wheel that is 70 cm diameter, that subtends an angle of about 2.5 degrees, and gives a "flattening" of less than a mm. This is why a road bike rolls so smoothly over a flat surface - the less distortion, the less friction.
Using the same equations to determine a "maximum" load, let's assume we can tolerate a distortion of 5 mm; we can now work backwards:
$$f = 5 mm\\ \cos\theta = 1 - \frac{5}{350}\\ \theta \approx 10°\\ s = 2r\sin\theta \approx 120 mm$$
With the contact patch 12 cm long, it has an area of 2*12=24 cm2 and can support a force of 24*8~200 kg
But it would get pretty hard to pedal. Note that when you go over a sudden bump, you can quite easily hit these kinds of distortions - and if you land a jump, you will also significantly increase the (transient) force supported by the tire. Note also (per my linked answer (2) below) that the pressure in the tire will hardly change because of these distortions - the fractional change in volume of the tire is very small. Overloading the tire will lead to
- lots of friction (hard to pedal)
- excessive wear (the distorted patch "rubs" on the ground)
- possible puncture ("snakebite" when your tire gets pinched between the ground and the rims)
- broken spokes (especially if the wheel is warped and spoke tension is uneven)
