Normal modes
Let us first take a classical view of coupled oscillators - e.g., balls connected by springs or atom in a lattice (which, for small displacements, can be reduced to the balls coupled by springs.) Classical mechanics teaches us that we can find the normal oscillation modes of such a system, and represent any oscillation as a superposition of such normal modes. Any single mode then obeys a harmonic oscillator equation:
$$
\ddot{Q}_k(t)+\omega_k^2Q_k(t)=0,\\
H_k=\frac{P_k^2}{2}+\frac{\omega_k^2Q_k^2}{2}.
$$
See here for an example of a dispersion relation that we can get:
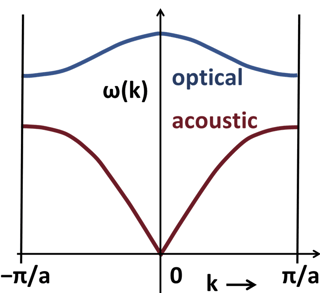
Quantization
If we consider the same problem from the point of view of quantum mechanics, we expect that the excitations will be quantized. The quantization of Harmonic oscillator is a well-known problem - each mode will have discrete energies:
$$
E_k=\hbar\omega_k\left(n_k+\frac{1}{2}\right),
$$
where $n_k$ characterizes the level of excitation of modes. Note that, given the finite crystal size (i.e., the finite number of atoms - say $N$ atoms) we have finite number of modes, $k_1,...,k_N$, but the excitation numbers can be arbitrary.
Second quantization
We now can perform second quantization or, in a simpler and oscillator-specific language, describe the modes in terms of raising and lowering operators:
$$
H_k=\hbar\omega_k\left(a_k^\dagger a_k+\frac{1}{2}.
$$
Note that the number of the oscillators is still $N$, but the operators now work in the Fock space (filling numbers space), so the level of excitations can be arbitrary.
Phonons
The last step is calling these new excitations - phonons. In this case, $a_k^\dagger, a_k$ are no more raising and lowering operators, but phonon creation and annihilation operators, whereas $n_k$ becomes a number of phonons excited in mode $k$. In many ways phonons behave just any other particles, although for the sake of caution one calls them quasiparticles. There exist many other similar excitations, which are obtained from oscillatory modes - plasmons (for electron plasma in metals), magnons (for spin waves), polaritons (in Bragg lattices or cavities), etc.
Related
Is differentiating particle and quasiparticle meaningless?
Do quasiphotons have mass?
Reading
Charles Kittel, Introduction to solid state physics - standard text
H. Haken, Quantum field theory of solids - an accessible introduction to solid state physics from quantum quasiparticles viewpoint
